Why won't my perpetual motion
machine work?

by Donald E. Simanek.

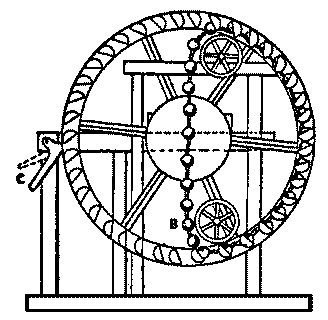
The machine in this illustration is from an idea of 18th century Scottish astronomer James Ferguson, intended to show why perpetual motion wheels can't work.

I don't maintain a web message board. But many people contact me by email. Here's a compilation of questions people have raised over the last 15 years or more, and some reasonably short responses.

Is an "over-unity" machine the same as a perpetual motion machine?

The fundamental laws of physics do not prohibit perpetual motion. But properties of physical objects conspire to prevent it. Friction and other dissipative processes convert orderly motion to disorderly motion, thwarting our efforts to achieve perfect (100%) energy efficiency in machines.

Inventors have little interest in merely producing perpetual motion. They want over-unity performance—unlimited energy output for free.

"Over-unity" means an energy efficiency greater than one. Suppose you could make a device with efficiency of 200%. It puts out twice as much energy as it takes in. Then you could take half the output energy and use it as input energy for the device, and keep it running forever. This would be a perpetual motion machine and would put out useful energy as well.

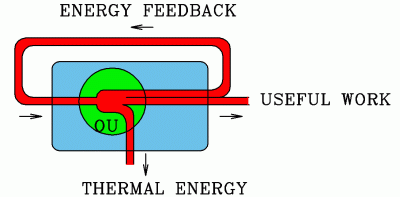	Energy flow chart of a hypothetical over-unity machine. The secret over-unity device (OU) increases the energy by some unknown physical principle. Some of the energy is fed back to the input, some wasted as thermal energy, and some output as useful work.

This is why "over-unity" and "perpetual motion" are often used as if they were synonyms. If you can achieve over-unity, you have also achieved perpetual motion, but not vice versa.

Wouldn't this be dangerous?

Feedback.

Suppose you have built such a 200% efficiency machine. Feed back half its output to its input and it then needs no other input power source. Take its remaining output and feed it into another such device, and then you get double the initial output. Cascade a bunch of these in series and you get unlimited output power for free, except for the cost of making the machines. For a modest investment in machinery you could power the earth. Or destroy it.

But, even simpler, just feed back all of that output to the input. The machine is now out of control, a case of positive feedback leading to instability. The power output could only be kept from going to infinite values by the machine's internal power-handling limitations, or by power-limiting safety systems that we build into it. Even if the over-unity is modest, say an efficiency of 110%, you could still get unlimited power output, it would just take a bit longer to build up. Don't try this at home!

A perpetual motion machine would be safer, for it doesn't increase energy.

Pierre Richard (engineer, Paris), 1858, British patent No. 1870. Notice the friction brake on the left side. Dircks (1861), p. 482.

You may have noticed that no one has yet achieved this, or has even come close. I'm not worried about this doomsday scenario happening. I have noticed that several times in recent years some people have claimed they have achieved something like 135% efficiency in their machines. I strongly suspect that they don't know how to measure efficiency properly.

In the older literature one sometimes sees perpetual motion machine designs in which the inventor has included a friction brake, or a speed governor, presumably to guard against such catastrophes. Or maybe they were hoping that the load at the output would keep the machine from destroying itself. But in recent claims of presumably more sophisticated devices, the inventors seem to have completely ignored the possibility of instability due to over-unity performance. And yet we haven't heard of any of their devices suffering meltdown. Curious, isn't it? But then, we haven't heard of any confirmed reports of their wonderful devices producing useful work continuously either. Hmm...

The reader may recognize the similarity of this to the ancient mathematical fable of the Wheat and chessboard. One grain of wheat is placed on the first square of the board, 2 on the second, 4 on the third; each time doubling the previous amount. When all 64 squares are filled with wheat, there are 18,446,744,073,709,551,615 grains on the board. After 64 passes through our 200% efficiency over-unity device with energy feedback, the energy has been multiplied by that much.

If this could be controlled, wouldn't it be completely non-polluting?

This exposes a common misunderstanding. In the world of real machinery poor efficiency is thought to be only due to friction. Just reduce the friction to zero, and all we'd have is a perpetual motion machine with efficiency equal to one. But reducing friction and other dissipative processes to zero won't get you to efficiencies greater than one, that is, over-unity performance. To do that you'd have to find some way to "multiply" energy, or create energy. If that were possible some of the newly created energy could be useful output, but some would still be converted to thermal energy due to friction.

What does "closing the loop" mean?

Example: A motor is used to drive a generator, and the electrical output of the generator is fed back (closing the loop) to power the motor. Sounds absurd, doesn't it? It is. Even if the motor and generater were both 100% efficient, it would not work.

How can I calculate or measure the efficiency of my device?

My device has an energy efficiency of 95% when it has input power driving it, but without power input it comes to a stop. If I increase the efficiency just a bit more, say just 10% more, won't it have efficiency of 105%, that is, over-unity performance? It could then run itself without input power by closing the loop. That shouldn't be difficult.

Light objects rise in liquids, the buoyant force overcoming gravity. Can we tap that buoyant force continually to do useful work?

Some inventors try to tap energy of the buoyant balls by letting them fall from the top of the liquid tank down some sort of ramp to the bottom, extracting energy from them as they fall down. Then the balls are inserted back into the water through some clever mechanical valve at the bottom of the tank. Unfortunately, the work required to push a ball through that valve, working against the pressure difference between liquid and air, is just equal to the energy it would gain as it rises to the top of the liquid. There's no energy gain in the process, only energy lost to viscous drag.

Simple mechanisms can multiply force. They can also multiply the distance through which a force acts. Can't we simply combine or reconfigure these to multiply both force and distance simultaneously, and therefore multiply work?

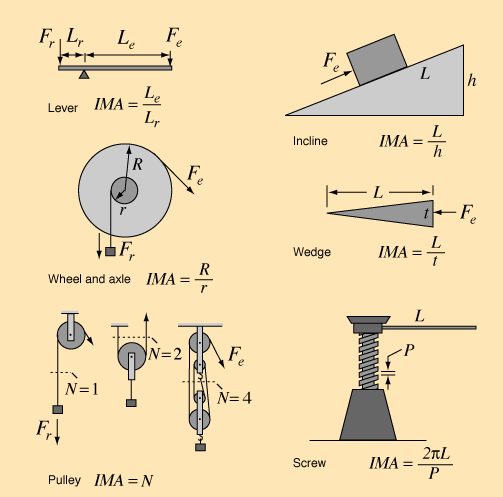
	The simple machines known to the Greeks in the 3rd century BCE. This is a typical presentation from a modern elementary physics textbook. IMA is the "ideal mechanical advantage", i.e., the mechanical advantage (ratio of output to input force) in the perfectly frictionless case.

The early engineers did more than discover these machines. They also analyzed them to find out how they worked. In the process they learned that the product of force and distance can never be increased in any of these mechanisms or any combination of them. Work is the product of force and distance. Work out = Work in - Energy losses due to dissipative processes. Some perpetual motion machine inventors haven't yet caught up on this fact of nature.

My device will require magnets and magnetic shields. Where can I buy suitable magnetic shields?

Advertisement for a magnetic corset.

Magnetic shields are useful for keeping the magnetic component of electromagnetic radiation away from sensitive electronic circuits. They are not as effective for steady or slowly varying magnetic fields. Magnetic shields work by redirecting magnetic field lines so that they are mostly kept away from regions where we don't want them. They do this by re-routing magnetic field lines through the shielding material instead of somewhere else. Therefore the magnetic material still experiences forces from the magnets, and Newton's third law still applies. A magnetic shield and a permanent magnet are strongly attracted to each other. So in analyzing a device with magnetic shields you must include all forces acting on the shields and the forces shields exert on other parts of the device. Inventors usually totally neglect even considering the forces on the shields and the work done on and by these forces. For rapidly varying AC fields, the average force exerted on the shield can be nearly zero, but considerable mechanical energy is still lost by heating the shield. While the electric field component of AC radiation can be almost entirely shielded from a finite volume by a full metal enclosure around that volume (acting as a Faraday cage), the magnetic component can never be completely shielded.

Nickel-Neodymium magnet on top of a sheet of mu-metal magnetic shielding easily supports unmagnetized ferrous objects on the bottom side.

Commercial shielding materials are ferromagnetic alloys. They cannot create or eliminate magnetic fields, only redirect them. You can't "block" the attraction of two magnets by placing such a shield between them. The magnets will then be attracted to the shield, experiencing forces in nearly the same direction as before.

If you place a strong magnet near one side of a magnetic shield (such as mu-metal), the region on the other side of the shield will not be field-free. In fact, a ferrous metal object on the "shielded" side will still be attracted toward the shield. Experimenter's note: Manufacturers of shield materials advise against placing strong magnets in contact with a magnetic shield, for this can induce a residual permanent magnetism in the shield. I place a 2 mm thick nylon washer between the magnet and shield.

When an inventor uses magnetic shields in an over-unity device proposal, he's imagining a kind of shield that does not exist. He assumes a "magic shield" that violates fundamental laws of physics. But most proposals I've seen still wouldn't work even if the magic shields did perform as the inventor hoped.

At least one company that sells magnetic shields warns buyers that these shields are primarily useful for radio frequency shielding. The company must have had quite a few inquiries from perpetual motion machine inventors. I can only imagine letters asking "Which of your many magnetic shielding materials is best for use in a perpetual motion machine?"

Antigravity shield woven into a carpet.

The inexperienced inventor imagines that magnetic shields act on static magnetic field lines in the same way that an opaque object "blocks" (absorbs) light. They don't. Only for high frequency electromagnetic radiation does anything of that sort happen.

Before anyone asks, there's no such thing as a gravity shield. And magnetic adhesive patches do not relieve the pains of rheumatism, can not increase your gasoline mileage if you put them on the fuel line, nor ionize or detoxify water if placed on water pipes. Nor are all of those refrigerator magnets making the food inside the refrigerator any healthier to eat.

My device uses an innovative method to maintain continual overbalance of mass, force, and torque. But it still stubbornly sits there, unmoving, taunting me.

In any wheel-type device, each mass must complete a closed path. The work gained over part of the path as a mass falls is equal to the work required to raise it up again. To try to get around this fact of nature is as futile as finding a round trip walking path that is downhill all the way, in either direction.

The closest you can come to perpetual motion is a simple flywheel with frictionless bearings. It would turn on its own for a very long time, but produce no output of useful work. Any "improvements" you make on this, no matter how ingenious, using gears, shifting weights, magnets, fluids, quantum mechanics, etc., only reduces its performance.

Magnetic perpetual machines can never work because magnets eventually wear out.

You can't create something from nothing.

Suppose you have a strong box. Put one apple in it and close the lid. Open the lid and find two apples inside. Assuming no trickery you know that can't happen. So why would you think any machine can take in a certain amouunt of energy, and output twice as much energy. Energy is a thing, and you can't create something from nothing.

Can I improve the performance of my over-unity wheel or belt device by making it larger?

Making the chain longer and adding more weights won't help.

Larger is not always better. Making such a mechanism larger may seem a good way to increase the "overbalance" that you suppose will make it work, but increasing the size also increases the net load that needs to be moved and/or the distance it must be moved, and these factors are in direct proportion. So save money and make a small model. It will fail for much lower cost than the larger one. Some inventors have even built wheels as large as a carnival Ferris wheel. They only turn when the wind is blowing.

Some have modified an overbalanced wheel device into a belt device, then supposed that the overbalance could be made greater by making the belt longer. It can, but that also adds more mass that must lifted a greater distance up the other side of the belt. Nature has gotcha again.

My wheel with moving masses doesn't work. Would it work if I add more moving masses or make them heavier?

My wheel has many identical moving parts to achieve continual overbalance. I can't afford the expense of building a model.

Second, consider testing just one of those moving parts in a simpler design, perhaps with a pendulum arrangement. It still won't achieve perpetual motion, but you might learn some physics hands-on.

I did a computer simulation of my idea and it works beautifully. But when I build it, the darn thing just stubbornly sits there at rest.

I'm not refering to animated pictures here. They can, of course, depict both possible an impossible situations. The internet has many examples of such animations of impossible devices and situations, done for amuseuemt. I'm talking about the professional and expensive software programs that engineers use to predict behavior of systems in the real world. These use standard laws of physics and properties of materials, and are incable (if properly configured and supplied with valid and complete data) of predicting anything that would violate thoe laws.

Similar caveats apply to folks who say "my idea works fine on paper." Yes, and visual illusions of impossible objects also look fine on paper.

Too complicated to understand.

My design has gone through many changes and improvements. It's now so complicated that I don't understand how it works. But I'm certain that it will work. Can you help me?

My magnet wheel won't turn. Should I buy stronger magnets?

My wheel-type device doesn't turn by itself even once around. Will reducing friction help?

Reducing friction won't fix an unworkable device. © Raymond James.

Friction is never the sole reason for the failure of a supposed over-unity device. Even if you could remove friction entirely it wouldn't work. Look for the real reason for its failure. You can be sure the reason isn't friction.

Likewise, viscosity is never the sole reason for failure of perpetual motion and over-unity devices using liquids. Assume an over-unity machine that is free of friction, viscosity, and all other dissipative processes. Analysis will always show that it still can't work even if completely idealized.

Most machines depend on friction to work. Imagine a world without friction of any kind. You couldn't walk, vehicles could not move, belts would slide over pulleys, knots would come undone, structures would collapse.

Removing all friction may not even be a good idea. Cartoon © 1987 by John Holden.

Looking at books and websites, I conclude that all the simple perpetual motion ideas have been tried, and all have failed. Can some of these ideas be modified, improved or combined to be successful?

That has been tried, too. Any clever improvement or ingenious mechanical gimmick increases a device's mechanical complexity and degrades performance. The closest you'll ever come to a perpetually turning wheel is a simple flywheel with frictionless bearings. Any "improvements" you add will bring it to a stop sooner.

I want to tap energy from natural sources. Which would be the best source, gravity or magnetism?

But someone objects. "When I ski down a snowy mountain slope I gain kinetic energy. Doesn't that come from gravity?

When we are talking about perpetual motion we are dealing with cyclic machines, devices that complete a closed cycle of operation, indefinitely. When you go to the ski resort the potential energy you have at the top of the ski run came from the work you did walking up the mountain, driving up in an automobile, or taking a ski lift. At the top you have potential energy relative to the bottom. That potential energy is what gives you kinetic energy as you ski down the slope. Gravity was not the source of that energy; it was an intermediary agent.

But couldn't gravitational fields provide an unlimited source of energy? All our machinery operates in a gravity field, and some depend on it.

Someone may bring up waterwheels. Isn't that a cyclic motion dependent on gravity? Waterwheels are cyclic, but they are only part of a larger process which isn't a closed cyclic process and doesn't extract energy from gravity. The energy comes from water flowing from higher to lower elevation. The water then flows down streams to lakes or oceans, where radiant energy from the sun evaporates some of it and atmospheric circulation (also sun-driven) moves it elsewhere and dumps it as rain. Some of that rain falls at higher land elevations, forming streams which power waterwheels, and so on. This is a cyclic process, but not a closed one. It requires energy input from the sun. And gravity, though necessary to the process, is not a source of energy. The energy came from the sun.

The fact that gravity is not diminished by all of our machinery, space satellites, etc. should tell you that all of these processes aren't stealing any energy from gravity. Now some things may steal a bit of energy from the rotating earth (they'd have to be pretty massive events), slowing it slightly. But that doesn't come from the earth's gravity and it doesn't diminish the earth's gravitational strength. The gravitational strength of the earth is strictly dependent on the mass of the earth.

Gravity is always directed toward the center of the earth. We can get energy from the wind with windmills. Couldn't we make a gravity windmill to extract that energy that is blowing toward the earth?

But don't magnets have unlimited stored energy? A refrigerator magnet will support itself on the wall of the refrigerator forever, continually exerting force against gravity to keep itself from falling. So isn't it capable of unlimited work?

Force and work are different things. Work requires motion. A force that produces no motion does no work, and consumes no energy.

Some magnet motor and magnet engine proposals have continually moving magnets. Can't these extract energy stored in the magnets?

The stored energy in a magnet is only that due to the magnet's manufacturing process. It is a small amount. In normal use, the internal stored energy of a magnet is not used or diminished at all. Heating or hammering the magnet can, however, destroy its internal domain alignments, and therefore, its magnetic effect.

Besides, if the magnet did "contain" such a tremendous amount of energy, it must have required at least that much energy to manufacture it, and magnets would be far more expensive.

It's irrelevant, but interesting, to consider just how much energy is stored in a small experimenter's magnet. That information isn't easy to find on the web. I was astounded at how small it is, and asked Rick Hoadley to do an independent calculation, which agreed with mine.

Rick considered an Alnico-5 magnet, in the form of a bar magnet is 6” x 1” x ¼” in size, a volume of about 2.5 × 10^-5 cubic meters. The energy stored in an Alnico-5 magnet bar of that size is 1.2 Joules. [If you used a stronger magnet, say an NdFeB magnet bar, it would store about 14.7 Joules.]

Quoting Rick:

1.2 Watts for 1 second is = 1.2 Joules = 1200 Watts for 1 ms. A typical hair dryer uses 1200 Watts while it is running. If there is an easy way to take the energy out of a permanent magnet with 100% efficiency, it could run the hair dryer for 1 ms. That’s all the energy in the magnet, 1.2 Joules.

If, however, you had a similarly sized NdFeB magnet, it could run the same hair dryer for almost 13 ms! Wow, one hair might get dry!

So anyone supposing they could "extract" considerable energy from magnets to solve the energy crisis had better rethink the matter.

Is centrifugal force a good energy source?

Technically, centrifugal force, Coriolis force and Euler force are called "fictitious" forces that arise from analyzing a system in a non-inertial reference frame. All physical results are the same as if the system was analyzed in an inertial frame where these fictitious forces are not present. So ficitious forces can never be the "cause" of any physical effect.

I've seen many analyses that show perpetual motion wheels can't work. When they do an analysis of forces and torques, they consider the wheel at rest, showing it is in equilibrium in any position. But if we gave it a push and set it into motion, might it continue motion, undiminished? Shouldn't we do the analysis of it in motion?

The dynamic analysis can be done, and is more lengthy and difficult—too involved to discuss here. But it reaches the same conclusion. The wheel will not exhibit continual undiminished motion unless it is spun so fast that it acts as a simple flywheel.

How about converting momentum to energy?

Could we convert angular momentum to linear momentum, or vice versa?

Rotational kinetic energy is just ordinary kinetic energy, since kinetic energy is a scalar and does not depend upon the direction of a body's motion or whether the path of a moving body is straight or curved. So there's nothing more to say about that.

Energy, angular momentum and linear momentum are all different beasts. They have separate conservation laws, different dimensions and units, and aren't convertible one to the other.

In your analysis of perpetual motion proposals you never include centripetal and centrifugal forces in the math. Isn't it possible that if you did include them, you could show that the idea could really work after all?

Centripetal force is just the radial component of real forces acting on a body. Centrifugal force is a "ficitious" force necessary when analyzing a system in a non-inertial coordinate system. Ficitious forces are never the cause of a physical effect. See my earlier comment about fictitious forces.

I've never seen an analysis that includes centrifugal force. Motion creates centrifugal force, so if we give the wheel a push, maybe the centrifugal forces of its movable parts could sustain continual motion of a wheel.

I'd like to build a prototype, but I don't have much money and don't have a machine shop.

If you are clever enough to invent such an original device, you should be clever enough to build an inexpensive prototype that would conclusively show whether it works as you expect.

Be aware that some people become so obsessed with an idea that they are blinded to all else. They spend money and time on a quest that leads only to failure. This is especially true if they choose to work in isolation, and never listen to reasonable and informed criticism of their ideas.

I've made a wheel with carefully positioned magnets, and it turns continually when I hold another magnet near it in just the right position. But when I clamp that same magnet in that same position, so I don't have to hold it steady, it doesn't work. Why?

This is often compared to the table turning or table tipping phenomena reported during séances in the heyday of Spiritualism. Gullible people sat around a table in a darkened room with their fingers pressing on a small table. They were instructed to try to prevent the table from moving. Sometimes the table moved, often vigorously. (Often with a little help from the spiritualist medium who also sat at that table.) Sensing slight motion, the sitters would try to prevent the motion, but because of the delay in their responses, they just caused a rocking periodic motion of the table.

Pendulum divination. The string is held by the fingers with the hand relaxed.	One (of many) charts for use with pendulum divination.

This has been compared to the ancient "pendulum divination" game of holding a finger ring (or mystical-looking pendant) on a string suspended from your finger. It supposedly answers questions by its mode of swing. But there's a difference. In the magnet motors and the table turning the nervous and muscular system response time plays a crucial role. In the pendulum divination game the person holding the pendulum can subconsciously (or consciously) control the nature of the motion produced with very slight finger motion. The pendulum has several modes of motion, all with very nearly the same natural frequency. If its support isn't rigid it can switch slowly from one mode to another. Also, seeing a small deviation toward a change of mode, the person holding the string can subtly encourage or discourage that to make the ring "answer" whichever way is desired. See also Ouija board.

Model of the Hamel spinner.

A neat version of this perpetual motion deception uses a large steel ball bearing with a ring magnet placed on it, the whole thing resting on a very smooth table. Another magnet is held above, causing the ball bearing to move so that the ring magnet is near the top. The ball bearing may start to rotate slowly, then speed up, as you try to hold the magnet above it in the optimum position. To make this work the magnets' strength, ball bearing weight and ring magnet strength and weight must be balanced. When turning, the ball bearing/magnet assembly is in precarious equilibrium, and just a bit of tilt will change its contact point with the table. So the device is a delicate magnet-gyroscope. Perpetual motion machine scam artists have used this in public demonstrations of the "principle" of their motors. All of these work best if the natural rotation period of the physical system matches the natural period of the hand holding the magnet. This is sometimes called "parametric excitation by hand".

I've seen this called the Hamel Spinner. For a picture of this toy, see David Hamel spinning device. Don't fuss too much about the dimensions of the parts, so long as they are in proportion to the diagram. When I first built one I used a relatively weak 1.25" ceramic ring magnet on a 2" steel ball, and a very strong magnet above, which must be strong enough to lift and keep the ball-magnet assembly upright, but not so strong that it lifts it off the table. It worked well. But I was once careless and the ball was yanked up to the magnet, breaking the ring magnet. The upper magnet need not be a ring magnet.

Rodney Brian has done some experimentation on this device. See his video and review. He shows quite persuasively that (a) the device is not over-unity, (b) energy from the hands drives it, (c) the steel ball can be replaced by a glass or plastic ball, and finally (d) magnets aren't necessary. The key to the toy's behavior is (1) A round ball, weighted above, rotating about a slightly tilted rotation axis, and (2) slightly out of phase motion of the hand, supplying energy to sustain the toy's motion.

Click here to see this effect at work in the Minato motor. Watch Minato's hands "working". There's a simple test to see what is happening. Instead of holding the magnet in the hand, clamp it to a solid support. Then the wheel, once started, will spin for a long while (like a flywheel) but eventually slow to a stop. There are many videos of such devices on the web.

I accept that the laws of physics in textbooks are well-tested, valid and correct. But we don't know everything. Maybe there are other laws that we haven't discovered yet that would not contradict other laws, but would allow for perpetual motion or over-unity performance. We could do an "end run" around the existing laws.

Can't a machine extract energy from the gravitational field? That is abundant and free.

Scientists should have learned a lesson there. But some now think of fields in the same way that they thought of the aether. They speak of "energy of the field" as if the field itself contained energy. Well, it does, in a mathematical model. But that doesn't mean that when a body in a gravitational field gains energy from gravitational forces that they are getting that energy "from the field". The energy comes from the body producing that field, not from something "in space". The same is true for electric and magnetic fields.

This is a difficult concept to explain, and many textbooks promote misconceptions about it.

Can we use gravitational sheids around parts of a machine to reduce the weight on one side continually to produce perpetual motion?

What about electric fields? There exist both positive and negative charges. Can we make an electric field shield? Yes, a completely enclosing metal Faraday cage does that. Charges separate in the metal due to the field, effectively creating a new field that can subtract from the strength of part of the existing field, while adding to the strength of another part of it. (No net energy change.) This creates an essentially field-free finite region of space. Metal objects placed in a field modify that field, but don't change the total energy of the system, except for the work required to insert the metal into place. Though it is a long story, this doesn't allow perpetual motion of over unity devices.

And so it goes with magnetic fields, though the mathematics is somwewhat different.

Might dark energy and dark matter provide unlimited sources of energy?

Suppose my machine actually produces more energy than it takes in. Might it be tapping some previously unknown energy source that's invisible all around us, one we hadn't previously detected?

I think I see why gravity wheels don't work. And magnetic wheels, too. But what if I combine gravity and magnetism?

Semantic paradoxes aside, the idea of combining natural forces is highly seductive to perpetual motion inventors. Isn't this what all successful machines do? And all attempts to achieve perpetual motion or over-unity in this way lie rusting on the trash heap of history. The bottom line is that the conservation laws of mass, energy and momentum apply to any combination of material objects. This is one generalization that one can rely upon.

I understand that an over-unity machine would violate conservation of energy. But would it also violate conservation of momentum?

When you turn on (or release) an over-unity wheel it increases its speed from zero. As it gains speed it gains rotational energy, and angular momentum, all without any energy or momentum input except the slight force of flipping the "on" switch. If it drives a vehicle, it increases linear momentum as well.

An interesting subset of unworkable devices includes those that supposedly work by creating momentum. They are called "reactionless drives", "reactionless thrusters" "fuel-less thrusters" or "internal propulsion engines". Harry Bull (1932) gained media attention with a clever demonstration with a pendulum, two masses and a spring. He claimed it moved the center of mass without any external force acting on the system. Norman Dean (1961) got a lot of press in Popular Mechanics magazine with his "Dean Drive". He was misled by the "stiction" (stick and slip) property of friction. Other such devices include Robert L. Cook's inertial propulsion system US Patent 4,238,968, and James Woodward's theoretical proposal of a reactionless propulsion system, US patent 5,289,864 and US patent 6,347,766. See Dean Drive for descriptions of the first two of these.

It is no surprise that all of these turned out to be misinterpretations of the experiments and perversions of physics to justify those misinterpretations. In all cases the devices obey classical physics, and nothing unusual is happening.

Doesn't the patent office refuse to patent perpetual motion devices?

For examples, see this sampling of patents for unworkable devices. Certain devices, like the gear and lever, and the mechanisms of Archimedes (see above) aren't patentable because they are so old and have been in common use so long.

Why not apply simple logic. A perpetual motion machine would run forever. An over unity machine would do better than that, putting out excess energy forever. That would be an infinite amount of energy over its lifetime. And there's nothing infinite in the universe, so this is an impossible thing.

What are the most important physics principles that a perpetual motion or over-unity inventor should know, but often doesn't?

1. A force does no work on a body unless it moves that body in the direction of the force. The work done on a body is W = F•d, the scalar product of the force and displacement vectors. It is of size Fd cosθ where θ is the angle between the force and displacement. So a force acting perpendicular to the direction of a body's motion does no work on that body.
2. Newton's laws and how to use them in real-world situations. Especially one needs to be able to do vector algebra properly in order to do free-body force analysis of systems. A good course in elementary physics covers that material, but many students don't ever really understand it well enough to use it properly.
3. If you think some force or torque drives your system, look carefully for reaction forces and counter-torques. You might easily overlook them in your enthusiasm.
4. Nature abhors over-unity devices. All the laws of nature conspire to make such devices impossible.
5. "Overbalanced" wheels can easily be designed that have continual imbalance of weight, force, or torque. But these don't initiate or sustain motion.
6. If a mechanical system moves through a closed cycle, and the final and initial states of the system and all its components are indistinguishable, then the wheel won't initiate or sustain motion. (Simon Stevin's Principle.)
7. If the center of mass of a wheel is always below its rotation axis in any possible position of the wheel during a cycle, then the wheel won't initiate or sustain cyclic motion.
8. If a wheel works equally well when pushed in either direction, it won't initiate or sustain continual motion by itself.

If perpetual motion and over-unity machines aren't possible, why are so many people on web forums talking about ways to do it?

Don't be so negative. Isn't anything possible if you are clever enough?

How can you be so certain that conservation laws and Newton's laws are inviolate?

Now might there be unobserved places where such laws do not apply? We can't deny the possibility. But science doesn't deal in notions that are unobserved, and especially does not deal in imagined notions that have no known connection to anything we can observe. This doesn't mean we reject such possibilities, but that we have no way to observe them or their imagined effects, so scientific confirmation is (at present) futile, and speculation about them is idle daydreaming.

For this reason, if someone tells us that there might be somewhere in the universe where gravity is proportional to 1/r³ we don't drop everything we are doing to investigate that possibility. We ask some skeptical questions first:

1. What experimental evidence suggests this idea?
2. What other reasoning supports it?
3. What experiment could be devised to measure it or its consequences?
4. How does it mathematically relate to other established and tested laws?
5. If this idea were true, how would established laws have to be modified to maintain the mathematical consistency of physics? How could we test that?

While there's a core of physics that is rock solid, critics often fail to distinguish that from the less certain areas of physics understanding. Certainly our ability to predict next week's weather is poor. This is because weather systems are complex, data is spotty and often poor, and there are just too many interacting variables to deal with. But as we improve our understanding of these processes, will any of this constitute "new physics"? No. The underlying physical laws will remain unchanged. Newton's laws will be untouched by any advances in weather forecasting.

Perpetual motion machines are powered by cranks. — D.E.S.

Advances, even revolutions, in physics have been made, such as atomic theory, relativity, and quantum mechanics. And still, the laws of conservation of momentum, angular momentum and conservation of energy still apply as firmly as ever.

The very notion of someone undertaking research to disprove Newton's laws seems absurd.

This example may seem trivial and obvious. Other examples could be cited: stellar evolution, elementary particle theory, quantum mechanics, medicine, nanotechnology. Whatever advances are made in these areas, the classical mechanics that we see operating in everyday life will remain untouched. Whenever a perpetual motion inventor presents us with a mechanical device, we know it will not violate classical physics laws, and if he claims it has 200% energy efficiency we will know he made a blunder in measurement or calculation. But if this machine has a "black box" in it that supposedly operates on "quantum principles" then the sensible thing is to independently test it. Testing methods are simple. See Testing Perpetual Motion Machines. If the test shows its energy efficiency is less than one, we conclude that those claimed quantum principles, or other magical processes, operating in that black box aren't doing anything remarkable or useful.

If subatomic particles and processes do strange things (quantum weirdness) at small scale and small time intervals couldn't this be used to build devices that do similar strange things at the macroscopic level, even to violate macroscopic laws?

Input and suggestions are welcome at the email address shown here. When responding to anything on these web pages please indicate the specific document by subject, name or filename.

© Donald E. Simanek, Feb, 2010, revised, March, 2018, August 2020.

Return to the Museum of Unworkable Devices.
Return to the main menu.