Carl Theodore Heisel
Squares the Circle.

Behold! The grand problem no longer unsolved: The circle squared beyond refutation.

"Mathematical and Geometrical Demonstrations by Carl Theodore Heisel: Disproving Numerous Theorems, Problems, Postulates, Corolleries, Axioms, and Propositions, with Ratios, Laws and Rules Hitherto Unknown in Mathematical and Geometrical Science, Naturally Growing Out of the Extraordinary and Significant Discoveries of a Lacking Link by Carl Theodore Faber, in the Demonstration of the World Renowned Pythagorean Problem Utterly Disproving Its Absolute Truth, Although Demonstrated as Such for 24 Centuries; and by this Discovery Establishing the Fact of the Existence of Perfect Harmony Between Arithmetic and Geometry as a Law of Nature, and Calculating to Settle Forever the Famous Dispute Between the Two Great Philosophical Schools." —From the title page.

Carl Theodore Heisel (1852-?).

Whenever mathematics or science declares something to be impossible, kooks come out of the woodwork to try to do it anyway. There's a rich history of such futile attempts to square the circle, duplicate the cube, trisect the angle and create perpetual motion. Such people used to be called "paradoxers".

Heisel's classic book is an homage to round-off error. He rejects the "reality" of irrational numbers. Diagrams are cleverly distorted (faked) so they appear to demonstrate that the sides of all right triangles are in integer ratio. This book is seldom seen for less than $100 on the used-book market. This is just one of several books Heisel wrote on this subject. I have a photocopy of the First Edition, 1931.

Carl Theodore Heisel (1852-?) acknowledges the earlier work of his namesake Carl Theodore Faber (1811-1887). Among Heisel's astounding mathematical discoveries is the true and exact value of the ratio of a circle's circumference to diameter: 3 13/81 = 256/81. He has only contempt for decimal notation, using it just once in his book to demonstrate that you can use it if all you want is an approximate result. You can use your calculator to show that 3 13/81 = 3.1604938... Clearly the textbook value of π = 3.1415927... is wrong. At the time of his writing, π had been calculated to some 700 decimal places, an accomplishment that Heisel considers a monumental waste of time.

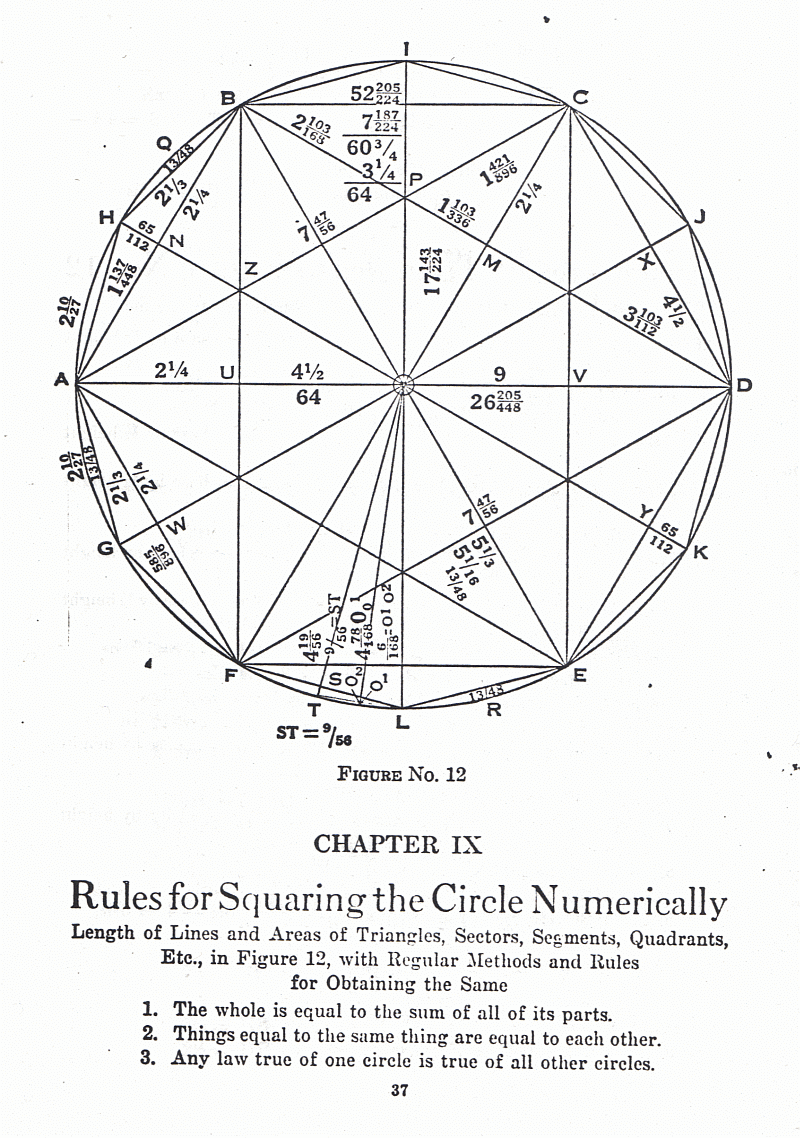
Heisel's fuzzy geometry.

But more wondrous math follows. The book is dense with numerical calculations and some elaborate geometric diagrams, with all dimensions given as integers and fractions of integers. The sides of all right triangles are in integer ratio, you just have to find the right integers. We see on page 99 a nice diagram showing a 4:4:6 right triangle. Careful examination of the figure shows that it has been subtly fudged, and the diagram also includes a 3:3:4 right triangle. Yet, several times, Heisel declares (p. 115), "I have no arguments to offer, my figures are my proofs. The laws of nature are in harmony with me and sustain me. Laugh these facts and truths away if you can."

Heisel was proud to be a 33° Mason. He said he became a Mason because "Masonry was originally a secret society based on the search for truth; and the search for lost truth should run through the life and the work of every true Mason, even as the blood runneth through his veins." Heisel says he didn't publish his ideas for profit. In fact he gave several thousand copies of his books to libraries and educational institutions, printed at his own expense. Your library may have one.

He chides the "failure of professional mathematicians to solve the problem for the last 45 hundred years..." He doesn't claim to be a mathematician, but only a practical man with common sense.

Mathematicans. Heisel says, have never disproven his demonstration that the circumference/diameter ratio of a circle is 3 13/81 or 256/81, and that the area/diameter is 9/8. The Pythagorean theorem, he says, is only true in "special cases".

Heisel's "exact" calculations.

Heisel's books are filled with tedious numeric calculations. But these are riddled with mathematical errors and hidden contradictions.

The Decimal System.

What motivates Heisel to devote his life to challenging mathematicians? His passionate mission arises from his conviction that irrational numbers are, well, simply not rational. They defy (his) common sense. All numbers are measurements, he declares, and therefore must be expressible as rational numbers. Unending decimal numbers (which Heisel calls "infinite numbers") cannot represent reality if they cannot be expressed as fractions of integers. He also rejects mathematician's idealizations such as dimensionless points and lines without width. It follows that he has no use for the decimal system, considering any results obtained with it "only approximate" and therefore inadmissible in true mathematics. "...decimals are not in harmony with the laws of nature..." (p. 84). You will not find any algebraic equations using symbolic mathematics in Heisel's books, for he rejects mathematical abstraction (p. 9). "Numerical proof is the only actual proof for any proposition, because it is seen with the mind's eye" (p. 84).

Lurking behind his tedious calculations is the conviction that areas cannot be measured in linear units, but must be an integral number of 'square units'. Therefore any number that has an irrational square root is not "natural". Its square root must be rational for Heisel to accept it.

In the history of mathematics, the decimal system of notation made calculations easier. It also enabled the introduction and adoption of the metric system of weights and measures, based on multiples of ten. This system was passionately opposed (and still is in some quarters). It is no surprise that Faber published his ideas in the International Standard a publication of the International Institute for Preserving and Protecting Anglo Saxon-Weights and Measures. Heisel, in his introduction mentions this favorably and even includes a lengthy list of "prominent scientists" who were members.

Going around in Circles.

Rule 1. Convert any given circle-diameter by the ratio nine to eight (9:8) and you have the exact square root of the circle area.

Rule 2. Multiply any given circle-diameter by the mixed number three and thirteen eighty-firsts (3 13/81) and you have the circumference; half of which, multiplied by radius, gives the circle area (according to Euclid).

Now, using textbook mathematics:

A = π'R² = (256/81)R²

√A = √(256/81)R = (16/9)R = (8/9)D

Rule 2 says that Dπ'= C and (C/2)R = A.

So A = [(2π'R)/2]R = π'R², as we expected.

Heisel declares that "the truth of the two ratios affords their own mutual test, or criterion of truth, of the respective two ratios employed. The eye may be deceived and a geometrical solution may prove false, but when seen through the mind's eye and demonstrated numerically the problem looks very different, for figures {numbers} do not lie."

This relation between his two ratios, 8/9 and 256/81, is, Heisel claims, the confirmation of their truth. He may consider this profound, but had he used symbolic algebra (which he never does) it comes as no surprise.

It only shows that [2(8/9)]² = 156/81. He could have used 4/5 and 64/25, then he'd find that [2(4/5)]² = 64/25. This calculation alone does not certify that his two ratios are correct.

So, now that Heisel has gotten off on the wrong foot on page 1 and 2, where does he go from there? Downhill, all the way.

The Grand Problem.

"The fact that over 100 years ago two of the greatest scientific societies in Europe publicly announced their belief that the solution of the "Grand Problem" of the circle quadrature is impossible will only serve largely to enhance the credit due to this great American discovery which cannot fail to be immortal, because absolutely irrefutable!" (p. 3)

Heisel's solutions arise from his consideration of the Pythagorean theorem for right triangles. Every school child learns that some right triangles have sides in integral relation, for example the 3:4:5 triangle, and it is easy to show that these obey the rule of Pythagoras: "The sum of the squares of the two legs of the triangle is equal to the square of its hypotenuse." 9 + 16 = 25 and √(25) = 5. Heisel calls these "special cases". But what about, for example, a right triangle with legs of lengths 4 and 5? The sum of the squares is 16 + 25 = 34. So √(34) = 5.8309519..., an unending decimal—an irrational number. Heisel will not accept this, for, he says, every length, even that of this hypotenuse, must be expressible as a "finite number" (i.e., not an unending decimal).

So how does Heisel avoid irrational numbers? He explains on page 5:

An infinite root must strike every reflective mind as utterly incompatible with a conception of form, the very element of geometry, a science which can have no possible meaning in the formless, in which there can be no difference. A so-called "hypothenuse," then must be a finite quantity. This necessity led to the final discovery of an artificial root of an irrational quantity, with the following rule for determining the same, viz.: First—From any given irrational quantity extract the largest square, calling the root of the same = a.

Second—Dividing the difference between the given quantity and the square by twice a, calling this fraction = b. This a + b root, when squared, is equal [to the] given irrational quantity +b², therefore the irrational quantity is = a² + 2ab forever. The new law is simply a deduction from this rule and defies all science to upset it.

Let's use Heisel's rules to find the square root of 5. His table says the square root of 5 is 2 1/4. He calculates 2² + (1/4)² + 2[2(1/4)] = 4 + 1 = 5. So 5, Heisel says, is the square of 2 1/4, and 2 1/4 is therefore the square root of 5. He has thrown away the b² term in (a + b)² = a² + 2ab + b².

Let's check another entry in his table. Consider the square root of 50. His table gives 7 1/14. His rule gives a=7, b=1/14, so we have 49 + 2(1/14)7 = 49 + 1 = 50. Wow! Agreement! But let's not be seduced by one or a few cases.

Let's try a larger number, say 99. The integer that gives a square closest to 99 is 9, whose square is 81. Then a = 9 and b = 19. Heisel's rule gives 81 + 2(9)19 = 81 + 342 = 423. Whoops! The rule doesn't seem to work when b is large. Heisel's table gives the root as 9 18/18. Even in Heisel's screwy mathematics, 18/18 is still equal to 1. So his table is saying that the square root of 99 is 10, identical to the square root of 100. We see the same thing in his tabulated values for roots of 8, 15, 24, 35, 48, 63 and 80, whose squares are all one less than squares of the next higher integer. These cannot be dismissed as typographical errors. Similar errors (fudging) are found throughout his books. One wonders how many people looked at this table on p. 87 and failed to notice these obvious contradictions.

Summary.

If the mathematical scientists in their narrowness and prejudice do not willingly acknowledge this new and exact 3 13/81 ratio, then the thinker, the student and the world at large will eventually acknowledge it in spite of them, and ridicule and laugh at them in the future.

—Donald E. Simanek, July 2014.

Notes.