A-3: UNIT SYSTEMS
I: UNITS
Sciences are built upon measurements. Measurements are expressed with numbers. This allows the logic, precision and power of mathematics to be brought to bear on our study of nature.
Units of measurement are names which characterize the kind of measurement and the standard of comparison to which each is related. So, when we see a measurement expressed as "7.5 feet" we immediately recognize it as a measurement of length, expressed in the unit "foot" (rather than other possible length units such as yard, mile, meter, etc.) Since many possible units are available for any measurement it is essential that every measurement include the unit name. A statement such as "the length is 7.5" is ambiguous, and therefore meaningless.
II: THE METRIC SYSTEM
As early as 1670, European scientists were recommending reform of the chaotic unit systems then in use: systems which differed from country to country. The reformers urged (l) uniformity and universality, (2) simple ratios of sizes of units, (3) rational relations between units, and (4) units referenced to constants of nature (such as the circumference of the earth, boiling point of water, etc.)
In 1791, in the aftermath of the Revolution, the French National Assembly adopted a more rational system based upon decimal ratios. This came to be known as the metric system. In the United States, at this time, there was also interest in reform of units and standards. In 1786 Congress approved a decimal system of coinage. In 1790 Congress considered a report on units which Secretary of State Thomas Jefferson had prepared at the urging of George Washington. In the report Jefferson proposed, as one alternative, a decimal system of weights and measures. His system had several unfortunate features, (1) it retained some of the old unit names (pound, foot, inch, furlong, mile, etc.) but assigned them new sizes (1 foot contained 10 inches, for example), and (2) his system was not fully compatible with the metric system then being developed in France. Congress, confused and ill-informed (as usual) took no action on the proposal.
John Quincy Adams' 1821 Report Upon Weights and Measures was an exhaustive study, presenting pros and cons of unit reform. Though praising the virtues of the French Metric system (and noting some shortcomings) he concluded that the U. S. had not attained sufficient maturity to require adoption of the system. Further he noted that the states had laws of weights and measures which were substantially uniform. To impose a new system on all states would raise sticky questions of states' rights.
So our best opportunity for adoption of a sensible unit system slipped by. While other countries, one by one, adopted the metric system, the U. S. arrogantly went its own way, feeling no need nor desire to adopt a "foreign" system of units.
In 1890, metric units were established as the legal basis of all weights and measures in the United States, but this did little to establish the use of the metric units in industry, commerce, and everyday life. Today the revised and standardized metric system, called the international system (SI, for Systemé International) is used in nearly all countries. The United States, South Africa and less than a dozen non-industrialized countries have not made a commitment to convert fully to the metric system.
But change is coming—"slowly. Several states have marked their highway distance signs in both miles and kilometers. Some radio stations report daily temperatures in both degrees Fahrenheit and Celsius. U. S. automakers use metric parts in automobile engines. Science and medicine have been almost exclusively metric in all countries for many years. Other industries use metric standards because of international trade and competition.
The relative simplicity of the metric system is well illustrated by comparing measurements of length in the United States System with those of the metric system.
U. S. SYSTEM METRIC SYSTEM
1 mile = 5280 feet 1 kilometer = 1000 meter
1 mile = 1760 yards 1 hectometer = 100 meter
1 rod = 5.5 yards 1 dekameter = 10 meters
1 yard = 3 feet 1 decimeter = 1/10 meter
1 foot = 12 inches 1 centimeter = 1/100 meter
1 millimeter = 1/1000 meter
1 gallon = 4 quarts 1 liter = 1000 milliliters
1 quart = 32 fluid ounces
1 fluid ounce = 8 drams
The sizes of U. S. units usually have no simple relation to each other, and the relations lack consistency. They are therefore difficult to remember. The metric units are consistently constructed and consistently named. The metric system is based upon multiples and submult- iples of 10 (very convenient for calculation in our decimal system). Also, the unit names have prefixes which consistently indicate which multiple of 10 is meant. The prefixes keep the same meanings throughout the metric system. The table on the next page shows this simplicity and consistency which is the strength of the metric system.
Some of the prefixes may already he familiar. "Deci" appears in our word "decimal." "Centi" appears in our monetary system as "cent," the hundredth part of a dollar. In tax assessments one encounters the "mill" which is 1/10 cent, or a thousandth of a dollar. The prefixes even appear in slang words, as in "megabucks" for a large amount of money.
Each different kind of measurement has a root name, from which other names may be constructed by combining a root name with a metric prefix. Three root names are commonly used in introductory physics courses:
meter, a length unit
gram, a mass unit
liter, a unit of capacity (volume)
Names for other sizes of these three types of measurement are obtained by applying the metric prefixes. The starred names below are the only ones commonly used in physics.
kilometer* kilogram* kiloliter hectometer hectogram hectoliter dekameter dekagram dekaliter meter* gram* liter* decimeter decigram deciliter centimeter* centigram centiliter millimeter milligram milliliter*
Metric Prefixes.
EXPONENTIAL
PREFIX SYMBOL MEANING NOTATION
Yotta Y 1024
Zetta Z one billion billion billion 1021
Exa E one billion billion lO18
Peta P one million billion 1015
Tera T one thousand billion 1012
Giga G one billion 109>
Mega M one million 106
Kilo* k one thousand 103
Hecto h one hundred 102
Deka da ten 101
Deci* d one tenth 10-1
Centi* c one hundredth 10-2
Milli* m one thousandth 10-3
Micro μ one millionth 10-6
Nano n one billionth 10-9
Pico p one thousandth billionth 10-12
Femto f one millionth billionth 10-15
Atto a one billionth billionth 10-18
Zepto z 10-21
Yocto y 10-24
* Most commonly encountered prefixes; learn these first.
The liter is really an unnecessary unit, since volumes may be expressed in cubic length units. But the liter is so entrenched in common usage, that it will probably persist for many years. One liter equals 1000.028 cubic centimeters, but in all but the most precise work the approximate relation, 1 liter = 1000 cubic centimeters is precise enough.
To give a feeling for the sizes of the metric units, we list some approximate relations between U. S. and metric units. The exact relations would require more decimal places.
1 meter = 1.09 yards
1 cm = 0.394 inches
1 kilogram mass weighs about 2.20 pounds avoirdupois
1 liter = 1.06 liquid quarts
80 kilometers = 50 miles
SPELLING AND PRONUNCIATION OF METRIC NAMES: All metric prefixes have a single syllable, and metric names with prefixes always have their emphasis on the first syllable (the prefix). Say "keelo-meter," not kee-lahm-iter. (The prefix is never broken into two syllables.)
In the United States we have long used the spelling "meter" and "liter." In Europe these are spelled "metre" and "litre." There is now a lively argument over whether we should conform to the European spelling, or whether these spelling variants can be allowed, since they are unlikely to cause confusion. Most U. S. texts still prefer the "-er" form. Watch for future developments.
III: BASIC MEASURABLES, DIMENSIONS
Measurable physical quantities (measurables) are of two kinds:
(1) BASIC (or FUNDAMENTAL) MEASURABLES. Those so directly connected with measurement that they are defined by specifying a measurement process.
(2) DEFINED MEASURABLES. These are defined by mathematical equations in terms of other previously defined and/or fundamental measurables.
The measurables of the physical sciences are interwoven into a tight net of equations and definitions. Remarkably few are so fundamental that they cannot be defined in terms of others. Those few, the basic measurables, are defined through operational definitions (by specifying a measurement process).
Only three basic measurables are required in mechanics. Length and time are always chosen as basic. Some unit systems choose force as the third basic measurable, others choose mass instead.
But as physics developed beyond mechanics, other basic measurables were added to the list; temperature, electric current, the mole, and the luminous intensity of light are often chosen.
The basic measurables are called the dimensions of the system. The use of this word is analogous to its use in analytic geometry. In space, any point can be specified by its coordinates measured along axes of a three dimensional coordinate system. Likewise, in a system of measurables, any measurable may be expressed as an algebraic combination of the basic measurables (dimensions) of the system.
A few examples may clarify this. In elementary mechanics we take length, mass and time as the dimensions of the system. For brevity these are abbreviated L, M, and T. The dimensions of other measurables can be determined by looking at their defining equations. Velocity is defined as the quotient of length and time; the dimensions of velocity are therefore L/T, usually written LT-1. Acceleration is defined as the quotient of velocity and time, so its dimensions are LT-2.
Force is defined as the product of mass and acceleration; its dimensions are therefore MLT-2. The dimensions of energy are obtained either from the kinetic energy expression, or from the definition of work: force times displacement. In either case, the result is ML2T-2.
The dimensions of a quantity tell us nothing about its units. The dimensions arise from the logical (mathematical) interrelations between quantities, reflecting the structure of physical laws and definitions. We will see how dimensions can be helpful for checking equations for consistency, and even in some cases for predicting the mathematical form of equations.
IV: COHERENT UNIT SYSTEMS
As we construct equations involving measurables we discover that our choice of units can influence the form of an equation. Consider this empirical equation from engineering.
d = 67.39h - 0.33
Here d represents the distance in feet at which a road sign is generally legible to an automobile driver, h is the height of the lettering in inches.
Clearly this equation requires a statement of what units are used for b and h. If d were in miles and h in feet, the equation simply would not give physically correct answers! However, it could work for a different choice of units of we were to modify the values of the constants.
d = K1(K2h) = K3
By finding suitable values of the constants K1, K2, and K3, this equation could be made to work with any units for d and h, but each choice of units would require a different set of constants.
To avoid such nuisances we agree upon a "standard" set of units. This allows us to write just one equation for each physical law.
Such a standard set of units is called a coherent unit system. A coherent system is built by choosing appropriately sized units for each of the basic measurables. The units of other measurables will then be determined by their defining equations, as combinations of the units of the base measurables, in the same manner as dimensions are determined.
When a coherent system is built from a basic unit set which includes mass, the system is called absolute. If the basic unit set includes force, rather than mass, the system is called gravitational. The International Metric System is an absolute system. Its basic units are the meter, kilogram, and second. It is called an MKS system.
A number of coherent systems have been used in the past, and are still used to some extent. A few decades ago the cgs (centimeter-gram-second) system was standard in physics. Some branches of engineering still use a U. S. (or British) system, the FPS (foot-pound-second) system, which is a gravitational system of units.
Fortunately these three systems (MKS, cgs, and FPS) are all mutually coherent for most branches of physics, especially mechanics (but not including electricity and magnetism). In mechanics the equations have the same form in all three systems, but one must be careful to express all constants (like Newton's gravitational constant) with a value appropriate to the system being used.
If all equations were written with a sufficient number of constants, tables of values of these constants could be constructed for any system of units. The constants would generally have units, and might have dimensions as well.
V: UNIT CONVERSIONS
Data and measurements may be expressed in any units, usually chosen for convenience of size. But when this data is used in physical equations, it must be converted to the units required by the coherent system chosen. Units must also be converted when translating from one coherent system to a different one. This process of unit conversion is not conceptually difficult, but the student would be wise to adopt a systematic procedure which will avoid time-wasting blunders.
Unit conversions begin with equations which relate sizes of units, for example
1 yard = 3 feet
is obviously correct, and so simple in appearance that the reader is likely to dismiss it as trivial. But such physical equations relating measurables are significantly different from equations relating pure numbers.
The above equation tells us that the measurement "1 yard" is equal (equivalent to) the measurement "3 feet." To write simply 1=3 would be incorrect (and absurd!). Equation (3), relating measurements, is correct even though neither the numeric parts nor the unit parts are equal.
Equations relating measurements are manipulated by the ordinary rules of algebra, and the units are carried along according to the same rules. For example, if both sides of Eq. (3) are divided by 1 yard, the result is:
Therefore:3 feet feet 1 = —————— = 3 ———— 1 yard yard
1 = 3 feet/yard
This last expression represents an identity relation for measurements. We call it a conversion factor. In algebra it is often convenient to multiply an expression by another expression which is equal to one. When doing unit conversions, expressions may be multiplied by conversion factors, since they are physically equal to one. Suppose we had a measurement of 2.5 yards which we needed to express in feet. We would simply multiply by the appropriate conversion factor:
2.5 yards = 2.5 yards (3 feet/yard) = 7.5 feet
The unit "yard" has canceled by the rules of algebra, leaving a result in feet.
Example 1: Suppose an equation gives the result
velocity = 1.1 inches/second
but we want an answer in feet per second. The conversion equation between feet and inches is
1 foot = 12 inches.
Dividing both sides by 1 foot gives:
But we actually need its reciprocal: 1 = (1/12) feet/inch1 foot 12 inches/foot inches ——————— = 1 = —————————————— = 12 —————— 1 foot 1 foot foot
(The distinction between "foot" and "feet" is grammatical, of no concern in these calculations).
Now multiply our measurement by the conversion factor:
Example 2: Often a string of conversion factors will be required. Suppose we wanted to convert 1 mile/hour to its equivalent in ft/sec.
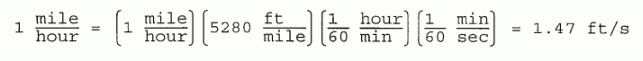 |
Conversion factors may be inserted as a factor multiplying any part of an equation, or the entire equation. They do not alter the physical truth of the equation, since they are physically equal to 1, and are dimensionless.
In the physical sciences, nearly all equations are constructed in such a manner that the dimensions balance on both sides. That is, all terms which are added together have the same dimensions. Such equations are called homogeneous. Since conversion factors are dimensionless, their use in an homogeneous equation does not destroy its homogeneity. (Conversion equations and factors are themselves homogeneous.)
When a physical equation is expressed with all quantities in one coherent unit system, the units will also balance on both sides. You will notice that the units do not balance on both sides of a conversion equation. Conversion equations are not coherent. Their primary use is to convert an incoherent physical equation into a coherent one, or to convert a coherent equation into an equivalent equation in a different coherent system.
VI: SUMMARY
1. An equation in which the units balance on both sides of the equal sign is called coherent.
2. An equation in which the dimensions balance on both sides of the equal sign is called homogeneous.
3. A unit system constructed so that all physical laws are represented by coherent equations is called a coherent unit system. Physics, chemistry, and most of engineering are built upon coherent systems.
4. Conversion factors are homogeneous, but may be incoherent. Their primary use is to transform equations from one coherent unit set to another.
5. Such "relations" as "one cubic centimeter of water = one gram" are inhomogeneous and incoherent, for they relate units of different dimensions and different physical meaning. An "equality" relation such as this represents a more relaxed meaning of "equal" than is normally permissible, though if one is careful such "conversion equations" can be used to get correct results. Such "equations" are, however, conceptually misleading, and are best avoided at the introductory level of physics. It is better to say "one cubic centimeter of water has a mass of one gram."
The tables below directly compare the commonly encountered coherent systems of units. The table of electrical units also includes conversion factors.
VII: PHYSICAL UNITS: MECHANICS
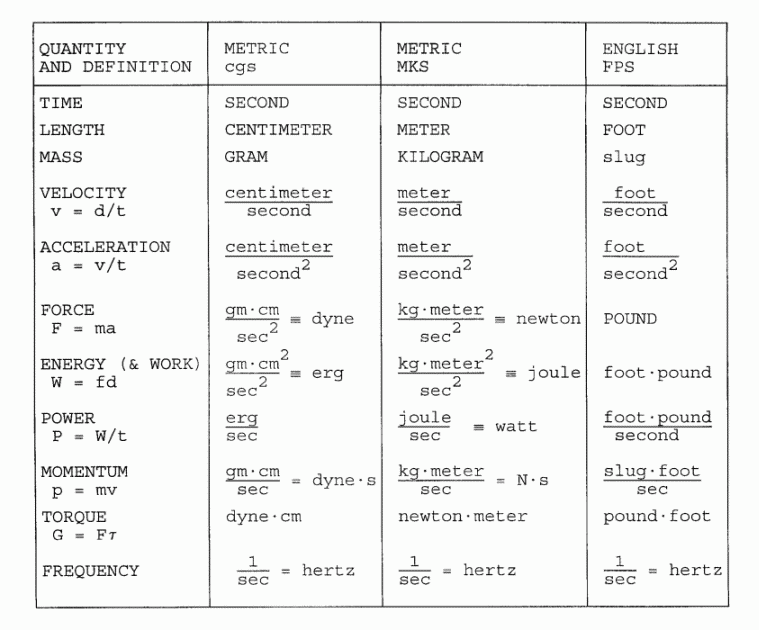 |
STANDARD ABBREVIATIONS FOR UNIT NAMES:
s = second N = newton V = volt cm = centimeter lb = pound W = ohm m = meter J = joule W = watt ft = foot Hz = hertz A = Ampere g = gram mi = mile C = coulomb kg = kilogram
Note 1. Unit names derived from the names of persons are not capitalized,
while the corresponding unit abbreviations are capitalized.
Note 2. The centered dot representing multiplication of units is often replaced by a dash
"-". This cannot be confused with a minus sign, since unlike units are never added
or subtracted.
VIII: PHYSICAL UNITS: ELECTRICITY AND MAGNETISM
Use of the conversion factors: Suppose you wanted to convert a quantity expressed in statcoulombs into its equivalent in abcoulombs. Multiply the measurement in statcoulombs by the conversion factor 1/c to get abcoulombs. When proceeding from left to right in the table, the unit conversion factors are used directly.
In going from right to left, use the reciprocal of the conversion factor. So, in converting amperes to abamperes, divide by 10.
A column of esu/mks conversion factors is included for convenience. These entries are simply the product of the reciprocals of the other two conversion factor column entries.
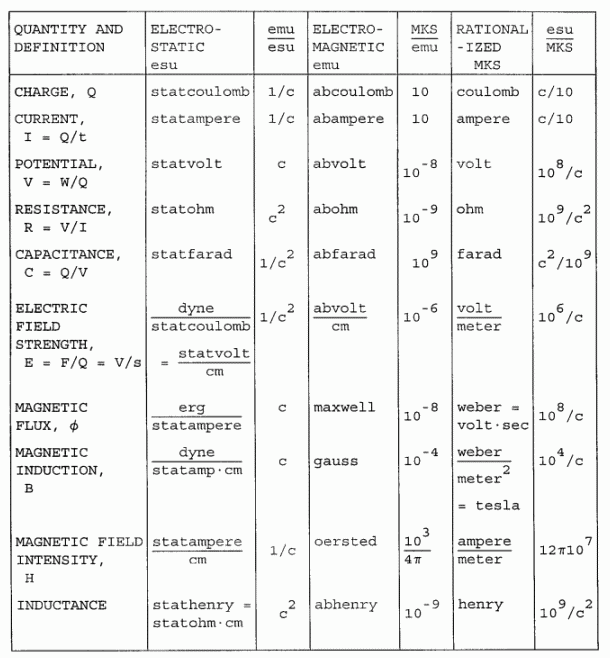 |
NOTE: c is 2.9979 x 1010, the cgs value of the speed of light.
IX: RULES FOR DIMENSIONS AND UNITS
1. Dimensions combine by the ordinary rules of algebra. Units do also.
2. Terms which are added or subtracted must have the same dimensions and the same units.
3. Quantities on either side of the equal sign must have the same dimensions and the same units.
4. Powers are dimensionless and unitless (though factors within them may have dimensions and units).
5. dy/dx and ∂y/∂x have the dimensions and the units of y/x (look at the formula for the definition of the derivative).
6. ∫ y dx has the dimensions and the units of yx.
7. Arguments of sin, cos, tan, log, etc. must be dimensionless, but may have units.
8. Sin, cos, tan, log, etc. are dimensionless and unitless.
9. The mathematical constants π and e are dimensionless and unitless.
Test your understanding by classifying each of the following equations as homogeneous or inhomogeneous, coherent or incoherent.
1. 1 mile = 5280 feet
2. Length of table = 1 yard + 2 feet + 4 1/2 inches
3. 1 kilogram = 2.2 pounds
Answers:
1. Homogeneous and incoherent. This is a correct conversion equation.
2. Homogeneous and incoherent. This equation is perfectly proper and unambiguous.
3. Inhomogeneous and incoherent (and improper!). We should avoid equating apples and oranges, unless we are interested in counting fruit! The kilogram is a unit of mass, the pound is a unit of force. We should say, "A one kilogram mass at the earth's surface would weigh 2.2 pounds."
X: DlMENSIONLESS MEASURABLES AND OTHER ODDITIES
We define some measurables in such a way that they are dimensionless. Some of these are given unit names, and some are not. Specific gravity, being a ratio of two densities, is dimensionless. It has no unit name. Index of refraction, a ratio of two speeds (of light), also has no unit name. Pi (p), the ratio of a circle's circumference to its diameter is therefore dimensionless and has no unit name.
But the radian (a measure of angle) is defined as the ratio of arc length to radius, so its dimensions are L/L, that is, it is dimensionless. Yet it is given a name, the radian. The name helps to distinguish this measure from other angular measurements such as "degrees of arc" (which are also dimensionless).
Sometimes measurables of physically different quantities have the same dimensions. The commonest example is work and torque: both result from multiplying force by distance. In these cases the unit names are often assigned in a distinctive manner. Names of work units, erg, Newton, etc. are never used for torques. In the "English" unit system, the work unit is called the "foot-pound" and the torque unit is called the "pound-foot."
It is also possible for different quantities with different unit names to have the same dimensions. The quantity luminous flux has the unit lumen. A light's source strength is expressed in the unit candela. A one candela source is said to emit 4π lumens. We can write 4πC where C is the source strength and F is the flux. 4π is dimensionless, so C and F have the same dimensions, even though representing distinctly different quantities with different unit names.
XI: REFERENCES ON UNIT SYSTEMS AND DIMENSIONAL ANALYSIS
(1) Bridgman.P. W. Dimensional Analysis, 2nd Ed. Yale U. Press, 1931.
(2) Chertov, A. G. Units of Measurement of Physical Quantities. Hayden, 1964.
(3) Dimone, Daniel V, and Treat. A History of the Metric System Controversy in the United States. U. S. National Bureau of Standards, 1971.
(4) Isaacson, E. de St. Q, and Isaacson. Dimensional Methods in Engineering and Physics. Edward Arnold, 1975.
(5) Jefimenko, Oleg D. Electricity and Magnetism. Appleton Century-Crofts, 1966. Appendix I.
(6) Scott, William Taussig. The Physics of Electricity and Magnetism. Wiley, 1966. Appendix A7, Electrical Units.
(7) Reitz, John R., Milford and Christy. Foundations of Electromagnetic Theory. Third Ed. Addison-Wesley, 1980. Appendix II. Systems of Units. ISBN 0-201-06332-8.
(8) Dale Corson and Paul Lorrain. Introduction to Electromagnetic Fields and Waves. Freeman, 1962. Appendix C. Conversion Table.
(9) Symbols, Units, and Nomenclature in Physics, Document U. I. P. 11 (S. U. N. 65-3) International Union of Pure and Applied Physics. (Reprinted in the Handbook of Chemistry and Physics, Chemical Rubber Co.)
(10) Pankhurst, R. C. Dimensional Analysis and Scale Factors. Chapman & Hall, Reinhold 1964.
(11) Perry, John. The Story of Standards. Funk and Wagnalls, 1955.
XII: UNIT CONVERSION EXERCISES:
Supply the appropriate conversion factors in the square brackets. Find the numeric value to complete the right side of the equation. The answers are on the last page.
Example: Problem: 5 yards [ ] = [ ] ft.
Answer: 5 yards [3 ft/yd] = 15 ft.
PROBLEMS:
C-1. 9 ft [ ] = [ ] yds
C-2. 6 dm [ ] = [ ] cm
C-3. 7 lb [ ] = [ ] cm
C-4. 4 oz [ ] = [ ] lb
C-5. 3 minutes [ ] = [ ] seconds
C-6. 1 hour [ ] [ ] = [ ] seconds
C-7. 6 seconds [ ] = [ ] hour
C-8. 1 day [ ] [ ] = [ ] sec
C-9. 1 year [ ] [ ] [ ] = [ ] sec
C-10. 7.896 m [ ] = [ ] cm
C-11. 3.428 km [ ] = [ ] cm
C-12. 8.529 km [ ] = [ ] ft
C-13. 20 miles/hr [ ] = [ ] miles/sec
C-14. 5 cm/sec [ ] = [ ] m/s
C-15. 1 mile = 1.61 km (approx), therefore: 1 mile/hr [ ] = [ ] km/hr
C-16. 1 mile = 5280 ft, therefore 1 mile/hr [ ] [ ] = ft/s
C-17. 1 mile/hr [ ] [ ] = m/s
C-18. 1 cm3 [ ] = [ ] mm3
C-19. 55.89 m3 [ ] = [ ] cm3
C-20. 1 km3 [ ] = [ ] cm3
SOME OF THE ANSWERS:
C-1. 9 ft [1/3 yd/ft] = [3] yds
C-2. 6 dm [100 mm/dm] = [600] mm
C-8. 1 yr [365 day/yr][24 hr/day][60 min/hr][60 sec/min] = 31,536,000 seconds/yr. Some students use π×107 as an approximate value, easy to enter into their calculators. It's a curious approximation of no physical significance.
C-10. 7.896 m [100 cm/m] = [789.6] cm
C-13. 20 miles/hr [(1/3600) hr/sec] = [1/180] miles/sec
© 1996, 2004 by Donald E. Simanek.