K-1 THE ACCELERATION DUE TO GRAVITY

Instructor's notes.

[This experiment was previously numbered M-5.]

This is the standard free-fall experiment using the Behr apparatus.

One set-up of the apparatus is sufficient for a class of usual size. The instructor should explain the apparatus and operate it once to obtain a data tape, then let each group in turn run a tape. It takes only 3 to 4 minutes for inexperienced students to run a tape once they have seen it done. Another 30 to 40 minutes is enough to record the data from the tape into the lab notebook. The remaining time in lab may be devoted to a preliminary analysis, to obtain a value of g, to ascertain that the data is good, and the method understood. The remaining analysis can be done as homework.

GRAPHICAL METHOD

Many students ask whether to plot the velocities at the end, middle, or start of each time interval. I'll admit I've gotten tired of explaing that it doesn't matter, so I simply tell them to plot at the middle. Therefore I removed the following paragraphs from earlier versions of these instructions:

You needn't worry about whether to plot the velocity at the end, middle, or beginning of each interval. All that matters is that you be consistent, for a constant shift of the entire graph along the time axis does not affect the fact that it's a straight line, and does not change the value of its slope. It's the slope we want to measure.

However, it does affect the graphical determination of the actual zero point, the time when the plummet started to fall, so I don't ask students to find this, as I had in previous years.

ANALYTIC METHODS

There are more sophisticated methods of data analysis than described here. These have not been included for several reasons. The "best" methods are often somewhat obscure, and will be used by the student as "magic formulae" which happen to give the "answer." These methods are often unique to this specific experiment, and have very little transfer value to other experiments.

The best methods use long time intervals, about half the time of fall, to minimize fractional error in each interval.

Method 3 arose from my review of Bernard and Epp's laboratory manual, Laboratory Experiments in College Physics (5th edition, Wiley, 1980), which had committed the fatal error of recommending the "method of differences." I had contracted to review this book for the publisher. I responded as follows:

...the data analysis recommended for this experiment is defective. In step 5, the calculation of the acceleration, the method seems to be using more data than it actually is. The time intervals are all equal, call them Δt. Then in the calculation of, say a₂, you'd have:

    v  -  v     s - s  - (s - s )  s  - s 
     3     1     4   2     2   0    4    0
a = ———————— = —————————————————— = ———————
       Δt            (Δt)2           (Δt)2

This is the inherent defect in the simple "method of differences" approach, it does not really use all of the data which are input to the equations, intermediate values cancel out. If one then goes on to step 6 and calculate the mean acceleration, all data points cancel out except the first two and the last two. I made this mistake once myself, which made me more alert to the many AJP articles which have appeared describing other methods of data reduction which do not have this defect.

The flaw is hidden here because the time intervals were written in terms of the endpoints of the interval. But in most timing systems one assumes that the time intervals are indeed equal (within their experimental uncertainty). The numbers one puts into these equations will indeed give identical denominators in every case.

I've gotten a few gray hairs over the years worrying about what is the best way to reduce this data. The method must also be clear enough to be understood by freshmen. The trick is to ensure that one takes data intervals long enough to have inherently low error propagation, yet statistically independent of each other (not having common endpoints). I'll suggest one that seems feasible:

(1) Group the data into 16 equal time intervals Δt (instead of the 8 you suggest).

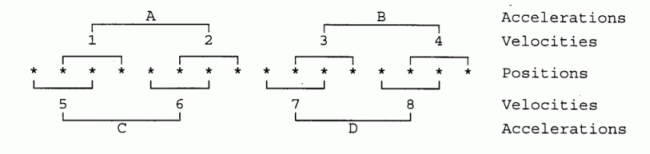Fig. 3. Data point grouping for method three.

Calculate velocities over the intervals labeled 1 through 8. These will have 2Δt in the denominator, for the time interval is 2Δt. These velocities are clearly independent, for no two velocity calculations used the same position data points.

Now calculate four accelerations, labeled A through D in the diagram. They will all have 4Δt in the denominator. These accelerations are clearly independent. Average them. No position data has been used twice, so there is no possibility of data cancellation in the computation. Also, from the point of view of error analysis, it gives roughly equal weighting over the whole span of the data.

Apparently I wasn't sufficiently clear. Although quite a number of my other suggestions were implemented in the sixth and seventh editions of Bernard and Epp's manual, they ignored this one, and the mistaken method persists to trap new multitudes of innocent students. Oh, well. they paid me for the pre-publication review.

BIBLIOGRAPHY

Humphrey, Clyde L. "Analysis of the Free Fall Experiment." Am. J. Phys. 41, 965 (1973).

Heald, Mark A. "Comment On: 'Analysis of the Free Fall Experiment'." Am. J. Phys. 42, 1121.

Barker, David R. and L. M. Diana. "Simple Method for Fitting Data when Both Variables Have Uncertainties." Am. J. Phys. 42, 224 (1974).

ANSWERS TO QUESTIONS

(1) On the basis of your data, calculations and graphs can you say that the acceleration of the plummet was constant? Within what experimental error?

Yes, the plummet acceleration is constant, as shown by the linearity of the velocity graph. The experimental error shows on that graph as the scatter of the points, and their deviation from the fitted straight line.

In grading this question, look to see whether the student has (a) supported the answer by citing experimental data, graphs, and calculations, and (b) that those really do support the conclusion.

(2) Considering the "scatter" of the individual acceleration values, and the scatter of the points on your graphs, what error estimate (in %) would you give for your value of g?

Again check whether the student's cited evidence supports the conclusion. 1% to 3% is reasonable.

(3) Compare your value of g with that in the CRC handbook. Look up Helmert's equation in the handbook. You'll need to know that LHU is at 41° 8' North latitude, and the parking lot behind the physics is 580 feet above sea level. [You can check this data by consulting a U. S. Coast and Geodetic Survey map of the Lock Haven area.] This data, used in Helmert's equation, gives you the "accepted" value of g with which to compare yours. What is the percent discrepancy between your value and the accepted value?

In a CRC handbook like mine, there's no index entry for Helmert's equation. Look up "acceleration due to gravity at any latitude and elevation, equation." It is in the "definitions and formulas" section.

Helmert's equation gives g, taking in account the centrifugal effect (second term) the earth's oblateness (third term) and the elevation above sea level (fourth term). The first term is the value of g at sea level at latitude 45°. Helmert's equation is (in cgs units):

g = 980.616 - 2.5928cos(2φ) + 0.0069cos2(2φ) - 3.086x10-6 H

where φ is the latitude, H the elevation in centimeters (above sea level).

LHU is at latitude 41° 8' N.

Ulmer Hall parking lot is 580 ft above sea level.

1 ft = 30.48 cm

g = 980.616 - 2.5928(0.13514) + 0.0069(0.01826) - 3.0806x10-6(1.65x104)

This gives g = 980.3 cm/sec²

(4) Suppose students at the University of Alaska did this experiment with the same apparatus. Would you expect them to obtain the same numerical result you did? Be specific in explaining why or why not. What about students in the class of 2025 at Lunar Tech, on the moon?

From the Chemistry-Physics Handbook,

Quiet Harbor, Alaska, 56° lat, 132° long, 4m alt. g=981.5 to 981.59 State College, PA, 40° 47.9' lat, 77° 51.8' long, 358 meter alt. g=980.127

Height of Mt. Everest is 28,028 ft = 8848 m
Height of Mt. McKinley is 20,320 ft = 6194 m

My World Almanac says that U. of Alaska is located in the town named College, Alaska. Very helpful! My Information Please Almanac says there are two campuses, at Anchorage and at Fairbanks. Anchorage is a coastal town, Fairbanks is well inland. The value for Quiet Harbor should be a reasonable estimate, 981.6 cm/s². Even on top of Mt. McKinley g = 979.7 cm/s². Bottom line: the Alaskan students would get a larger value of g, primarily because they are closer to the center of the earth and closer to the axis of rotation of the earth. The inverse square law of gravitational force and the centrifugal effect of earth's rotation are responsible.

On the moon, the acceleration of a falling body would be much less, because of the smaller gravitational force. The adventurous students will calculate how much less: The moon's mass is 1/81 that of the earth and its diameter is about 1/4 that of the earth (radii in same ratio). Therefore the gravitational force, and the acceleration, at its surface is (1/81)(16) or approximately 1/6 that on the surface of the earth. [Centrifugal effects are much smaller than on earth partly because the rotation rate of the moon is about 1/30 that of earth and partly because of the smaller radius. ac = v²/r, so centrifugal acceleration is 1/(30² × 4) = 1/3600 as on earth.]

[Students should be expected to give quantitative responses to these questions, and use "rough" calculations as appropriate to the implied intent of the question. They should look up the data they need, use the library, and relate the questions to their experience. For example, they might relate this to the jumping and golfing antics of the astronauts on the moon.]

(5) [Removed] Have you demonstrated the constancy of the acceleration due to gravity? No, you haven't. What have you demonstrated, in this experiment? Keep in mind that one should not claim more than is justified by the experiment.

The student has demonstrated (perhaps) the constancy of the acceleration of the plummet over the 1.5 meter path in the LHU physics lab. The student has also obtained a measurement of the value of the acceleration due to gravity in the LHU physics lab.

(6) [Removed, difficult to evaluate responses.] In this experiment the plummet was released from rest. A clever student wants to eliminate the closely spaced and unreliable sparks at the beginning of the tape. This student designs a plummet release mechanism which gives the plummet a downward push, only during a short interval before the beginning of the spark record. This makes the plummet velocity larger at the beginning of the spark tape. The student's lab partner worries that this might affect the experimental value of the acceleration due to gravity. What effect would it have on the calculated values of velocity? What effect would it have on the calculated value of g?

Initial velocity has no effect on the calculated value of g, for during the free fall, the only accelerating force is gravity. The velocities would be larger, however, and the sparks spaced farther apart, but the changes in velocity, from interval to interval, would remain the same.

QUESTIONS FROM WALL, LEVINE AND CHRISTENSEN

(1) An error of 0.01% in the time intervals produced by the spark timer would produce what error in the value of the acceleration?

The error in g will depend on what data analysis method was used, and the student should show how his answer to this question was derived from that particular method, i.e., from the error equation(s) for that method. However, one can say, that in any method, since the error term for time has the square of t, then it will contribute at least 0.02% to the error in g, all else being equal.

(2) [Calculus] If S is a quadratic function of time t, i. e., S = α + βt + γt², show (using calculus) that v is a linear function of t and that the acceleration is constant.

v = dS/dt = β + 2γt, and a = dv/dt = γ

QUESTIONS FROM OTHER SOURCES

(1) If the student plotted average velocity vs. time, at what value of time should each average velocity be plotted? At the beginning of the time interval, at the middle, at the end, or somewhere else?

The midpoint of the interval, see answer to next question.

(2) Construct an analytic proof, starting from Galileo's laws of motion, showing that the average velocity during a time interval is the same as the instantaneous velocity at the midpoint of the time interval.

Non-calculus proof:

a = (v_e - v_o)/t = (v_m - v)/(t/2)

Then: (v_e - v_o)/2 = v_m = <v>

(3) [Simanek, 1st edition. Dropped because most students can't figure it out.] What would be wrong with using the method of differences here? Show, by an appropriately chosen algebraic example, what would happen if you did this.

Let the successive velocities be labeled by the letters A through I, and the time interval be one unit. Then the method of differences gives the acceleration as:

(F-E-B+A) + (G-F-C+B) + (H-G-D+C) + (I-H-E+D) = A - 2E + I

Only the end and middle points are used.

(4) [Simanek, 1st edition. Dropped because it's difficult to read and compare the answers students give.] Suppose that at about the middle of your spark record an unknown number of spark marks were missing. How could the experimental data be "salvaged" so all of the remaining data on both sides of the "break" could be used in the calculations. How much would this affect the accuracy of the results? (Say something more meaningful than "not much.") Discuss this for each of the methods of analysis.

The two parts of the tape could be analyzed separately, and the two accelerations then averaged. The accuracy would depend approximately on the total number of points used just as for a single complete tape.

The velocity time graph will have a "break" at the missing points. Fit a straight line to each complete portion, using the same slope for both. The size of the discontinuity will indicate the number of missing points. That is, displacing the upper portion of the graph along the time axis will bring both portions of the fitted line into alignment. The amount of the shift indicates missing time.

Quantitative conclusions about error in this example may be too much to expect from a non-calculus class.

GRADING CHECKLIST

1. Clear statement that the acceleration was found to be constant over the run of 1.5 meters.

2. Reasonable value of g.

3. Reasonable uncertainty estimate for g, with supporting method.

4. Questions, total three points.

5. Graph

© 1998, 2004 by Donald E. Simanek.