M-6 HOOKE'S LAW

1. PURPOSE

To verify Hooke's law for a spring and to determine the spring's elastic constant by static deformation.

To investigate the vibratory motion of a mass on a spring and to determine the elastic constant from the period of this motion.

2. APPARATUS

Laboratory stand, tapered springs of various types (brass, iron, steel), weight hanger, weight sets, stopwatch, metric scale.

The maximum loading of the tapered springs is:

Iron or Steel: 1.5 kg
Brass: 800 gm

3. THEORY

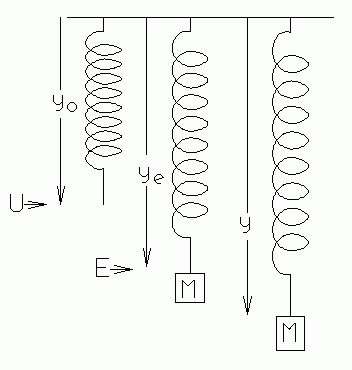Fig. 1. Analysis of a susppended spring-mass system.

Hooke's law can be written:

[1]

F_s = -ks

where F_s is the tension in a stretched spring and s is the spring's displacement from its unstretched position. k is the elastic constant, or "spring constant."

In the figure, the force F_s of the spring acts upward on the suspended mass, m. The downward force on the mass is mg. The net force on the mass is therefore F_net = F_s - mg, in all cases.

The position of the end of the spring is y, measured from an arbitrary reference position. In the diagram the reference is the upper support, though the reference height could be taken at any position.

Since the reference point for the tail of the y vector is arbitrary, so is the reference for the head of y. We take it to the end of the spring, that being a convenient point to locate in the diagram.

The diagram shows three positions of the spring. The "unloaded" position U is the position when no weight hangs from it. The equilibrium position E is when there's mass M on the end of the spring, suspended in equilibrium. The third position is a "snapshot" of the mass while it is moving up and down with oscillatory motion around the position E.

Since Hooke's law is linear, then if we add weight W to the hanger,

[2]

W = -kΔy, or in this case, W = - k(y - y_o) , for any y.

W is the weight of the mass added to the hanger. For the equilibrium position, we have,

[3]

W = -kΔy = -k(y_e - y_o) = Mg

When a mass M is displaced from the equilibrium position, and released, it undergoes oscillatory motion. Here M must be the total mass (including hanger). At any displacement y we have:

[4]

W - Mg = Ma , or -k(y - y ) - Mg = Ma

[5]

But from (3), -ky + ky_o + ky_e - ky_o = Ma

[6]

and therefore, -ky + ky_e = Ma

[7]

so, -k(y - y_e) = Ma

or, a = -(k/M)(y - y_e)

This is the defining equation for simple harmonic motion: "The acceleration of the mass is proportional to its displacement from the equilibrium position and in the opposite direction to the displacement." So we have proven that a mass hanging from a spring which obey's Hooke's law will move with simple harmonic motion when displaced from the equilibrium position.

By solution of the differential equation (7) one may derive the period of the simple harmonic motion of the mass-spring combination:

[8]

T = 2π√(m/k)

Since F = ma, and from Hooke's law, F = -kx, then ma = -kx. Therefore, m/k = - x/a, and

[9]

T = 2π√(-x/a)

3. PROCEDURE

Fig. 2. Apparatus arrangement. Note that the large diameter end of the string is at the bottom. [CENCO]

(1) Suspend the spring from the ring stand. If the spring is of the "tapered" kind, be sure that the larger diameter end is down. Hang a weight hanger from the spring, and take data on suspended mass vs. extension. Do not exceed the stated limits of the spring. For the steel spring do not exceed 1.5 kg, for the brass spring do not exceed 800 gm. If in doubt, consult your instructor. Plot this data and determine the spring constant from the slope of the straight line.

(2) Using the same spring, take data on the period of simple harmonic motion of a spring mass combination. Do this for a variety of values of mass. Keep the amplitudes small. Make an appropriate plot relating period to mass.

You may find that the graph of period vs. mass shows an unexpected relation. Eq. 8 does not take into account the mass of the spring itself, which is also a moving part of the system. However, since each part of the spring moves with a different amplitude, we cannot simply add the spring's mass to the mass of the load. The period of the system, with spring included is:

[10]

T = 2p[m + m_e/k]^1/2

where m_e is the "effective" mass of the spring. Find the spring's effective mass from your graph, and express it in the form: m_e = ( )m_s where m_s is the mass of the spring. That is, find the constant ratio between effective mass and actual mass of the spring.

(3) As time permits, repeat the above with a spring made of a different material.

(4) Rather than suspend the mass, one can do this analysis using an air track, with a glider as the mass, and two springs attached to either end of the glider. Lighter springs, of smaller spring constant are best.

4. QUESTIONS

(1) The tapered springs obey Hooke's over the widest range of loads when suspended with the large diameter end down. This is why they are made that way. Explain why this works.

(2) Some springs are wound with the wire under torsion, so that they are naturally tightly compressed. The plot of extension vs. applied force will show that the intercept on the extension axis is negative, that is, the "unstretched length" is shorter than the actual unloaded length. Explain.

(3) Does your data show that the weight of the spring itself is important? How can you properly include the spring's weight in your analysis? If you have had calculus, you can do this exactly, finding the formula for the "equivalent" mass of the spring. If you haven't had calculus, you can still find the equivalent mass from your data. In either case, you'll have to know the total mass of the spring, and its unstretched length.

(4) Find out from library research, or by direct derivation (calculus) the relation between a spring's effective and actual mass. Is this the same for a static measurement of k as it is for a dynamic measurement?