Secrets your physics textbook didn't reveal.

Is the path of a trajectory a parabola or an ellipse?

Many physics textbooks tell you, in the early chapters, that the path of a trajectory is a parabola. Indeed, all of the mathematical formulae and calculations dealing with trajectors of objects falling, thrown or propelled support that interpretation. But in later chapters, when earth satellites and ballistic missiles are discussed, the textbook may reveal that their orbits are portions of ellipses. Later, when the orbits of planets and comets are discussed, they are all elliptical, or, in the case of non-periodic comets, hyperbolic.

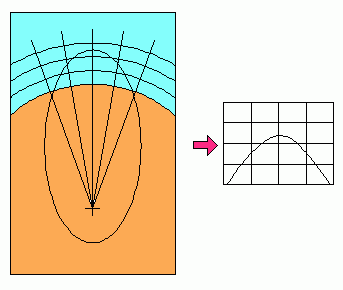Transforming an ellipse to a parabola.

So what's going on here? What is the mathematical shape of a trajectory?

It depends on your coordinate system. When dealing with trajectories of short range and near the earth's surface, we use a coordinate system in which one axis is "horizontal" and one axis is "vertical". We treat it as a cartesian coordinate system. When we take position data on a projectile and plot it on cartesian graph paper, we get a parabola. End of story?

Not quite. Two "vertical" lines aren't exactly parallel. They diverge from the center of the earth. Also, "horizontal" surfaces aren't flat, they are portions of spheres. Also, the equations of the ballistic parabola are based on the assumption that the acceleration due to gravity, g, is constant. In fact, g decreases with height.

If we were to look at the trajectory from a long way off in a coordinate system anchored on the earth's center, we would see that the trajectory is actually a portion of an ellipse, with one focus of the ellipse at the earth's center, as shown in the left figure. Here we have sketched in some radial lines, and some horizontal surfaces. Clearly polar coordinate paper would be appropriate for plotting this curve. But when we plot it on cartesian paper, as shown on the right, the curve transforms to a parabola.

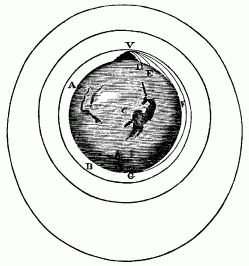Newton's mountain, 1729.

All ballistic trajectories of this sort are ellipses, including earth-satellite orbits. [A circle is just a special case of an ellipse.] One of the first to recognize this was Sir Isaac Newton, who included this sketch in his Principia (1729). It shows a cannon firing horizontally from a high mountain. With low velocity, the cannonball strikes the earth. With higher velocity, it lands farther from the mountain. With sufficient velocity, it could "fall" continually around the earth in a circular orbit. The moon may be thought of as falling continually toward the earth, in a near-circular orbit. This was Newton's great insight, which showed that the moon and the cannonball obey the same laws of physics, as do all the planets and their satellites, and everything else in the universe.

Of course, no mountain is this high relative to the earth. And no cannon in Newton's day could have achieved sufficient velocity to put a cannonball into orbit. Newton's "mountain" was a clever visual illustration of an important concept about orbits and trajectories. Yet, it is remarkable, that when we finally achieved the technology to launch a man-made earth satellite, we did it exactly this way (but without the mountain). A booster rocket carried the satellite to an altitude of several hundred miles, by firing straight up to get through the densest part of the atmospher most quickly, then leveling off and releasing the satellite from the booster while moving nearly horizontally.

NASA does it without the mountain.

If we choose to pretend that all verticals are parallel, and all "level" surfaces are planes and are parallel, then we distort the real situation, converting ellipses into parabolas.

There are other factors that cause real trajectories to depart from these idealized paths. The friction of the air (air drag) continually slows a projectile, making its orbit somewhat asymmetric. If we choose a coordinate system that is fixed on the earth's surface, then the path, in this coordinate system, will depart in important ways from the idealized path. This is because the earth itself is rotating on its axis, and our coordinate system fixed on the earth is a non-inertial coodinate system. The effects of earth's rotation on the trajectory include centripetal and Coriolis effects, which are very important in atmospheric and oceanographic studies, as well as military applications of long range cannons and ballistic missiles.

For short distances, near the earth, the small portion of path we observe can be equally well approximated as an ellipse or a parabola, and we don't even "see" the results of coriolis and centripetal effects. In fact, for shallow trajectories (launched at small angles to the ground), the trajectory is approximately a circular arc. This is the case for rifle bullets, baseballs, and golf balls. If you take a small enough portion of any curve, it approximates a circular arc.

But the projectiles' paths, expressed in an inertial (non-accelerating) coordinate system anchored on the earth's center, are approximately ellipses. Any textbook or exam that asks you "What is the mathematical shape of a projectile's path?" is asking an unfair question, especially if it's multiple-choice.

The question gets even more murky if you consider the path as seen in a coordinate system anchored on the sun. Then the path of an earth satellite is a "loop-the-loop" spiral path of considerable complexity. However, since the moon takes a whole month to orbit the earth, its path (in the sun's coordinate system) is a rather smoothly undulating wavy curve that never crosses itself and (surprise) is always concave toward the sun. Here's another case were some textbooks draw it incorrectly. Try drawing it yourself, to scale. You'd better have a very large sheet of drawing paper to clearly see the undulations on the moon's path.

There are several "morals" here:

• When using the word "is" be aware of the logical difficulties associated with "verbs of being".
• When specifying the shape of the orbit of a moving body, always specify "with respect to what?".
• Transforming curves from one coordinate system to another can seriously alter their apparent shape, and even change their names.
• Textbook pictures are often schematic only, not to scale, and therefore potentially misleading.

References:

1. Ballistic Trajectory: parabola, ellipse, or what? by Lior Burko and Richard Price. Thorough discussion, with the math.
2. Reduced English discusses the dangers of "verbs of being" and advocates throwing them out entirely.

Top of this page
Physics documents.
To Donald Simanek's front page