Nature's Impossibilities.

The difficult we do right away. The impossible takes a little longer. —Engineer's joke.

It's only impossible if you stop to think about it. —The Pirates! Band of Misfits (2012 movie).

The inventive spirit never dies.

Unlimited optimism.

They dislike any suggestion that particular things might be impossible, especially particular things they desire very strongly, such as perpetual motion machines and over-unity machines. Sometimes this is expressed as an almost religious conviction that if mankind needs something very badly, then somehow, someday, some clever person will find a way to accomplish it. It is a "faith in the possibility of unlimited progress". So, we are running out of fossil fuels? Not to worry, someone will find a substitute fuel in great abundance. Is our population growth threatening to exceed the resources of earth? No problem. By the time that gets dicey, we will be colonizing the planets, and eating delicious synthetic food made from industrial waste. This sort of thinking has (apparently) always worked for us in the past, so surely, some think, such progress will continue forever.

I submit that this excessive faith in science stems from a profound misunderstanding of science. It is a selective reading of history, concentrating on past instances of success, without examining why those successes were possible. Also, people love stories (often only partly true) of cases where pessimistic predictions were found to be wrong. Very often these pessimistic predictions were casual hunches and not carefully thought out analyses, and some of these stories are simply urban legends. The "bumblebees cannot fly" legend is one such case. [1] Such negative predictions, especially when made by prominent persons of history, make entertaining reading, and I have a collection of them on my website, titled It'll Never Work. Equally entertaining are past optimistic and fantastic predictions of future developments that haven't come about, and never will. Several published books have mined those as cautionary examples of the dangers of overconfidence in science and technology. [2]

The chimera of over-unity devices.

One might have thought people would give up this effort once scientists formulated and then understood the laws of thermodynamics, which tell us that energy is strictly conserved in any mechanical device, whatever its detailed construction, whether it be strictly mechanical, or electrical or magnetic, or whatever else you might conceive.

How can we know what's impossible?

So, the perpetual motionists forged on, tinkering and fiddling with wheels, gears, magnets and fluids, each hoping to be the first to stumble on the secret of unlimited energy. "It must be possible," they said, "because mankind desperately needs it." Each year patents are issued for devices that any competent physicist would find laughable. The Internet has many websites and forums describing incredible devices, along with claims of the potential of achieving incredible results—with a little more refinement and tinkering. These accounts are frustratingly short of details and experimental results. Indeed, they sometimes describe a machine as "working" that hasn't yet been built, even in prototype.

Where's the secret hiding?

Others pin their hopes on certain notions currently popular in speculative theoretical physics, such as "dark energy", "dark matter", and "zero point energy". After all, they say, these are "another form of energy" and we know that even matter can be converted to energy. This new energy must be there, free for the taking. These folks propose, and some even build, machines that are superficially indistinguishable from previous attempts to get energy from nothing, only these folks claim that the energy does not come "from nothing" but from energy that's invisible and all around us.

Perpetual motionist announcing a breakthrough.

Personally, I think those who seek to make such "free energy" machines are destined to fail. But my reasons aren't easy to explain. Since I have been a physicist my entire career, I have a different perspective on these questions than non-physicists do. I am well aware that science is not yet complete, and that present-day scientific laws and theories will surely be modified and improved in the future. We don't know everything yet, and probably never will, but it doesn't follow that anything we might imagine "could be true". Perpetual motion believers hope that there's a flaw or loophole in our understanding just such as to allow clever inventors to exploit a "sea of energy" that they imagine must pervade the entire universe. The catch is that (1) that "sea" of zero point energy the physicists postulate is locally very dilute, and only tiny amounts of this energy would be within the "grasp" of such a machine, and (2) nature has certain "gotchas" in its laws that provide no way to extract even that energy to produce useful work. In fact, the laws governing it specifically prevent this. Finally, even if we are wrong about those "gotchas", present scientific models give us no clue how to get around them, so inventors are like blind men fumbling around in the dark, hoping that something might magically work if they tinker with it long enough. Along the way they are encouraged when they see some result that was unexpected (to them), and seemingly not in accordance with their (limited) knowledge of physics. They proclaim such observations as "breakthroughs".

In their enthusiasm, the inventors seem to think that "environmental" energy comes completely free. Every such energy source we know of requires expenditure of energy to extract it from nature. Extraction methods aren't perfectly efficient, and no one knows how to make a perfectly efficient system of any kind. If they did, that system itself would be a perpetual motion machine, even if it didn't have over-unity performance. It would turn forever without producing any useful work.

Another thing that perpetual motionists overlook is that the physicists' "zero point energy" is a "phantom" energy, a mathematical fiction that is part of the mathematical theory of quantum mechanics, and is not necessarily equivalent or convertible to what we call "real" energy (of a kind that can be converted to useful work). These "energies" are something of the character of the older concept of "electric field lines" that are shown in textbook pictures, depicting how charges act upon other charges, or the "gravitational field lines" from masses. Physicists know that field lines are not "real" entities in space, but only a convenient mathematical concept to help us visualize what's going on and predict what would happen if a charge or mass were placed at a certain place. No one would think that we could reach into "empty" space, grab a handful of field lines, and put them in a box.

Even people who do not for a moment accept the possibility of perpetual motion sometimes fall into this philosophical trap. They say, "Perpetual motion can't work because you can't create energy from nothing." That is an empty argument. They are thinking of energy as "stuff" or a "substance", then implicitly assuming that stuff is indestructible and cannot be manufactured from something else." But energy isn't material stuff like matter. Energy is a convenient mathematical concept for doing the "bookkeeping" as we observe how things interact and influence each other. Energy is an "accounting" scheme for describing the behavior of physical things.

Mesmerizing magnets.

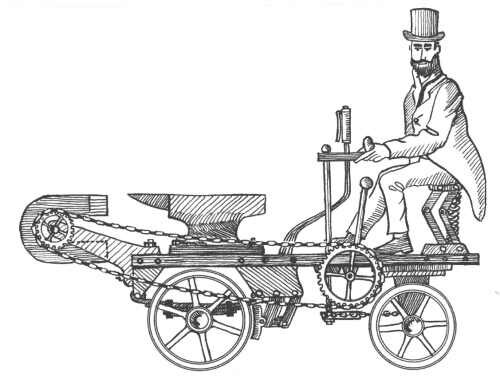Hans-Peter Gramatke's magnet car requires no fuel at all. One little problem, though. It can't go in reverse.

Why does the behavior of magnets fascinate so many people? Probably because magnets are not part of our daily experience, and in certain situations behave in ways that are surprising (and seem "magical") when first observed. Those who do laboratory physics and electrical engineering have become familiar with the behavior of magnets, and know the laws of their behavior. Making predictions with those laws can be complex. When magnets interact and move relative to each other, the shape of the magnetic field around each one changes. Still, the bottom line is this: we know very well the laws they obey, and no experiment we've ever done with magnets has shown the laws to be violated. Furthermore, the laws can be shown, mathematically, to be fully consistent with strict conservation of energy and momentum—with no exceptions. A vast amount of experimental evidence confirms that. If there were flaws in these laws, that fact would surely have shown up in the functioning of one or more of the electromagnetic machines (motors, generators, etc.) that keep our industrial technology humming along. So the bottom line is that those who hope for a magnetic perpetual motion machine are doing so because they do not yet understand the physics and behavior of magnets.

How can we be certain nature is always lawful?

But there are philosophical questions. These have to do with the concept of nature's lawfulness. Does nature operate according to strict and regular laws (even if we do not yet know all of those laws)? If nature is perfectly lawful, we can predict its behavior in particular situations, although not perfectly (since our measurements are never perfect). Or does nature sometimes do something "capricious" and unpredictable? In that case, our predictions might be far off the mark, and the event would qualify in some people's minds as a "miracle".

In physics, when something like an unexpected scientific observation turns up, more careful examination eventually shows that our predictions were mistaken. Either we blundered in the calculation, or we overlooked a subtle condition or variable. But in a few much-publicized cases, some previously accepted law was at fault, and the observation tells us something new that may lead to refinement of a that law. Then we realize that nature was behaving lawfully after all, we just hadn't gotten the law completely right. No such case, in the whole history of science, has convinced us that nature has ever, not even once, behaved in a capricious manner. So we conclude, at least as a working hypothesis, that "nature is strictly lawful" and it is our job to keep trying to express those laws better and better. Actually we are trying to construct mathematical laws that fully describe nature in all cases. But we aren't so arrogant as to assume that these laws we invent are "nature's laws". We know, from past examples, that two different sets of laws may describe nature equally well, and to say that one set is "better" or "truer" than the other is saying too much. So the laws we formulate to describe nature may change, but nature's lawful behavior continues unchanged.

Lest my meaning be misinterpreted, I am talking about basic laws here: laws like Newton's laws of mechanics, Maxwell's laws of electrodynamics, the laws of thermodynamics, etc. Sometimes, things unexpected to us occur in complex situations, without violating any basic laws. It may rain when no rain was predicted. These are situations where outcomes depend on the interaction of so many basic laws of nature in combination that we can't possibly know the relevant variables well enough to make precise and fully reliable predictions, or we don't have the computing power to process the data.

Even in complex situations as this there are some physics laws that can be tested, after the fact, and shown to have been fully obeyed. No matter what the weather does tomorrow or next week or next year, it will never violate the laws of thermodynamics, the conservation of energy, the conservation of momentum, the conservation of angular momentum, etc. There is a class of physical laws, called "conservation laws" that allow us to make definite, measurable predictions about entire complex systems without knowing everything that's going on in the system. Physicists like such laws, because of their robust character. They allow us to make correct predictions even though we have some ignorance of the system. And, so far, nature has not let us down.

These conservation laws are not laid down by scientists as dogma by fiat. Nature has imposed them upon us, and we express them in words and mathematics because they are so useful. Such laws are inferred (generalized) from the whole history of our observation of what nature does, and from experiments done in laboratories around the world (and by observations made by astronomers in the larger laboratory of the universe). They have been tested not only experimentally, but also by forging the mathematical links between them and the other fundamental and well tested laws in all fields of science, to convince ourselves that they constitute a logically consistent and comprehensive framework for describing nature.

That's why we confidently say, "No one will ever make a machine that outputs more useful work than the amount of energy input to it." Such a machine would violate conservation of energy, and we can show mathematically that it would also seriously violate Newton's law F = ma, Newton's third law, and therefore also violate conservation of momentum. These violations would not be small and easily overlooked. They would be large and serious violations, easily observed. Yet no such violations of these laws have ever been observed in the history of science.

Universality of physical laws.

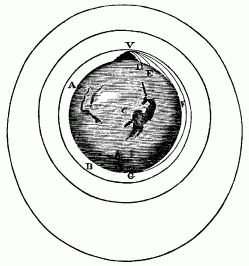Newton's diagram of the relation between earthbound cannonball trajectories and celestial orbital motion.

We must mention another important point, one that is seldom discussed in modern textbooks. That is the universality of physical laws. In the early history of science laws were assumed to have applicability only to limited areas of experience. A law about water was not necessarily assumed to also apply to some other liquid. Laws that were true on earth did not apply to the sun, moon, and stars. In fact, Aristotelian physics declared that the laws of the terrestrial realm (on earth) did not apply in the celestial realm (the heavens). This view was held till the time of Newton. Newton is credited with unifying the laws of motion of the moon and planets with the mechanical laws of motion on earth. His famous diagram relating the motion of a hypothetical cannonball with the orbit of the moon nicely illustrates this. Newton's law of gravitational attraction accomplished this unification, and is called "Newton's Universal Law of Gravitation", because it is assumed to apply everywhere in the universe and at every time in history or in the future. That is, the very same equations apply on earth as in the heavens, and in the past and future. Now when put that way, that's a rather audacious claim, for we haven't yet tested the equations everywhere and at every time. Of course we are testing and confirming them every day, as scientists observe and measure motions of everything they observe in the heavens, and as NASA computes orbits of spacecraft and space probes and puts the results to the test with every space vehicle that is sent from earth.

Science continually tests the universality of laws, as well as the logical unity of laws. So far the laws have met the tests with flying colors. Of course unexpected facts of nature continually turn up, and some of these were not anticipated by the old laws as they were previously formulated. For example, research on atomic physics taught us that the law of conservation of energy needed to be amended to the conservation of mass-energy. And later, relativity showed that momentum and energy are part of an energy-momentum four dimensional vector—which is a conserved quantity in a closed system. So our physics at any given time may not yet have the universal laws of nature formulated perfectly, but as we discover new facts and devise new laws and modify the old laws and theories, it still appears that nature itself does operate with universal lawfulness and regularity. We just haven't yet learned all the details.

Every advance in physics has confirmed that "Nature abhors macroscopic perpetual motion," and that "no device can put out more useful macroscopic work than its energy input".

None of this gives any encouragement to perpetual motion enthusiasts. Some may still hope that there's some place or special situation in the universe where over-unity machines are possible. Perhaps over-unity machines only work on Tuesdays at 3:15 PM at a particular spot on Mars. Of course that's an absurd suggestion. But, seriously, if a particular one of our physical laws is violated at some particular place and time, how do we interface a machine operating there with the rest of the universe where the ordinary laws are scrupulously obeyed? You can hypothesize all sorts of fantastic ways to get around this, but there's no hard evidence supporting any of them, and not a clue how to implement them. [3]

Still, the perpetual optimist asks, "How can scientists be so sure of the validity of laws that they claim apply to things that haven't yet been seen?" It's a reasonable question. Of course part of the answer is that we can't be absolutely certain of any particular law's applicability to any particular, as yet unobserved, device or process. No law is claimed to be perfect. But some are so thoroughly tested and precisely confirmed in so many diverse situations that it would be foolhardy to bet against their being universally valid. Also, we must distinguish between well-established and well-tested laws, and other, less well-validated ones. We must also note that some laws are so thoroughly integrated into the logical/mathematical scheme of other laws that the likelihood of all of them being seriously in error is virtually nil. Underlying all of this is, of course, the confidence that nature, though sometimes subtle, will not play games with us by changing the rules just when we think we've figured them out.

Some things really are impossible.

The unified web
of physics laws
and theories.

Some also have the mindset that "Anything is possible if you are clever enough and tinker with it long enough". If we took that idea seriously, we'd have to conclude that any law of nature could be broken or circumvented. Some go farther, and, with religious conviction, say, "If you believe in something, you can make it happen". This is "magical" thinking of the sort held by the ancient alchemists. Do these people really suppose that future technology will allow someone to jump over the Moon with an unpowered pogo stick? Or that medical science might produce a human who could run a one-minute mile? Or perhaps allow someone to walk through a brick wall without evidence of damage to himself or to the wall? Perhaps they also believe it will someday be possible to teleport a human being anywhere in the universe, or jump backward in time. Well, maybe perpetual motion machines could be used to power these innovations.

Seriously, we must admit that some things are clearly impossible in nature, though we don't always know which ones they are. Some are logically impossible, and are of little interest as scientific examples. Here are a few of that sort: It's impossible to make a four-sided triangle. It's impossible to make a triangle in a plane with equal sides and unequal angles. You can't make a perfect circle in a plane with a circumference equal to 8 times its radius. Are these physical impossibilities, or are they logical impossibilities? If our universe is structured on Euclidean geometry, they are physical impossibilities. And from what we have learned from all available evidence, the universe is, very nearly, Euclidean, and certainly is in our local region of it.

So which laws are fundamental?

From Newton's laws the conservation laws are derived, then the thermodynamics laws. But in fact one could begin with the conservation and thermodynamics laws and derive Newton's laws from them. So which laws are the "fundamental" ones?

We found, perhaps to our surprise, that some of the classical laws still hold in modern physics. The conservation laws and the thermodynamics laws are still valid in modern physics, while Newton's laws are modified. This shouldn't be surprising, for Newton worked within a world-view that supposed influences like gravity were instantaneous over great distances, and no one suspected that the speed of light was finite. Now that we know that the speed of light is finite, and the speeds of particles with nonzero rest mass cannot even go that fast, and also that electric and magnetic fields (and presumably gravitational fields as well) travel at the finite universal speed of light. [4]

So this suggests that conservation and thermodynamics laws are more "universal" than we previously thought, and that they are the present best candidates for fundamental and universal laws, from which other laws can be derived.

Geometry is fundamental.

The importance of geometry in physics could justify a separate essay. The conservation laws of energy, momentum and angular momentum are perhaps the most fundamental physics laws we have. And where do they arise? They are based on the underlying geometry of the universe. The German mathematician Amalie Emmy Noether (1882–1935) showed the relation between geometry and conservation laws in 1915 (published in 1918). Noether's theorem is recognized as the foundation of the conservation laws of physics. While the various versions of this theorem are highly mathematical, the essence of them is this: If a physical process obeys laws that are invariant (constant) over time, then the energy of this process is conserved. If a process obeys laws that are invariant under spatial transformation, then its momentum is conserved. If a process obeys laws that are invariant under rotation, then its angular momentum is conserved. This is seldom mentioned in popular treatments of perpetual motion or even in elementary textbooks. Once its significance is appreciated, it makes the efforts of perpetual motionists seem pathetically misguided. They are playing around with wheels, gears, pulleys, magnets and electrical devices, all of which operate within the strict laws of physics imposed by geometry, and therefore cannot achieve what the inventors hope for. Now if these folks could figure a way to make something move continually around a closed path downhill all the way—in either direction—they might be onto something. They would have modified the geometry of space, which would open up wonderful possibilities for new physics. But no one has a clue how to do that, and no hint that it's even possible.

You can read more about Noether's Theorem at these sites:

• Noether's Theorem from the Wikipedia.
• John Baez's briefer account of Noether's Theorem.

What about relativity and quantum mechanics?

For many years I've been getting perpetual machine proposals via email, but I've never gotten even one that appeals to relativity or quantum mechanics as its operating principle. None are accompanied by any analysis that uses vectors, conservation of momentum, or calculus. This is a good indication of the technical level of my audience.

I do sometimes get questions such as "Does conservation of energy still apply in relativity?" The simple answer is "Yes, but the definition of energy is broadened." I recommend Is Energy Conserved in General Relativity? by Michael Weiss and John Baez. The bottom line is that, even when relativity is taken into account properly, no device can put out more useful work than the amount of energy it takes in. Isn't nature perverse?

Many readers could benefit from a good review of conservation laws. See:

Conservation Laws, Answers.com.

Conservation Laws, NationMaster Encyclopedia.

For a good history of quantum mechanics see The Strange World of Quantum Mechanics by Dan Styer, Oberlin College Physics Department.

Some people, reading about the Heisenberg uncertainty principle, suppose that it might be used to violate energy conservation on a very small (quantum) scale, then find some way to make this happen simultaneously in all particles in a huge sample, accumulating a large enough total energy to be useful. The trick is to make all this happen simultaneously in the quantum world where time also has quantum uncertainty. However, there are some interesting discussions on the web:

Losing energy in classical, relativistic and quantum mechanics by David Atkinson.

Is energy conserved in quantum mechanics? Answers.com.

I get some proposals of thought experiments that claim to show that (a) classical physics is wrong in some situations, and (b) relativity and quantum mechanics allow for perpetual motion. Invariably these make common errors: (a) using "common sense" non-rigorous arguments, (b) using classical physics for a situation that demands a relativistic or quantum mechanical analysis. Common sense is simply inadequate for dealing with physics that isn't part of our everyday experience, and such arguments aren't worth the trouble of reading. We've known for more than a century that classical physics is inadequate for certain situations, which is why quantum mechanics and relativity were found to be necessary. I could give many examples, and don't need any more to illustrate that point.

Endnotes.

This popped up on an internet discussion group back in 1999, with this interesting response.

Subject: FW: Bumblebee Flight
Date: Wed, 13 Oct 1999 12:47:46 -0700
 
A long time ago [1989] I wrote an article for the journal American
Scientist entitled: "The Flight of the Bumblebee and Related Myths of
Entomological Engineering" (Am. Sci., Vol. 77, pp. 164-8).  In this I
gave what still appears to be a correct account of the "Didn't the
aerodynamicist prove that the bumblebee can't fly? [sarcastic ha ha]"
story.  I too had tried to find the name of "The aerodynamicist" who
did this to us.  After a long search I was told by a very reputable
source that he thought that individual (who was badly misrepresented
subsequently by the "press") was the Swiss gas dynamicist Jacob
Ackeret - a famous name in supersonic aerodynamics. It was about the
right vintage, so I wrote that in my article without naming Ackeret
explicitly. Following publication, however, I got mail. Boy did I
get mail - including half a dozen Xerox copies of portions of the text
of the book Le Vol Des Insects (Hermann and Cle, Paris, 1934) by the
famous entomologist August Magnan. On page 8 of the introduction, one
finds:
 
    "Tout d'abord pouss'e par ce qui fait en aviation, j'ai applique' aux
    insectes les lois de la resistance del'air, et je suis arrive' avec
    M. SAINTE-LAGUE a cette conclusion que leur vol es impossible."
 
Thus the culprit is finally named: Sainte-Lague, Magnan's lab
assistant who was apparently some sort of engineer. 
 
Share and enjoy.

John McMasters
Technical fellow
The Boeing company
Seattle, Washington

The error seems to be that the analysis compared the bee to a fixed-wing aircraft, without a proper dynamical analysis of the rapidly moving wings.

[2] Books that treat the theme of over-optimism about science and technology include: "Wasn't the Future Wonderful?" by Tim Onosko (1979), "Out of Time" (2000) by N. Brosterman, "Where's My Space Age? The Rise and Fall of Futuristic Design" (2003) by Sean Topham and "Follies of Science. 20th Century visions of Our Fantastic Future", by Eric Dregni and Jonathan Dregni (Speckpres, 2006). The last of these is nicely illustrated, but has superficial text and too many errors to suit me.

[3] There really should be a clear demarcation between three kinds of talk about physics, theoretical, experimental and speculative. Of course these are interactive and cannot exist independently. Theoretical physics deals with the logical structure of laws and theories, expressed mathematically. Experimental physics is the realm of experimental discovery and testing. Speculative science is the one that seems to get the most media attention these days. It is more than mere hypothesizing, but it is the construction of elaborate speculative scenarios that attempt to fit known data and known laws and theories into a new and hopefully more revealing form. Some critics suggest that this is more philosophy than physics, and not to be taken seriously as hard science. In speculative physics, new concepts may be introduced, such as strings, superstrings, dark matter, dark energy, parallel universes, etc. These are not supposed to be "real", but are "virtual" or "conceptual" concepts that facilitate the mathematics. One current speculation is that fundamental laws and even fundamental "constants" of physics may vary in space and in time. This allows that these things may not be as "universal" as we previously supposed. At the present time, this speculation is not anywhere near being confirmed and accepted physics. One may ask "Is there evidence supporting it?" Of course, for speculative physics is designed to account for all known evidence. It must, if it is to be taken at all seriously. "Does the fact that evidence supports it also confirm it?" No, for the same evidence equally well supports other speculative theories, though evidence very well might come along later that would discredit one or another speculations. Only time (and more evidence) will determine which of the many speculative scenarios becomes (tentatively) accepted physics. But none of those I've seen gives any encouragement to hopeful seekers of perpetual motion and over-unity machines. And none of the speculative theories proposed by these perpetual motion "seekers" accounts for all of the known evidence well enough to be taken seriously by knowledgeable scientists.

[4] Elementary textbooks often fail to reveal that classical Newtonian mechanics has an unstated fundamental assumption that when bodies influence each other by forces "acting at a distance" these influences act instantaneously. Thus there is no time delay due to separation distance, no matter how great the distance. It is curious how few students notice this, or suggest that it seems absurd based on "common sense". They have already learned that common sense is not a reliable guide to physics. In the early 20^th century experimentalists were learning that no force influence could travel faster than the speed of light, a speed that was constant, the same for all observers. Still, many students forget this, thinking that relativity only limits the speed of objects that have nonzero rest mass.

Students should have suspected this when they learned about Newton's third law: "If body A exerts a force on body B, then B exerts and equal sized and oppositely directed force on A." Can this really be true always? What if A and B were light years apart? If the gravitational influence can't travel faster than light, any change in position of A relative to B would result in a change of its force on B, but B wouldn't "get the message" for several years. So, during the transit time, Newton's third law would be violated. But if the propagation of the gravitational impulse were instantaneous, Newton's third law would be valid. Once we realized that fields do not propagate instantaneously, we had a problem. This is just one of the many reasons why we know that Newtonian classical mechanics was in need of adjustment.

The modified equations of relativity reduce to the equations of classical mechanics when we allow the limiting velocity for all things, c, (the speed of light) to go to infinity and force field influences to propagate instantaneously. This is often called the "classical limit" test of a relativistic equation. Usually textbooks express it differently: as "v/c goes to zero" where v is the relative speed of two observers. This obscures the important point about field propagation. Obviously in the limit when c approaches infinity, v/c approaches zero.

This document is evolving. Suggestions are welcome for other points I ought to discuss. Contact me at the email address to the right. This revision: October 2008.

© 2008 by Donald E. Simanek.

Return to front page.
Return to the top of this document.
Return to The Museum's Main Gallery.