The Roberval Balance.

Steelyard. Single-pan balance.	Double-pan laboratory balance.	Roberval balance. Double-pan balance.

Laboratory double beam pan balance with Roberval linkage hidden.

Though many students have never heard the name "Roberval balance", most have used one. The standard laboratory double-pan balance has a Roberval construction that keeps the pans level as they move up and down. More importantly, it prevents the pans from tilting as they move up and down, and this is the principle that ensures that the position of weights on the pans has no effect on the balance.

This balance mechanism was invented by the French mathematician Gilles Personne de Roberval (1602–1675). It may not seem remarkable, but compare it to the simple lever. The position of the weights on a lever (balance-bar) relative to the fulcurm is what determines its balance. Many scales used for weighing have one such bar with a sliding weight. (Even the laboratory double-pan balance incorporates such a sliding weight for fine adjustment.) The balance of a simple lever depends on the distance of the weights from the fulcrum. The balance of a Roberval system does not depend on the distance of the weights from its center of rotation.

Do students really understand the various forms of balance? I doubt it. Illustrated above are several kinds, each operating on a different principle. The steelyard was a direct and simple application of Archmedes' law of the lever. Changing the position of the sliding weight changes the torque on one side. The double-pan balance has pans that usually have the same lever arm. In either of these scales, the position of weights on the pans doesn't matter, for each pan moves to find an equilibrium position so that the center of mass of pan and weight is directly under the suspension point.

Surprising demo with the Roberval balance.

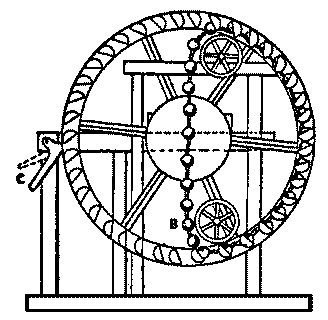
The Roberval balance is a compound lever system. Students who try to treat it as nothing more than a simple lever get into serious trouble. Consider the example shown to the left. The red weight D is on the right pan. The green weights A, B, and C are fastened or glued together and to the pan so that some of the weight is on the right of the central pivot of the balance. No matter. So long as A + B + C = D, the system is in balance.

The Roberval balance achieves non-rotation of the pans by use of a parellogram linkage of levers. This is a special case of the "pantograph" lever system used for copying drawings to larger or smaller size. Other systems may be used to achieve the same result, for example, gears or pulleys.

When Roberval invented this balance, many persons, including mechanics, had only superficial understanding of mechanical principles. The demo illustrated here might have surprised them, for they simplistically thought that balance was determined solely on weight and distance from the rotation axis of the scales. Many perpetual motion machine inventors still suppose that if there is "more weight on one one side" of a system's rotation axis, then the system can not be in equilibrium, and therefore must rotate. This simple demonstration clearly shows that is not so.

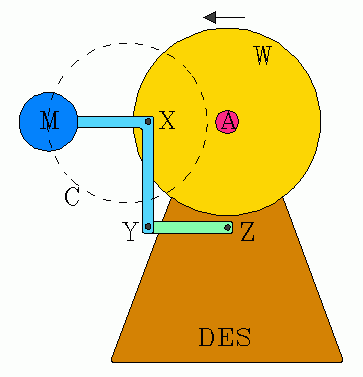Overbalanced chain. Doesn't move.	Very overbalanced system.

These two examples should convince anyone. But inventors still continue to make these same mistakes.

Gear balance, behaves like a Roverval balance.

We said that a balance on the Roberval principle could be made with gears. Here's an example. Five gears are on a rigid beam IH. The bar is free to rotate about the central axis located on the support pedestal. The gears rotate, except for the center one that is fastened to the pedestal and cannot move. Horizontal arms LI and KE remain horizontal at all times, for they are fastened to the gears at each end of the main beam IH. Therefore the equal mass round weights P and p can be placed anywhere on these arms and the system is still in balance. In fact, if the arm KE were made extremely long, both weights could be on the left side of the central axis. They could be directly above or below each other, or p could even be to the left of P. The system would still be in balance.

The weights on the pans of a pan balance are adjusted to equality. Fine adjustment is accomplished with a simple lever scale incorporated into the instrument. A Roberval linkage could be made to balance unequal masses, or to balance for a particular ratio of masses, say 1:10.

Meccano model of a geared Roverval balance.

Thinking about these systems leads us to reformulate naive ideas about balances. The law of the simple lever is, Aa = Bb where A and B are masses on lever arms a and b. The lever arms are the lengths from the centers of mass of A and B to the rotation axis. But we can also interpret a and b to be the radii of the arcs of the motion of A and B as the system moves near the equilibrium point.

The pans of a Roberval balance move in such a way that the radii of the arcs of motion of the weights are independent of their position on the pan. Wherever the weight is placed on the pan, it moves the same vertical distance . That's the "Roberval principle" common to all of these kinds of balance.

—Donald E. Simanek, 2004, 2011.

Return to front page.
Return to The Museum of Unworkable Devices..
Return to the top of this document.