Center of gravity analysis of machines.

by Donald E. Simanek

One of the reasons that analysis of unworkable devices is fascinating and educational is that there are usually several valid approaches, all leading to the same conclusions—the devices don't work. Some methods that we have used on these pages include:

Stevin's principle.
Free-body analysis, using forces and torques.
Analysis using energy principles.
Center of gravity considerations.
Conservation laws and thermodynamics laws.

But we have negleted method 4, center of gravity. It has the advantage of being easy to grasp by people who are uncomfortable with vector mathematics.

Take, for example, the most ancient of perpetual motion wheels, the Baskara Wheel. It consisted of a wheel with evenly spaced vials partially filled with sand, mercury or water. Supposedly the weight of the material in the vials was always biased toward one side of the wheel, causing that side to fall, and since the "overbalance" was present in every wheel position, the wheel should turn continually. But, to the frustration of believers ever since, the wheel just sits there unmoving.

We often hear folks say that the possibility of perpetual motion was believed because science hadn't advanced sufficiently to deny it. I don't buy that. Let's examine this idea using only principles well known from the time of the ancient Greek philosophers to the time of Newton. Specifically these two principles:

Inanimate bodies move downward if they are not prevented from doing so by some constraint or external intervention.
A system of bodies moves to make its center of gravity lower, if not constrained to prevent that.
Water seeks its own level.

One never sees round objects rolling continuously uphill unless something is obviously pushing them. This observation of a fact of nature was well known, even though Aristotle gave some unhelpful mystical justifications for it. Indeed, from the first two principles we can easily derive the third.

Consider this container partly filled with water. To simplify the following analysis, assume that the container is rectangular in shape, like a glass acquarium. Why does the water assume a level surface and not some other surface? Water is easily moved around, and if you stirred it vigorously it would no longer have that flat surface, but when you stop stirring it would soon settle down to a flat surface. What's the reason?

The ancients were good at geometry, and understood the importance of the concept we call "center of gravity". For a uniform density material, the center of gravity is its geometric center. The center of gravity of the water is shown here as "×".

Why couldn't the water surface remain tilted as shown here? The volume is the same as before, only part of the volume has moved up. The center of gravity of the water is no longer at ×, but has moved up to a higher position. The ancient mathematicians knew how to find the position of the new center of gravity. It is a simple exercise to find the center of gravity of a triangle. You just draw two lines from triangle vertices to the midpoints of the opposite sides of the triangle. These lines cross at the center of gravity.

This is shown here. The previous water level is shown as a horizontal dashed line. Clearly the center of gravity has moved up from its previous position (C) and is now at (C'). This is an unstable situation, since every bit of the water is free to move so that the water will flow so its center of gravity will assume its former position at C. This is as low as it can go, for water is highly incompressible. If you push the water surface down somewhere it rises up somewhere else. The water cannot, by itself, climb up the sides of the container and spill out, because to do that it would have to raise its center of gravity again.

The classic perpetual motion devices were usually in the form of a wheel. Consider a wheel with a heavy ball attached to its rim (black in the diagram). If the wheel were free to turn on its axle, we know that it would rotate clockwise due to the unbalanced weight.

In fact, it would rotate clockwise until the weight was raised up on the left nearly as high as it was on the right, then back counterclockwise, continuing this pendulum-like motion, but would eventually end up at rest at its lowest possible point, shown here. Medieval natural philosophers spoke of a body's "impetus" as a mysterious property that sustains motion, but is somehow "used up" until the body comes to rest. We mention this only to show that natural philosophers knew that this is the way nature operates, and that wheels eventually come to a stop, no matter how well balanced they are and no matter how smooth their bearings. Today we attribute this slowing of motion to friction.

This is a good place to mention Galileo's interrupted pendulum experiment. A weight (black ball) is suspended from a support by a string. It is pulled to point A and released. The string hits a peg and the weight swings in an arc of smaller radius on the right, only to point B, nearly the same height (the dashed line H) as point A. Even if the bob is released from a height greater than the height of the peg, it will only rise on the right to the same height it had initially. We assume the release is gentle, without giving the weight any push. This standard demonstration of introductory physics courses is very instructive if accompanied by a discussion of kinetic energy and potential energy, free body diagrams, force, torque, momentum and impulse, and it can even be used to illustrate Lagrangian methods of dynamics. But we needn't do that here. Here we mention it only to show how nature behaves.

Finally we return to the Bashkara wheel. To simplify analysis let's consider just two of the tilted vials, on opposite sides of the wheel. Suppose there's one heavy ball in each vial. In this position of the wheel, they will be at their lowest possible position, as shown, and their center of gravity is halfway between them. "Aha!", says the believer in perpetual motion. If the wheel rotates a bit counter clockwise, the center of gravity will move to a point just beneath the axle, which is lower. But then the wheel will just oscillate back and forth till its "impetus" is gone (using the language of the medieval philosophers).

Now let's add two vials, making four. One thing is obvious immediately. Because the balls are free to move within their vials to the lowest point, then the center of gravity of all the balls taken together is lower than the center axis of the wheel. The position shown is not necessarily the position of lowest center of gravity, so the wheel will rotate and then oscillate until it finds that lowest c.g. This will be true no matter how many vials are placed on the wheel.

Adding balls within the vials doesn't improve anything. In fact, if the vials were filled with balls, they could not move within the vials, and the center of gravity of the whole system would be exactly at the center axle of the wheel. A simple flywheel would work as well, and if given a push might turn forever—if only you could somehow make it frictionless.

Replacing the balls with sand or liquid doesn't help either.

But our perpetual motion seeker objects. He observes that at some point in the wheel's rotation two of the opposing vials will be horizontal, and at that point the vial's contents shift from one end of the vial to the other. Maybe that's where the wheel might get some impetus to continue its motion. But, it can't help, for even during this shift the center of gravity of the contents of these two vials still remains at the same level, and combined with the center of gravity of all the other balls, the center of gravity of all taken together is still below the wheel axle.

Finally we can attack the little puzzle we proposed back in 2004. It is a wheel with three chambers partly filled with liquid. It has three-fold symmetry, but the liquid inside moves (seeking its own level) as the wheel is turned. Anyone who tackles this by detailed analysis of geometry and free body analysis is in for a messy problem.

But is that necessary? Is it like using a sledgehammer to crack a walnut?

Lets suppose the wheel, empty of water, is perfectly balanced. Now completely fill the three chambers with liquid. It is still balanced. The center of gravity of liquid in each chamber is fixed in position relative to the wheel, for the liquid inside cannot change its shape. Now consider the chambers only half-filled with liquid. The liquid inside a chamber has a center of gravity that is always lower than that of the fully filled chamber, no matter what the wheel's position. So the center of gravity of all the liquid (all three chambers) is lower than when they were full, and is always lower than the wheel's axle in any positrion of the wheel.

This can be seen by considering how you could make the center of gravity at the center axis of the wheel. It can only be done only by filling the chambers completely. Any smaller amount of liquid will cause the center of gravity of the liquid in all the chambers to be lower than the center of the wheel.

After all these years since 2004 no one has proposed a simple solution to this puzzle, though some have noted that "It should be obvious." Yes, most physicists and engineers will take one look at it and say "It won't work." Yet few care enough to explain how they know that it won't. In February 2011 one finally did:

That’s an easy one...
The center of gravity will always be lower than the center of the wheel.
So, it would require an external force to move it [continually] in either direction.
Since the center of gravity will always be lower [than the rotation axis], independent of the position of the wheel (and the shape of the volume occupied by the liquid), the wheel will stay in equilibrium, no matter what its initial position.
The key to failure of a gravity wheel design is precisely that: having the center of gravity always lower than the center of the wheel. The end result will be similar to a rope with a weight at its end. It reaches equilibrium when the weight is at the shortest possible distance from the most massive attracting mass (Earth).
And there is a solution to all of this... An easy and logical one.
Best regards, Alex Pannis. Technologist.

I am guilty of misleading readers by suggesting that this might be a difficult puzzle. Well, it might be, if you make it so.

Gravity wheels are wheels with a fixed axis, intended to rotate continually about that axis. They usually have mechanisms, gimmicks and moving parts attached to the wheel and only to the wheel. The fixed axis is the device's only anchor to the outside world.

We can state a useful principle that can be applied for quick analysis of other gravity wheel proposals, without the complications of force and torque anaysis.

If a gravity wheel's center of gravity is always lower than its rotation axis for any position of the wheel, it cannot initiate or sustain unidirectional motion by itself.

This does not say that a gravity wheel with its center of gravity above the rotation axis will move continually by itself.

This simple rule applies only to this limited class of devices, not to all devices. For example, a pendulum is not a gravity wheel. Its fixed axis is its point of support and if friction and other dissipative processes could be eliminated it would qualify as a perpetual motion device. But no energy could be extracted from it without slowing it eventually to a stop. Unbalanced wheels typically can oscillate in the same way, but cannot sustain unidirectional rotation. To qualify as a perpetual motion wheel, the wheel's center of gravity must be able to "get over the top", that is, to reach the maximum height the constraints allow, with enough speed to continue in one direction, cycle after cycle.
Curiously, inventors never seem interested in inventing perpetualy swinging pendulums. Oscillatory motion can, we know, drive wheels (consider the steam locomotive), so why aren't inventors devising pendulum over-unity devices that can drive a wheel, or are otherwise coupled to something on which they could do useful work? Inquiring minds want to know. I can recall only a very few proposed devices of this sort. And they didn't work.
Oscillating objects need not have fixed axles. A rocking chair doesn't, and its contact with the outside world is through its contact with the floor, which changes periodically. Its center of mass is always above that point. Such devices require a far different analysis. I'm suprised no inventor has tried to use this mecchnism in a perpetual motion device.

Extension to other unworkable devices.

Ever since I began "collecting" perpetual motion device proposals, I struggled with two questions.

Why do these unworkable proposals still seem persuasive to many people?
How can one determine and explain, in the simplest possible way, that they don't work?

So I tried to avoid unnecessary technical language and mathematics, limiting myself to Newton's laws, with only occasional mention of work and momentum. That was surprisingly successful, I think, and is done on my other web pages on this subject. But, I wondered whether we could get by with even less, at least in some cases. Could we avoid the force concept, momentum, energy, and work? In the examples above, we have. Using only concepts that would have been familiar to the ancient Greek philosophers, Medieval thinkers, Leonardo da Vinci, and Galileo, we can show why many such devices don't work. How far can we go with this? This "minimal" analysis is appropriate for readers innocent of physics and its technical language. I do not claim it can substitute for more rigorous analysis using classical mechanics laws. But it is closer to the way most non-scientists think.

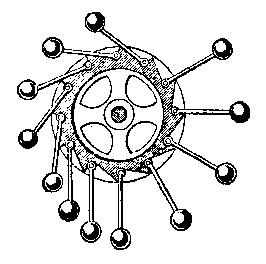
Consider this version of the Villard wheel, which is sometimes attributed to Jacob Leupold (1674 –1727). However, Leupold knew very well that it wasn't perpetual motion. Where is the center of gravity of the weights on the articulated arms? If all the weights were fully extended, the c.g. would be at the wheel's axle. But since some weights are flopped over, this lowers the c.g., so it is never as high as the wheel axle. Conclusion: perpetuum immobile.

Frank Walerij's device.

Here's a belt device. Look familiar? Balls in short enclosed tubes evenly spaced around the belt. It's just the Baskara device extended by using a belt. Where's the c.g. of the balls?

In belt devices we must compare the center of gravity of the movable elements to the center of gravity of a plain belt on the same two pulleys. In this case, halfway between the belt axles.

In any position of this device, its c.g. is always below the c.g. of the device without the shifting balls. It isn't going anywhere.

Yes, this was granted a German patent.

Almost every unworkable belt device is just this sort of extension of a wheel device, and it fails for the same reasons.

This old idea imagines a rotating sphere or cylindrical drum half immersed in water on the left, with a marvelous waterproof and frictionless seal to prevent water leaking out. The inventor supposed that the buoyant force of water would lift the left portion of the drum and cause it to rotate. This is a good puzzle to expose misunderstandings of buoyancy. Smaller minds will fuss about irrelevant considerations such as "You can't make frictionless and leakproof seals." Of course you can't, but that's not the point of this thought experiment, which we discuss elsewhere. But now, look at the center of gravity of all the moving parts of this device. Surprise! Even if the drum is rotated (by hand), its center of gravity never changes. So in any position, there's no other position with lower center of gravity, so the system has no tendency to move.

This 19th century invention by F. G. Woodward suggests that since there's more weight of ring to the left of the two rollers, it must turn counterclockwise. Even naive students immediately recognize that there's no reason to expect it to turn. Does our center of gravity principle apply? It qualifies as a supposedly gravity-driven wheel. The ring's center of gravity never goes above its own rotation axis. The rollers' centers of gravity never go above their rotation axes either. This curious paperweight will just sit there, unmoving.

By now, anytime you see an overbalanced gravity wheel, your first impulse should be to look at the center of gravity of the moving parts! If the center of gravity can never move above the wheel's rotation axis it's a non-starter.

Irrelevant, but interesting, observations.

Perpetual motionists often display a perverse addiction to their beliefs, even when their reasons for belief are demolished, one by one. Talking with them is a "whack a mole" game. When the fatal flaw in their idea is exposed, their response is often, "Yes, but what if...." followed by some proposed modification of the device that will, they think, ensure success.

The above examples do ignore some possible modifications. They assume slow motion of the wheel, and generally do not mention centrifugal effects. It turns out these don't matter, but let's consider them for a bit. They result from the fact that bodies in motion, unconstrained, continue in that same motion unless something (some physical constraint) prevents that. [Newton refined that observation into his first law of motion.]

Consider the Bashkara wheel with balls in tilted vials. If the wheel were spun rapidly enough by hand, the balls would stay at the outer end of the vialband the center of gravity would be exactly at the wheel's axles, and could go no higher. Any of our example devices would then continue spinning indefinitely if they were friction-free, as would a simple flywheel on frictionless bearings.

Return to The Museum of Unworkable Devices.
Return to Donald Simanek's page.