Whatever Were They Thinking?
The early history of perpetual motion isn't always easy to interpret. Sometimes we have only second-hand accounts, and usually we haven't a clear notion of exactly what the inventor assumed might make the device "go". But certain flawed ideas persist and evolve, and this gives us tantalizing clues. We examine some of these historical threads of the evolution of perpetual motion ideas.
When this document is finished (if ever) it will have several chapters:
- Mass Transfer Devices.
- Swinging hammers.
- Rolling Balls.
- Magnetic motors.
- Inclined ramps.
Mass Transfer Devices
 |
 |
The cover from the August 1922 issue of Science and Invention magazine shows artist Howard Brown's version of the overbalanced wheel of Fig. 2. Joseph H. Krauss, author of the article "The Truth About Perpetual Motion" on pages 338 and 339, describes the operation of the device as follows.
Fig. 2 is the machine shown on the cover of this magazine, and illustrated herewith: 1 are weights, 2 are cylinders in which pistons, 3, operate, through levers, 4. In the position of the machine shown, piston 3A in cylinder 2A is being forced upward by weight 1A, acting through lever 4A, compressing the mercury in those pistons and forcing it through the tube 5 to cylinder 2b. The piston 3B is being lifted upwardly, forming a partial vacuum in that side of the machine, caused by weight 1B descending and acting upon the piston 3b through levers 4B. This action, according to the inventors, Messrs. Larson and Ross, is to continue indefinitely.
The editors of the mazazine offered a prize of $50 for the best reason why the machine would not operate. They should have offered a prize just for making sense of the description. We wonder how much artist Harold Brown got for rendering it in color for the cover.
This is a category of perpetual motion device that has no accepted name, but might be called a "mass transfer device." What were the inventors thinking? They supposed that a gravitational force on one side of the wheel could be used to force mass (in this case, mercury) to the other side of the weheel, thereby making that side heavier. Then do it repeatedly as the wheel turns. It is somthing like what we have called the "Baron Munchausen bootstrap principle", or the "dog chasing its tail". In order to accomplish the mass transfer, these devices were generally mechanically complex. Perhaps that was one reason for their appeal, for they were sufficiently complex that even the inventors couldn't quite grasp the multiple interacting physical principles that applied, so they imagined something magical might be happening.
Krauss concludes his article: "For once and for all let us state that perpetual motion is an impossibility, and the inventors of these devices are either stupidly following false ideas or knowingly attempting to defraud the public."
Rolling Balls.
Bob Shadewald made this nice color picture of a perpetual motion machine. It has captive weights rolling in chambers on the wheel. On one side the weights move along the curved portion, and on the other side they move along the straight portion. This machine is sometimes falsely attributed to Leonardo da Vinci, just because he made drawings of it and similar perpetual motion wheels.
 |
Whatever the inventor had in mind, here's our interpretation of it.
It's a well-known fact that a ball can roll down a suitiably shaped shallow curved
incline in less time than it can roll down a straight incline between the
same two points, even though the fastest path is longer in length. This is the famous
Brachistochrone "challenge" problem posed by
Johannn Bernoulli in 1696, and solved by him, by Isaac Newton, and others.
It sought to find the particular mathematical
curve that gives the path of least time of descent between
two points at different heights, under the action of gravity.
The curve that accomplishes this is called a cycloid.
This is a common physics demonstration even today.
The result is surprising to most people, for it shows that path of the shortest time of travel between two points under the action of gravity is not always the shortest distance between those points. In fact this result is just another example of how non-intuitive many physical phenomena are, including those affecting a wheel with shifting weights in a gravitational field, which bears directly on the reasons why many people were seduced by clever designs for perpetually turning wheels.
 |
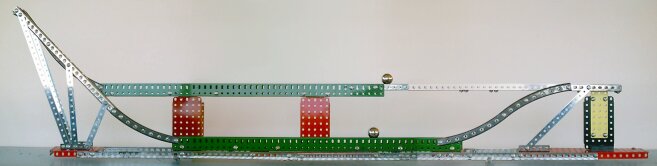| Steel construction set model. The ball that takes the lower track wins. |
|---|
The picture shows the race between two balls released from rest at the upper left. The ball that takes the lower track reaches the right end sooner than the one that took the straight track. In this model, the ball on the lower track wins the race, but the outcome does depend on the length of the straight sections.
One can't help being struck by the similarity of the boundaries of the chambers in these perpetual motion wheel devices to the straight and curved paths in the Brachistochrone problem. Could the inventor have had this in mind? If so, the inventor might argue in this manner:
The balls on the left move along the curve from rim to axle in less time than the balls on the right move along the straight line from rim to axle. Therefore there will always be more weight of balls on the right side.What's wrong with this argument?
All material in this museum is © 2002, 2005, 2008, 2012 by Donald E. Simanek, with the exception of text and materials quoted from other sources.
Return to front page.
Return to the top of this document.
Return to The Museum's Main Gallery.