Conceptual problems
about accelerating systems.
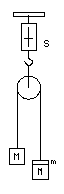
1. The Atwood machine shown is suspended from a spring balance. The mass on
one hanger is M, that on the other is (M+m). Suppose the heavier side (right
side) hanger is fastened to the top pulley by a thread. The scale reads (2M+m).
The thread is burned and the system accelerates. While the masses on the Atwood
machine accelerate the spring balance reads
- the same as before.
- more than before.
- less than before.
- zero.
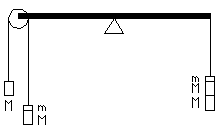 2. An atwood machine is suspended from one side of a
beam balance. The Atwood machine's masses are held in
place by a string, the system being in static balance. The
string is then burned. During the acceleration the left side of the
balance
2. An atwood machine is suspended from one side of a
beam balance. The Atwood machine's masses are held in
place by a string, the system being in static balance. The
string is then burned. During the acceleration the left side of the
balance
- does not move at all.
- moves up.
- moves down.
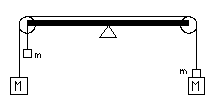 3. A beam balance is used again. This time the atwood machine spans all the way across. A
small mass m is required to balance the system initially, when the Atwood machine's masses are
held in place by a string. The string is then burned. During the acceleration the left side of
the balance
3. A beam balance is used again. This time the atwood machine spans all the way across. A
small mass m is required to balance the system initially, when the Atwood machine's masses are
held in place by a string. The string is then burned. During the acceleration the left side of
the balance
- does not move at all.
- moves up.
- moves down.
Problems such as these were much discussed and analyzed in the history of mechanics. See, for example, Mach, Ernst. The Science of Mechanics, a Critical & Historical Account of its Development. 1893. English translation by Karl Menger. Open Court, 1960.
The following is from Mach's book. Note that Mach uses γ for the actual acceleration. Also he speaks of "pressure" and "presses on" where we would prefer to say "force".
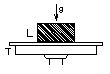 |
...We consider, say, a load L on a table T. The table is pressed by the load just so much, and so much only, as it in return presses the load, that is prevents the same from falling. If p is the weight, m the mass, and g the acceleration of gravity, then by Newton's conception p = mg. If the table be let fall vertically downwards with the acceleration of free descent g, all pressure on it ceases. We discover thus, that the pressure on the table is determined by the relative acceleration of the load with respect to the table. If the table fall or rise with acceleration γ, the pressure on it is respectively m(g-γ) and m(g+γ). Be it noted, however, that no change of the realtion is produced by a constant velocity of ascent or descent. The relative acceleration is determinative... [In another discussion, Mach emphasises: An unaccelerated motion ... would not disturb the equilibrium. But we cannot pass from rest to motion without acceleration.]A pendulum with the time of oscillation T = π(L/g) would acquire, if its axis received the downward acceleration γ, the time of oscillation T = π[L/(g-γ)], and if let fall freely would acquire an infinite time of oscillation, that is, would cease to oscillate.
We ourselves, when we jump or fall from an elevation, experience a peculiar sensation, which must be due to the discontinuance of the gravitational pressure on the parts of our body on one another—the blood, and so forth. A similar sensation, as if the ground were sinking beneath us, we should have on a smaller planet to which we were suddenly transported. The sensation of constant ascent, like that felt on an earthquake, would be produced on a larger planet.
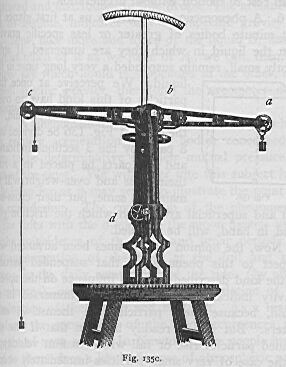
Poggendorf's apparatus. From Mach. The conditions referred to are very beautifully illustrated by an apparatus constructed by Poggendorf. A string loaded at both extremities by a weight P is passed over a pulley c attached to the end of a scale-beam. A weight p is laid on one of the weights first mentioned and tied by a fine thread to the axis of the pulley. The pulley now supports the weight 2P+p. Burning away the thread that holds the overweight, a uniformly accelerated motion begins with the acceleration γ, with which P+p descends and P rises. The load on the pulley is thus lessened, as the turning of the scales indicates. The descending weight P is counterbalanced by the rising weight P, while the added overweight, instead of weighing p, now weighs (p/g)(g-γ). And since γ = [p/(2P+p)]g, we have now to regard the load on the pulley, not as p, but as p[2P/(2P+p)]. The descending weight, only partially impeded in its motion of descent, exerts only a partial pressure on the pulley.
Answers
 Case 1. Assume that the hanger mass is included in the value M, and the pulley
has negligible mass and moment of inertia.
Obviously the left hanger accelerates upward and the right hanger downward.
Call this value a.
The tension on the string joining the two hangers is
considered uniform throughout the string.
Case 1. Assume that the hanger mass is included in the value M, and the pulley
has negligible mass and moment of inertia.
Obviously the left hanger accelerates upward and the right hanger downward.
Call this value a.
The tension on the string joining the two hangers is
considered uniform throughout the string.Taking signs as positive in the direction of the acceleration, the force equations are:
Left side: T - Mg = Ma
Right side: (M+m)g - T = (M+m)a
When one simply wishes to find the acceleration, these equations are solved to eliminate the variable T. The result is:
mg = (2M+m)a , or a = mg/(2M+m) .
This is an intuitively appealing form of the resuslt, for it says that the unbalanced force accelerating the system, mg equals the total mass (2M+m) times the system's acceleration, a. We could have used this idea to calculate the acceleration very simply. This tempts one to try a similar intuitive shortcut with other rope and pulley problems. But this can cause serious blunders when the two parts of the system don't have the same acceleration, as when there's more than one rope in the problem, or when a rope is attached to something that is not accelerating, like a solid floor or ceiling.
When we want to know T we eliminate the variable a. Again, the result isn't pretty:
T = 2g(M+m)M) /(2M+m).
The spring balance reading is twice as much,
T = 4g(M+m)M) /(2M+m) .
One should always check such results with a known case, for it's very easy to make sign or notation errors in the mathematical steps of derivation. The test case is this: When either mass is zero, the tension should drop to zero and the acceleration of the other mass should be g.
But this brute force mathematical derivation isn't necessary to obtain an intuitive answer to the question. The motion of the masses simply moves the center of the mass of the system. The center of mass accelerates downward.
The scale exerts an upward force Fscale = (2M+m)a where a is the downward acceleration of the center of mass.
If the masses weren't accelerating, the spring balance would exert a force of just (2M+m)g, the total weight of the suspended masses. When they are accelerating, M moving up, and (M+m) moving down at the same instantaneous speed, the center of mass of the two amounts M remains fixed. But the center of mass of the small additional mass m moves downward with acceleration a. The spring scale reading is therefore reduced by amount D F = m(g-a). In the above derivations I took the unorthodox strategy of labeling the masses on the atwood machine as M and (M+m). This expresses results in terms of the unbalance amount, m. Here's an example of where that strategy pays off. Let's find the force, S registered by the spring balance without finding the tension in the string, by looking at what happens to the center of mass of the system.
The center of mass of the two masses of size M does not move. The unbalanced extra mass m accelerates upward at value a. So the spring balance reading, S, is an amount ma less than in the static case, ie.
S = (2M+m)g - ma
This reduces to
S = 4M(M+m)g/(2M+m) .
This was a good way to check the previously derived answer for blunders. In fact, it is (I think) the method Mach used in the excerpt above. But we could only get away with it here because of the relatively simple system we are dealing with. As we noted above, when a system has more than one string, or a string attached to a fixed object, it's best not to try sneaky short-cuts unless you have justified every step.
Case 2. As a consequence of the above analysis, we conclude that the left end of the balance will move up. Experiment easily confirms this.
Case 3. This one is a bit tricky. When the motion is established, the tension is nearly uniform all along the string. so since the left side has the additional unmoving mass m, the left side should move down.
Notes on Ernst Mach
 The physicist Ernst Mach (1836-1916) is not well known to students today, but he was a pivotal
and somewhat enigmatic figure in late 19th century science. His critical book on mechanics is
valuable reading even today, for its historical and philosophical content.
The physicist Ernst Mach (1836-1916) is not well known to students today, but he was a pivotal
and somewhat enigmatic figure in late 19th century science. His critical book on mechanics is
valuable reading even today, for its historical and philosophical content.Mach is one of those who refused to accept the atomic theory. In a 1910 paper he admitted that the atomic theory had produced useful results, but that this still proved nothing of the reality of atoms. He grumbled that physicists were on their way to becoming a church, with the reality of atoms as its dogma. He didn't like relativity theory either, even though much of his own pioneering work in mechanics anticipated relativity. He refused to accept credit for this connection, saying in 1913:
I must as assuredly disdain to be the forerunner of the relativists as I withhold from the atomistic belief of the present day [1913]. I can accept the theory of relativity as little as I can accept the existence of atoms and other such dogmas.If atoms and relativity were to be required belief, then, said Mach, he would renounce the physical way of thinking, because freedom of thought was quite precious to him. Mach died in 1916, without ever accepting the reality of atoms.
Mach's skepticism about certain ideas in physics arose from his general opposition to metaphysical and spiritual incursions into science. He rejected any idea or concept which could not be directly measured. His philosophical position became known as antimetaphysical positivism.
Mach rejected as useless Newton's definition of mass as 'quantity of matter.' Instead, Mach defined mass by use of Newton's Second Law, measuring the acceleration produced by a known force.
Mach didn't like 'occult' concepts such as 'action at a distance.' He felt that such notions as force fields could not be directly verified by measurement, and therefore shouldn't be allowed in physics. We should not try to explain such things as gravitation. It was enough, he said, to state the mathematical 'functional connections' between observables. In this he is in harmony with Newton's refusal to make 'hypotheses' (models) to justify the laws of mechanics.
Though he disdained relativity, several of Mach's own ideas anticipated principles at the foundation of relativity. In particular, Mach rejected the notion of absolute space and absolute time. And he didn't like the ether theory either!
Notes on Johann Poggendorf.

|
The German physicist and chemist Johann Christian Poggendorf (1796-1877) is best known today for the "Poggendorf" illusion of alignment of a partially obscured straight line (1860). His contributions to physics covered most of the classical fields of physics. He showed that the difference in potential of two conductors may be measured by balancing it against a known difference of potential such as that of a voltaic cell. This became a standard technique of electrical measurement, still used today. He invented a moving-mirror ("multiplying") galvanomter and a magnetometer. In collaboration with Leibig, he coined the chemical term 'aldehyde'.
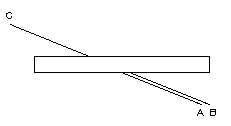 |
Poggendorf's illusion of alignment. Which segments represent a straight line, AC or BC? If you print this picture, or view it on a perfectly linear display screen and lay a straightedge along line C, you will see that the answer is BC. Yet most persons perceive that segments AC are aligned. |
|---|
Poggendorf edited the journal Annalen der Physik und Chemie from 1824-74 (160 volumes). He also compiled a two-volume comprehensive bibliographical dictionary of 8000 physicists from all countries and periods, through 1858.
Return to Physics demos.
Return to Physics problems to challenge insight.
Return to Donald Simanek's page.