|
Physics Problems
Compiled and annotated by Donald Simanek
to Challenge
Understanding,
emphasizing concepts,
and insight.
Textbooks abound with problems intended to develop one's mastery of physics. Too many are "plug-and-chug" without much opportunity to form deeper insights into physics. This is a collection of problems that are designed to require insight and correct use of basic physics concepts, but do not require mathematical drudgery.
That class of problems appears in some physics textbooks, particularly older textbooks. Often the problems proposed idealized and "unrealistic" situations to keep things simple. This seems to bother students today a lot more than it did in the 50s when I was attending the University of Iowa. Somehow we caught on to the idealizations and simplifications stated (or often just implied). Today many students want everything "spelled out". They seem to be continually looking for "defects" in problems, but not with the purpose of furthering their understanding by coping with ambiguity, but to provide an excuse for not even thinking further about the problem. They are mired in a concrete view of reality, and say, in effect "We don't have to understand the subtle implications of this, we can just do a computer simulation of it if we need an answer." The conceptual and intellectual challenges of such a problem seldom interest them. Frightening! For that audience, I shall try to be quite explicit in the statement of the problems.
I'll be continually tinkering with this document and adding to it. Some answers, and explanations of answers, may not be given yet but may show up at a later time. Readers are invited to submit answers and explanations (and criticisms) to me at the address shown to the right.
There's a surprising number of books devoted to physics problems and puzzles:
- Miller, Julius Sumner. Demonstrations in Physics. Ure Smith, Sydney, London, 1969.
- Miller, Julius Sumner. Millergrams. Two volumes, paperback. Doubleday, 1970.
- Jargocki, Christopher P. Science Brain-Twisters, Paradoxes, and Fallacies. Scribners, 1976.
- Jargocki, Christopher P. More Science Braintwisters and Paradoxes. Van Nostrand, Reinhold, 1983.
- Jargocki, Christopher P. and Franklin Potter. Mad About Physics, Braintwisters, Paradoxes, and Curiosities. John Wiley and Sons, 2001.
- Grimwall, Göran. Brainteaser Physics, Challenging Physics Problems. Johns Hopkins University Press, 2007. With answers and references.
- Gnädig, Honyek & Riley. 200 Puzzling Physics Problems With Hints and Solutions. Cambridge University Press, 2001.
- Cronin, Jerimiah A., David F. Greenberg and Valentine L. Telegdi. University of Chicago Graduate Problems in Physics. Addison-Wesley, 1967. Problems and solutions from the comprehensive exams for entering graduate students.
- Epstein, Lewis C. Thinking Physics is Gedanken Physics. Insight Press, 1983, 1981, 1979. Some answers are printed upside down, which is a nuisance. This is a revised and expanded compilition based on Lewis C. Epstein and Paul G. Hewitt's Thinking Physics, 1979.
- Ghose, Partha and Dipankar Home. Riddles in Your Teacup, Fun With Everyday Scientific Puzzles. Second Edition. Institute of Physics Publishing, 1994. Most of these are based on everyday pheonomena.
- King, A. R. and O. Regev. Physics With Answers, 500 Problems and Solutions. Cambridge U. Press, 1997.
- Povey, Thomas. Professor Povey's Perplexing Problems, Pre-University Physics and Maths Puzzles with Solutions. Oneworld Publications, 2015.
- Gardner, Martin. Space Puzzlers, Curious Questions and Answers About the Solar System. Simon and Schuster, 1971. Also a Archway/Pocket Books paperback, 1972.
- Gardner, Martin. The Collosal Book of Short Puzzles and Problems. Norton, 2006. Two chapters devoted to physics puzzles.
- Townsend, Charles Barry. Merlin's Puzzler. Hammond, 1976. Townsend's collections of old puzzles are mostly logic and mathematics puzzles, but some relate to physics.
- Townsend, Charles Barry. Merlinn's Puzzler 2. Hammond, 1977.
- Townsend, Charles Barry. Merlin's PUzzler 3. Hammond, 1979.
Contents
- Astronomy
- Solar System. Stop the Earth.
- Mechanics
- Tug of war, rolling on an infinite plane, sliding paradox, braking automobile, bouncing ball, a boring problem, skater's spiral.
- Mixtures
- Liquid transfer problem.
- Hydrostatics
- floating test tube, hydrostatics puzzles, physics on the lake, milk bottle, balance problem.
- Optics
- mirror reversal, simple magnifier, best image, microscope magnification.
- Atwood's machine variants.
- Why are some pulleys crowned?
Solar System
Our earth has orbital motion, revolving once around the sun in about 365 days. Suppose that this orbital motion suddenly stopped completely, but everything else remained the same. How long would it take for the earth to plunge along a straight line into the sun?I begin with this one because it is an example of the advantages, and practical disadvantages, of using insightful methods for homework problems. This was an assigned homework problem in classical mechanics when I was a university student in 1957. Always looking for ways to avoid excess work, I deliberately tried to find the answer by simple means. However, my professor did not take kindly to the solution I submitted, though I was quite proud of it. He wanted it done in the conventional "textbook" manner, just to show that I had read the textbook and mastered its methods. So I got no points for my ingenuity and insight.
Many years later I happened to meet the author of the textbook, so I related this anecdote to him. He said, "That's an old problem that has been in many textbooks. You did it the way I hoped students would discover. Why use a sledgehammer to crack a walnut?"
With that warning, here's the simple, non-calculus, way to arrive at the answer, which will set the tone for this document.
Answer:
Kepler's law applies to planetary orbits, whether they be of circular, or elliptical shape. It says that T22/T12 = R23/R13, where T is the period of an orbit and R is its semi-major axis. The semi-major axis is the average of the planet's maximum and minimum distances from the sun.
Let the earth's mean radius be R1. Now, if the earth's orbital momentum were suddenly reduced (without exerting anything but a tangential stopping force on the earth), it would fall straight to the sun. This straight fall can be considered 1/2 of a degenerate elliptical orbit with major axis equal to R1. Its semi-major axis is R1/2 (the average of R1 and zero). Its period will be designated T2.
So: T22/T12 = (R1/2)3/R13 = (1/2)3
And therefore, T2 = T1/23/2 = 0.353 year, and the time to fall into the sun is 1/2 of that, or 0.176 years or 64.52 days—a bit over two months.
So what fault had my first professor found with this elegant solution? Several. I had not established and justified that a straight line trajectory into the sun is really a degenerate ellipse. To do that I probably should have taken the limit as an ellipse's minor axis decreased to zero. Had I dealt with the fact that my crash orbit passes through the center of the sun, where its distance from the sun's center is zero, and the gravitational force there is infinite? Well actually it isn't infinite there since the sun isn't a point object (see problem below), and the earth would have vaporized before reaching the center. Nonetheless, if such a catastrophe happened, we can predict that the earth would take a bit over two months to make its plunge to a fiery death, giving us some time to enjoy the show before frying to a crisp.
Mechanics
Tug of War
Many students, even those who get good grades, exhibit modes of thinking that are counterproductive for later work. Teachers may be unaware of this because they don't ask the questions that could expose conceptual misunderstanding. Multiple-choice questions won't. Even short problem exams won't, if one only looks at the answers.
|
Here's an example, which I'll put in a multiple-choice format as best I can.
Arnie and Wimpy are playing tug-of-war. Arnie wins, pulling Wimpy into the mud puddle. Why did Arnie win?
a) Arnie exerted a greater horizontal component of force on the rope than Wimpy exerted.
b) The rope exerted a greater horizontal component of force on Wimpy than it exerted on Arnie.
c) Arnie exerted a greater horizontal component of force on the ground than Wimpy exerted.
d) All of the above.
Answer.
At the start everyone is at rest. For someone to win, there must be an acceleration from rest to initiate motion in one direction. This acceleration need not be large. During the acceleration there must be a net horizontal force component on each person in the direction of the acceleration.
(a) and (b) are wrong because the rope has relatively small mass, so the tension forces at its ends are nearly the same size (F =ma where m is essentially zero). Certainly any small difference in tension between the two ends is nowhere near great enough to account for the outcome. The rope's mass is very small compared to the masses of Arnie and Wimpy, so even when it accelerates (and so do they), the net force on the rope, F = ma, is negligibly small compared to the net forces on Arnie and Wimpy. In fact, most of this game involves small accelerations.
(c) is correct. The net horizontal force component on Arnie that allows him to accelerate with respect to the ground is the force of the ground on his feet minus the force the rope exerts on him, the former being larger. The person with the better shoes, greater coefficient of friction at the ground underfoot, and foot control, wins. Wimpy also accelerates, because the force of the rope on him is larger than the force of the ground on his feet. The player wins who can maintain the largest horizontal force between his feet and the ground.
(d) is the response most students choose.
After due consideration, I deliberately let stand the slight ambiguity of the phrase "the greater force" feeling that students should understand from context "greater than what?"
The student outcome is worse if you abandon the multiple-choice format and ask this as an essay question. Then you aren't "giving away" any possible answers, and students are far less likely to even consider (c).
In essay format, the question might be posed in this way.
Tug of War
Arnie and Wimpy are playing tug-of-war. Arnie wins, pulling Wimpy into the mud puddle. Explain why Arnie won, with explicit reference to the forces on Arnie, Wimpy, and the rope, and with explicit use of Newton's law F= ma and his third law.Follow-up to the tug of war problem. No. 1.
Since we have shown that the person wins who can maintain the greatest horizontal force component between feet and the ground, does this mean that the heavier player has the advantage?
Answer 1.
Yes, all else being equal. Whoever wins must have greater force of ground on feet than does the other team. Whoever wins must accelerate the total mass of all players, and that acceleration is the same whichever side wins. Contact forces, such as those due to friction, are proportional to the normal (perpendicular) force at the contact surface. If the game is played on a uniform level floor (same for all players), then the outcome depends on foot control and the coefficient of friction between shoes and floor. All else being equal, the heavier team should win. Outdoors on uneven and non-uniform ground, the terrain (and how effectively the players take advantage of it) may play the dominant role. Of course if one team uses spiked shoes, they may win even if they are lighter weight. [Thanks to William Katzman, Program Leader, LIGO Science Education Center", Caltech, for pointing out the importance of using "horizontal force components" in this question rather than just "force".]
Note that the question as posed didn't mention the players' relative weights. This was deliberate. Response (c) is still the correct answer, even if the greater force at the ground is due to the weight difference, or something else (rougher soles) or a combination of reasons.
Answer 2.
This form of the answer, in logical form, covers all strategies the winner might employ, moving backward, or standing still and paying in the rope.
- The loser must accelerate toward the mud.
- Therefore the loser experiences a net horizontal force toward the mud.
- The net horizontal force on the rope is nearly zero since it has such small relative mass.
- Therefore the forces on each end of the rope must be of nearly the same size at all times.
- The winner must not accelerate toward the mud.
- Therefore the net force on the winner must not be toward the mud.
- Therefore the force the rope exerts on the winner must be equal to or smaller than the size of the force on the winner's feet.
- So the force the rope exerts on the loser must be equal to or smaller than the size of the force on the winner's feet.
- But we already noted that the force the rope exerts on the loser must be larger than the force on the loser's feet.
- Therefore the horizontal force on the winner's feet must be larger than the horizontal force on the loser's feet.
I've been waiting for several years for someone to point out that the analysis above is incomplete. There's another way to win this game, especially applicable if only two persons are playing. One person can take advantage of the situation that may arise when one player begins to slip, as on wet ground or slippery grass. Then the person who is not slipping may "reel in" the rope, pulling the sliding player across the line. This takes advantage of the fact that sliding friction is generally smaller than static friction. However, on more careful examination, how can this situation arise? Unequal ground conditions are necessary.
But if both players were on a uniform surface, say slippery ice, could either player use this to advantage? Any jerk on the rope, to initiate slipping would cause both of them to slip. Any steady pull will have equal effect on both players. If either player shortens the rope, they both move toward their common center of mass. Aha! If they were equal mass and started equal distances from the line, it would be a draw. But if they were unequal mass and started equal distances from the line, the center of mass would be on the side of the heavier player, and the heavier player would win. Extrapolating this to the real case on irregular ground surface, where there's a lot of give and take and perhaps occasional slipping, the heavier player will have the advantage, as our previous analysis indicated, but for different reasons from those we considered earlier. Strength will have very little to do with it unless one player finds an especially firm patch of ground, then jerks the rope to cause the other person to slip, then takes advantage of that to pull the other player over the line.
And then, what if at the start, one player were closer to the line. That player should lose. How many textbooks that present this problem bother to consider all these contributing factors?
All of these qualifications, while interesting, do not change the fact that in the multiple-choice question we began with, answers (a) and (b) are clearly wrong.
Follow-up to the tug of war problem. No. 3.
Finally, someone raised the question of vertical components of force due to players of different heights. Leung Chi Ming of The Hong Kong University of Science and Technology suggests this strategy.
There is a practical way to win the game if all the factors are identical for the 2 teams. It's the arrangement of players. The tallest player should be in the front and the shortest player at the back. Please see my attached picture.
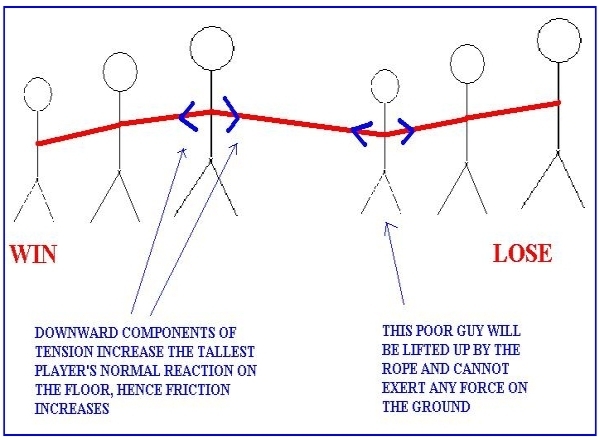
However, this raises some questions. On the left team, the tall fellow in front certainly is "lifting" his short opponent, and if it were only a contest between two people, one tall and one short, it seems clear that, all else being equal, the taller one has the advantage. The vertical components increase the normal force on the taller one's feet, increasing the friction at his feet. The short one's normal force is decreased and so is the friction.
But in the team case, the drawing above shows that the shorter players on the left team also experience an upward component of force, and therefore the friction on their feet is reduced. The shorter player on the right team certainly experiences decreased friction, but the other players on the right team have increased friction at their feet. So how does this all add up? In team play, would there be any advantage for a short player to run the rope over his shoulder, to increase its height and increase the friction at his feet?
But if it is a contest between just two players, the taller one should have an advantage.
Are there any official rules for the game of tug-of-war?
 |
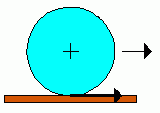 |
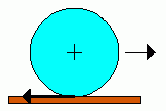
| The usual textbook depiction of the rolling ball with friction opposing the forward velocity. |
Friction the other way? Friction in the direction of the velocity. |
|---|
Some textbooks say that a ball or cylinder rolling on an infinite plane must come to a stop because "it is slowed by friction". As usually defined in textbooks, the force due to friction lies in the horizontal plane. If the force due to friction is in a direction to reduce the forward velocity, as shown in the first picture, it is in a direction to increase the angular velocity. If the force due to friction is in a direction to slow the rotation, as shown in the second pictre, it is in a direction to increase the forward velocity. Niether of these is consistent with a reduction in the cylinder's velocity. Clearly friction alone cannot stop the rolling body.
The resolution to this apparent paradox is to realize that perfectly rigid, non-deformable, bodies aren't possible in nature. All bodies deform somewhat on contact. This gives rise to non-uniform size reaction forces over the deformed surface of contact (and normal to it) and there can be a net torque from them. These reaction forces adjust size and distribution to allow slowing without slipping.
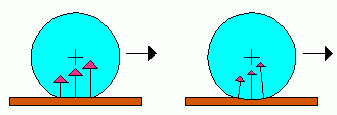 |
| The normal forces due to surface deformation. |
|---|
These figures show two cases. In the first case the ball deforms, but the surface doesn't. Normal forces are greater in front of the rotation axis, giving a net counterclockwise torque, the correct direction to slow the angular roation. In the second case, both the ball and the surface deform, but again the result is a net counterclockwise torque. Also, these forces normal to the deformed surface have components in the plane, whose sum is in a direction opposite to the forward velocity.
In fact, these forces also act to determine the exact deformation of the surface, and everything can adjust to slow the rolling body without allowing slipping.
Sliding.
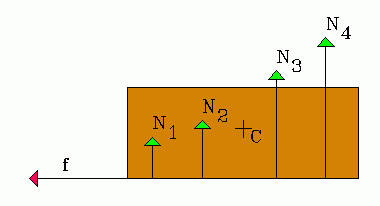
A block of wood is given a push and slides along a horizontal, flat table surface, coming to a stop. Of course it's obvious that friction is the reason that its kinetic energy drops to zero when it stops, work having been done as a result of the force due to friction at the interface between the table and the bottom of the block.(1) But what about the block's momentum? What happened to that?
(2) And what about the block's angular momentum? It doesn't have any, you say. Well, about its own center of mass it surely isn't rotating during this experiment. But why not? The only forces on the block are the forces due to friction at its bottom, the gravitational force, and the upward reaction force the table exerts on the block. The net (total) of the gravitational force and the reaction force must be zero, since the block isn't accelerating up or down. The force due to friction acts in the plane of the table and therefore has a lever arm about the center of mass, and therefore must exert a torque on the block about the center of mass. So why doesn't this set the block into rotation? There must be a counter-torque of equal size and opposite direction to the torque due to friction; but what is it?
 |
Solutions
(1) Momentum of a system is conserved. The horizontal momentum lost by the block is given to the table through the continuous impulse of the force due to friction at the table-block interface.
(2) This one is more interesting. Take an axis through the block's center of mass, a distance H above the table, where H is the height of the block's center of mass (C) above the table. The force due to friction f supplies a clockwise torque fH about this axis. The gravitational force is balanced about the center of mass, and gives no net torque. We conclude that the normal forces along the bottom surface of the block cannot be balanced about the center of mass, but must be greater at the front of the block, providing the necessary counterclockwise torque to balance the torque due to friction, and resulting in no appreciable rotation of the block about its center of mass.
See the next item for an application of this insight.
Braking automobile.
Automobile brakes are often designed differently for front wheels than for the rear wheels. The front wheels are more rugged, and sometimes are disk rather than drum brakes. Why is this?Solution
When the automobile brakes, you notice that the its front dips down. The front springs compress more than the rear ones, and the front tires do also. This indicates that there's more vertical force on the front axle than on the rear.
The reason this happens is that the force due to friction of the roadway on the tires produces a clockwise torque about the car's center of mass (see picture below). The vertical normal forces on the tires must adjust in size to produce an equal and opposite counterclockwise torque.
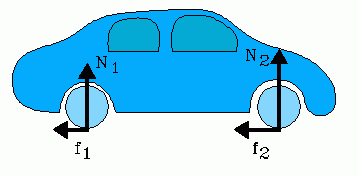 |
In the light of the previous problem of the sliding cube, the reason is clear. The center of mass of the vehicle is between the front and rear axles, and above them. Therefore the force due to friction between roadway and tires provides a torque that initiates a rotation, causing the downward dip of the front of the vehicle. This compresses the front springs more, causing the normal forces to be greater at the front wheels than at the rear. This torque is on the auto is opposite to the torque due to friction.
The forces on the tires due to friction f1 and f2 at the roadway are linked to the normal forces N1 and N2 through the relation f = μN where μ is the coefficient of friction. So, since N2 is greater on the front tires than is N1 on the rear tires, the friction at the roadway is also greater on the front tires. The brake drums or disks in the front also experience an increased force due to friction, hence they must be more rugged.
A boring problem.
Consider a rigid, solid Earth with radial symmetry and outside radius R, rotating with constant angular velocity ω. Start at the equator and drill a sufficiently large diameter hole straight through the center of the Earth and out the other side. Never mind that the center of the Earth is pretty hot, and probably flows. Don't worry about ground water, or ocean water, filling the hole, etc., etc. Consider that these don't happen, maintaining the integrity of the hole you've drilled.Now hold an object above the hole and release it to fall down the hole. Will the object hit a wall? If so, which one, the East, West, North, South, or some other wall of the hole?
Solution. Since the force on the falling object is radial at all times there's no torque on it (about the center of the Earth). Therefore, the angular momentum of the falling object is conserved. Then rω = Rωe where ω is the angular velocity of the falling object, r is its distance from the center of the Earth, R is the radius of the Earth and ωe is the angular velocity of the Earth. Since r decreases with time, ω must increase with time, therefore ω > ωe all the way down. The falling object gets "ahead" of the walls of the hole. Since the Earth rotates west to east, the falling object must hit the east wall.
The same argument can be used for an object dropped from a high tower at the equator.
This conclusion is not affected by the radial distribution of mass within the Earth, just so long as it is a solid Earth (as given).
Some books give the problem in a different fashion. They specify that the Earth also be at rest. Kleppner and Kolenkow [An Introduction to Mechanics, McGraw-Hill, 1973] ask students to show that in this case the motion is simple harmonic with a period of 84 minutes, and note that this is the same as the period of an Earth satellite in low orbit with r approximately equal to R. That solution is straightforward.
K&K specifically exclude consideration of hitting the wall, by saying: "In deriving this result, you need to treat the Earth as a uniformly dense sphere, and you must neglect all friction and any effects due to the Earth's rotation." I wonder why they didn't just say, "Assume the Earth doesn't rotate."
They fail to address some obvious questions.
- Why should this be the same period as the satellite in low Earth orbit? Is there some insightful-conceptual way we might have expected this?
- What if the hole were drilled through the center starting at some other latitude?
- What if the hole were drilled perpendicular to the Earth's axis and through the Earth's center, starting at any latitude?
- What if the hole were drilled in some other way? If it were drilled pole to pole, the rotation of the Earth wouldn't affect the fall.
Martin Gardner discussed this in one of his Scientific American "Mathematical Games" columns.
The period in the frictionless hole:
Restating the question: The period for the circular "skimming" orbit (of radius equal to the Earth's radius, R) is
- T = 2π(R/g)1/2
Show that this is the same as the period of motion in frictionless straight hole through the center of the stationary Earth.
Partial Answer:
First let's address the question of the force on an object within a homogenous spherical distribution of mass.
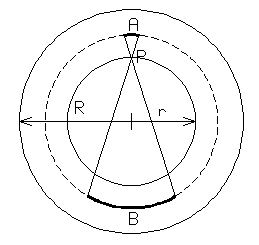 The force on a piece of
mass at point P a distance r from the center is due to all
mass in the sphere of radius less than r. All mass at
distances greater than r contributes nothing. This can be
seen by subdividing the mass in the outer shell into many
shells of infinitesimal thickness. Then take
infinitesimal solid angles with vertices at the point P.
The two pieces of mass within the solid shell contribute
oppositely directed forces at P, of size proportional to
the inverse square of their distances from P. But by
geometry, their volume is proportional to the square of
their distance from P. Therefore, their forces are equal,
and being oppositely directed, add to zero.
The force on a piece of
mass at point P a distance r from the center is due to all
mass in the sphere of radius less than r. All mass at
distances greater than r contributes nothing. This can be
seen by subdividing the mass in the outer shell into many
shells of infinitesimal thickness. Then take
infinitesimal solid angles with vertices at the point P.
The two pieces of mass within the solid shell contribute
oppositely directed forces at P, of size proportional to
the inverse square of their distances from P. But by
geometry, their volume is proportional to the square of
their distance from P. Therefore, their forces are equal,
and being oppositely directed, add to zero.
The mass within r is proportional to r3. Its gravitational force acts as if it were concentrated at the center of the sphere, and therefore the force it exerts at P is proportional to r-2. Therefore the gravitational force at distance r is proportional to r3r-2 = r.
Now consider the straight hole or tunnel through the Earth, but not through the center of the Earth.
The hole has frictionless walls, or tracks. The gravitational force within the Earth is f = -kd where d is the distance from the center. When d = R the force is mg. At the center of the Earth the force is zero.
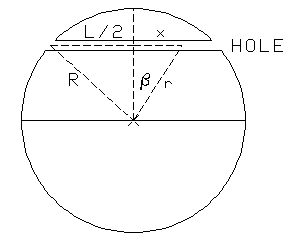 At any angle β, the component of gravitational
force along the tunnel is f = F(r)sin(β).
At distance x
from the center of the hole an object is distance r from
the center of the Earth. x = r[sin(β)], and we already
know that F(r) = -kr and k = mg/R so
At any angle β, the component of gravitational
force along the tunnel is f = F(r)sin(β).
At distance x
from the center of the hole an object is distance r from
the center of the Earth. x = r[sin(β)], and we already
know that F(r) = -kr and k = mg/R so
- f = -kr sin(β) = -kx
Therefore the force is proportional to the distance, meeting the requirements for simple harmonic motion with an effective "spring constant" k.
Orbits and the Simple Pendulum?
Some books note that these periods we've just found are the same as the period of a simple pendulum of radius equal to the radius of the Earth. The formula for a simple pendulum is
- T = 2π(L/g)1/2
where L is the suspension length. This formula is, of course, limited to small angles of swing.
Is there some profound significance in this fact? For a moment we are deluded into thinking that there's something profound lurking under this equality, something our insight should fathom. But we deceive ourselves.
First, imagine the situation and draw a diagram. The pendulum support is one Earth radius above the Earth's surface, or 2R from the Earth's center. Now you don't have to have a skyhook to hang such a pendulum. You can use a long air track and curve it upward till is has the radius of the Earth. The reaction force normal to the track plays the role of the tension in the string of a pendulum. It's a gedanken experiment, of course, but a lot less expensive gedanken experiment than using a skyhook. Remember that the amplitude of the pendulum motion should be very small compared to the radius of the track, which is the radius of the Earth.
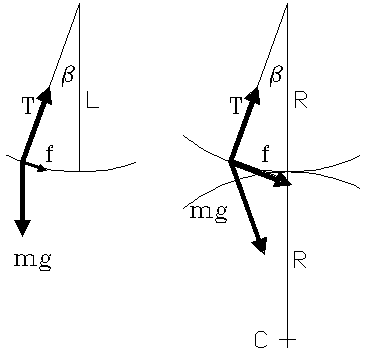
The diagram on the left shows an ordinary pendulum in which the suspension length, L, is much less than the radius of the earth, R. The right diagram shows the situation with a pendulum suspension length equal to the radius of the earth. In both diagrams the amplitude of swing is exaggerated.
The pendulum formula is based on the assumption that the gravitational acceleration g is constant in magnitude and direction, and that L is much less than R, where R is the radius of the Earth. It is not correct when L = R, not even when the pendulum angle is small. One must take into account the fact that the reaction force normal to the track and the gravitational force both make equal size angles with the vertical. So the "restoring force" (along the arc of the motion) is twice as great (in the limit as β goes to zero) as if you assume the gravitational force has constant direction. In fact, if this is done correctly, then when L = R the period is
- Tp = 2π(R/2g)1/2
or just 0.707 times the period of the skimming orbit.
Equalities between two cases don't always have profound significance, especially when one of the cases is a result of error, misconception, or misapplication of a formula.
Skater's Spiral
![[Rope secured and wrapped around a pole of radius R, free end extending
straight.]](skater.gif) A rope is tied securely
and wrapped around a cylindrical pillar in the center of a
skating rink, with a portion of the rope extending straight
out from the surface of the cylinder. A skater on
frictionless ice grabs onto the extended length of the
rope. The skater's velocity is perpendicular to the
straight portion of the rope when she grabs it. She retains
her grip on the rope as she spirals around the pillar with
continually decreasing radius. Assume that the rope segment
she holds remains perfectly straight at all times. What is
the skater's speed when she crashes into the pillar?
A rope is tied securely
and wrapped around a cylindrical pillar in the center of a
skating rink, with a portion of the rope extending straight
out from the surface of the cylinder. A skater on
frictionless ice grabs onto the extended length of the
rope. The skater's velocity is perpendicular to the
straight portion of the rope when she grabs it. She retains
her grip on the rope as she spirals around the pillar with
continually decreasing radius. Assume that the rope segment
she holds remains perfectly straight at all times. What is
the skater's speed when she crashes into the pillar? Answer. The skater crashes into the pillar with the same speed she had when she grabbed the rope. And her velocity at the time she contacts the pillar is perpendicular to the pillar's surface. The rope does no work on the skater. How can we know this without complicated analysis?
The rope has negligible mass compared to the skater, so we treat it as massless. The force the pillar exerts on the rope does no work, for the portion of rope wrapped around the pillar doesn't have any component of motion along the rope's length. Therefore the other end of the rope does no work on the skater. Since no work is done on the skater, the skater's kinetic energy, and speed, remain constant.
![[Tracing of the skater's path.]](skater2.gif) It follows that at all times the rope is perpendicular to
the skater's velocity. That's true, though not obvious, and
not particularly easy to show directly. It's probably a
calculus problem. But you can get a lot of insight by
taking a round jar lid, fastening a string to it, and
placing it on a sheet of paper. Put a loop in the free end,
and with a pencil in that loop hold the string taut and
draw the path on the paper as you swing around the round
jar lid. The path will end up with the curve closing in on
the lid, and the path will be perpendicular to the lid's
rim where it hits. This picture was made in this way.
It follows that at all times the rope is perpendicular to
the skater's velocity. That's true, though not obvious, and
not particularly easy to show directly. It's probably a
calculus problem. But you can get a lot of insight by
taking a round jar lid, fastening a string to it, and
placing it on a sheet of paper. Put a loop in the free end,
and with a pencil in that loop hold the string taut and
draw the path on the paper as you swing around the round
jar lid. The path will end up with the curve closing in on
the lid, and the path will be perpendicular to the lid's
rim where it hits. This picture was made in this way.
Consequences to ponder. The skater's speed remains constant. What about her angular velocity and angular momentum?
Answer: Taking the fixed point at the center of the pillar as a center of torques, we see that the rope's tension causes a torque on the pillar, giving the pillar and the Earth an angular momentum in the same sense as the skater's angular velocity. Therefore the skater's angular velocity and angular momentum decrease. (Her angular momentum reaches zero when she impacts the pillar.)
Another way to look at this: The rope exerts a torque on the skater. The lever arm of this force about the pillar's center is just the radius of the pillar, R. At any instant the torque, R × F is a vector opposite in direction to the skater's angular momentum, so this must decrease her angular momentum. [See Sutton, M-186.]
At the moment of her impact with the pillar, her angular momentum has become zero and she impacts with velocity that is directly toward the center of the pillar.
One reason people sometimes get off on the wrong track when analyzing this problem is their previous familiarity with problems of central force motion, where the force is always directed toward a fixed point. The force of tension on the skater with rope around the pillar is not directed toward a fixed point. For an example of central force motion, consider a skater on a frictionless rink, holding a rope that is fastened at a fixed point. Now, as time goes on, the rope length remains constant, and the skater's motion is constant speed around a circle. Now suppose, due to some clever mechanism, the rope is continually shortened, perhaps by having it pass over a pulley at the center of the rink, and then to a motor winch. But we take care that the rope at the center always passes through a fixed point there. [One of these days maybe I'll do a diagram.] By insisting that the rope pass through a fixed point, we ensure that there's no torque on it about that point. Now the angular momentum must be constant, as it always is in central force motion. So as the radius decreases, the skater's speed must increase as she spirals toward the center. This tells us that work is being done on the skater. The skater's velocity is no longer perpendicular to the rope, but has a small radial component. This means there's a radial displacement toward the center, and the radial force is responsible for that displacement. That's where the work is done on the skater—work done by the motor that drives the winch that's shortening the rope.
One might say that the central force circular motion case is the limit of the pillar case as the pillar radius goes to zero.
Follow-up question. Would you get the same result if the pillar wasn't round in cross section?
Answer: Yes. The analysis above in no way depended on the pillar being circular in cross-section. The next problem "Rope and Capstan" gives some additional insight into that.
Follow-up to the follow-up question. What if the pillar were square in cross section with slightly rounded corners. How does the time it takes to crash compare with the case of the round pillar? That time isn't hard to calculate in the square pillar case, since the speed is constant.
Rope and Capstan It is a well-known result in engineering statics that the tensions TA and TB at the ends of a rope or belt wrapped round a capstan are related by
- TB = TA e μ β
where μ is the coefficient of static friction between rope and capstan, and β is the angle of wrap.
This is generally derived for a cylindrical capstan. Prove that it also applies to other smooth cross-sectional shapes. Solution left for the reader.
The case of the skater problem with friction is much more involved. An excellent discussion of this problem can be found in:
Arons, Arnold. "An F=ma Analysis of the Spinning Skater and Decaying Satellite Orbit." The Physics Teacher, 37, 3 (March 1999), p. 154-160.
Mixtures
Liquid Transfer
A beaker contains 1 liter of pure water. Call it the "water" beaker. A second beaker contains one liter of pure alcohol. Call it the "alcohol" beaker. We have chosen two liquids that may be mixed safely. 100 ml of alcohol is taken from the alcohol beaker and put into the water beaker, then thoroughly mixed. The same amount, 100 ml, of this mixture is removed from the water beaker and put into the second alcohol beaker. Now both beakers contain 1 liter of liquid. (We ignore the fact that a mixture of water and alcohol occupies a volume slightly less than the sum of the volumes of the two materials before they were mixed.) Repeat this process 100 times. (This is a thought experiment, so this won't take all that long.)Now, both beakers contain a mixture of water and alcohol, and both beakers contain 1 liter of liquid. Is there now more alcohol in the water than water in the alcohol?
Answer:
This is a classic example of a problem in which more information is given than is needed. It's also an example of insightful approaches versus detailed mathematical approaches. Many people will get out their calculators and start calculating mixture ratios with the numbers given.
It doesn't matter how much is initially in the beakers; they need not have equal volumes. It doesn't matter how much liquid is transferred each time. It isn't necessary to mix the liquid after a transfer. It doesn't even matter how many transfers are made, so long as each beaker ends up with the same volume it had initially.
And the result is... The water beaker will end up with as much alcohol as the alcohol beaker has water. The reasoning is simple. The water beaker ends up with some alcohol in it. That alcohol must have come from the alcohol beaker. Since the alcohol beaker now has as much liquid as it had initially, the amount of alcohol it lost has been replaced by that same amount of water. Q.E.D.
The same principle may be applied to volume, mass, weight, or number. It may be applied to containers of marbles, beans, decks of cards, poker chips, coins, etc. I prefer this problem expressed in numbers of things rather than volumes. In the alcohol and water case, volumes are not preserved when the two materials are mixed, due to molecular interactions. This has been ignored in the answer given above. With discrete objects that problem doesn't arise. Also, it is not easy to verify the result if the problem is about volumes.
Martin Gardner discusses this in his chapter "Mathematical Card Tricks" in Hexaflexagons and Other Mathematical Diversions, a reprint of The First Scientific American Book of Mathematical Puzzles and Games, U. of Chicago Press, 1959, 1988.
With that in mind, here's a variation designed to baffle even physics students. Obtain some dry beans and some dry rice. Find a coarse screen or strainer that the rice can pass through but the beans can't. You'll also need three glass beakers or similar containers of equal weight and a beam balance for comparing their weights. Put a beaker on each balance pan, then add beans to one and rice to the other till they weigh the same. Now pour some beans from the bean container to the rice container. Shake or stir the mixture, and pour some back into the bean continer. Repeat this a few times, sometimes transfering a little (a spoonful), sometimes a lot (pour it from one container to the other).
Now ask someone in the audience to choose beans or rice. (This is a diversion that doesn't matter one bit.) Suppose they choose rice. Then ask them to choose one of the containers on the scales. (It doesn't matter which they choose, or we wouldn't ask.) Now transfer material from the heavier to the lighter container, pretending to very carefully select beans or rice, but actually it doesn't matter. (That's showmanship.) Do this until the two containers weigh the same. Announce that you have now made the rice (chosen by the audience) in the chosen container weigh exactly as much as the beans in the other container. You could even string the audience along by claiming that you could always judge the number of marbles in a container at carnivals, and win the prize. (That's irrelevant anyway, for we are using weight here, not numbers of things.)
Pour the contents of the chosen container through the sieve letting the rice fall into the empty beaker, and replace that beaker of beans on the scale. Now take the other container, with mixed beans and rice, and strain out the rice, replacing the remaining beans and container on the scale. The weights balance. It's a miracle! Finally, assign the students the task of explaining why it works every time.
The value of the rice-beans version is that the two can be easily and quicly separated. Another variant is to use brass and steeel hardware nuts. These are easily separated with a magnet.
Hydrostatics
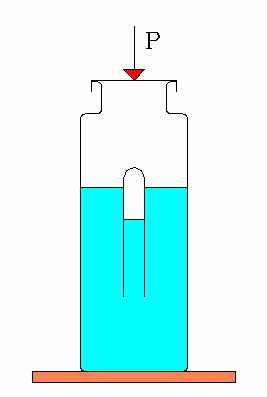 |
Floating Test Tube
The Cartesian diver consists of a small figurine of glass or ceramic just floating in water inside a tube, bottle, or other container. When the lid of the container is pressed, the diver sinks to the bottom. You can make your own, as we describe here.An inverted glass test tube floats in water if there's enough air trapped inside. Obtain a jar or bottle of water, and float an inverted test tube in the water with just enough water rising in the tube so that the tube floats as shown. Close the top of the jar with a flexible rubber sheet so it makes an airtight seal.
What will happen if we press the rubber sheet inward so that the volume inside the jar is reduced?
- The test tube will rise up a bit with respect to the water level inside.
- The test tube will sink a bit with respect to the water level inside.
- The test tube will neither rise nor sink with respect to the water level inside.
Answer: Some books invoke Archimedes' principle, and say that the weight of the test tube including the water in it increases because water is forced up into it, making the average density of the tube (including its air and water) greater. This is true, but it seems a rather unsatisfying explanation in this case, since you seem to be arbitrarily defining the tube to have a "boundary" at its open end. You could take the boundary to include the glass and the air only, for a more plausible "explanation" based on the increase of density of the air trapped in it.
It helps to look closely at details and consider Pascal's law. If we adjust the amount of air in our inverted test tube so that the tube just barely floats, then it behaves in the same manner as the ancient toy called the "Cartesian diver". Slight added pressure on the walls of the outer container causes the test tube to sink all the way to the bottom. Discussions of this device usually don't explicitly consider the air in the top of the container. Does that air make a difference?
Pressing on the lid of the container reduces the total volume inside. Water doesn't compress much; it's the air inside the container that compresses to smaller volume. The air trapped in the test tube compresses, and, by the gas laws, its pressure increases. But the pressure of the air in the top of the container increases also. Pascal's principle tells us that the change of pressure is the same at all points in the liquid. Pascal's principle also applies to the air in the top of the container, and to the air trapped in the test-tube. We must now determine whether these pressure changes result in a net downward, or upward, force on the glass test-tube.
Suppose the pressure in the top of the container increases by amount P. It exerts a downward force on the glass. The pressure of the air trapped in the tube increases by the same amount. It exerts an upward force on the glass. The force due to gravity on the glass test tube is the same as before. Nothing else can exert a vertical component on the glass test tube. Therefore the net force on the test tube is still zero.
From this we conclude that the test tube is still in equilibrium after the pressure change. But do not conclude that it didn't move up or down during the pressure change! Remember that pressure doesn't change instantaneously throughout the container.
The pressure of the air trapped inside the tube has increased. This causes that air to occupy a smaller volume (from the Gas law). But the pressure difference between the water level outside and inside has increased by the same amount. That pressure change is due to the pressure change increasing everywhere in the water by the same amount (Pascal's principle). The height difference between the water level outside and inside the test tube is unchanged!
To see this crucial point, it's helpful to write an equation. The net force on the glass tube is the vector sum of the downward force due to pressure of air above, the upward force due to pressure of air below, and the weight of the glass. We can write an equation for the pressure at the water surface within the test tube.
- P a + ρgh1 = Pb + W/A
where Pa is the initial pressure in the top of the container, Pb is the initial pressure of the air trapped in the test tube, ρ is the density of water, g is the acceleration due to gravity, h1 is the initial height difference of the water levels, W is the weight of the test tube, and A is the cross-sectional area of the inside of the test tube.
After the pressure change the two pressure terms have been increased by the same amount. W/A doesn't change. Therefore the dgh term must remain the same, and we conclude that the height between the water levels does not change.
Therefore, the test tube must have moved downward during the pressure change. Does anyone care to analyze the time-sequence of pressure and force changes during that brief time interval?
We conclude that the presence of air above the liquid in a Cartesian diver will not compromise its performance.
Variation. In my youth this was often done with a medicine bottle of the old fashioned kind that had flat front and back surfaces. The filled bottle could have its volume compressed by squeezing the front and back of the bottle, enough to cause the cartesian diver action if the diver was at the critical effective density very near that of water. That made the effect seem magical, for you could hide the fact that you are squeezing the bottle, and observers seldom caught on that glass is sufficiently deformable that you can even do this. Nowadays you can sometimes find plastic bottles that are shaped this way, but if you are using a plastic bottle, the shape hardly matters anyway.
Hydrostatic Puzzles
1. Consider an essentially cylindrical container with a flexible waist section (of rubber or such) half way up. A sturdy belt is wrapped loosely round the waist. The whole thing is on a sensitive balance. Now tighten the belt, narrowing the waist. The water level rises inside the upper part of the container.
- (a) Does the weight registered on the balance change?
- (b) Does the pressure on the bottom of the container change?
2. A glass beaker of water is on the balance. A metal ball is hung from the edge of the beaker but outside the beaker. The weight of everything is recorded. The ball is now put inside the beaker hanging from the string, not touching the bottom.
- (a) Does the weight registered on the balance change?
- (b) Does the pressure on the bottom of the container change?
3. Repeat experiment 2 with a cork, first outside the beaker on the balance pan, then floating in the water. 4. Do a free-body analysis of the ball and the cylinder in problems 2 and 3, accounting for everything.
Answers:
Cylinder: Force P downward on bottom due to liquid pressure at bottom. Force N upward, due to the balance pan. Force T downward, due to tension in string holding the ball.
Ball: In the first case: Force T upward, due to string tension. Weight W downward. In second case one also has an upward buoyant force B on the ball equal to the weight of the displaced liquid.
T decreases by amount B to maintain the ball in equilibrium. Therefore, since N is constant, P must increase by that amount.
Sinking Boat
Make a miniature model of a boat from metal foil and float it in a beaker of water. Topple the boat to sink it. Does the pressure of the water on the bottom of the beaker increase, decrease, or remain the same?Answer:
The floating boat displaces its own weight of water (Archimedes' principle). When it is sunk it displaces its own volume of water (displacement principle). Its own weight of water is larger than its own volume of water, since its density is greater than that of water (after all, it did sink). Therefore, after sinking, the boat displaces less water, so the water level in the beaker drops. Therefore the pressure of the water on the bottom drops. The force of the boat sitting on the bottom compensates exactly for reduction in force due to pressure, so the total force on the bottom of the beaker doesn't change. After all, if the beaker were sitting on a pan of a balance, we wouldn't expect the balance reading to change.
Alternatively one could argue: The weight of the beaker and contents doesn't change. There's no vertical component of force due to the liquid pressure on the side walls of the beaker, only on the bottom. When the boat floats, the force on the bottom of the beaker is entirely due to liquid pressure. After the boat sinks, the force on the bottom is partly due to the weight of the boat sitting on the bottom. The net force on the bottom must be the same in both cases, since the total weight of the system didn't change. Therefore the pressure of the liquid must have decreased.
Physics on the Lake 1
 |
You are in a boat on a perfectly calm lake. There's an anchor in the boat. You drop the anchor overboard and it sinks to the bottom of the lake. During this process, does the level of the lake rise, fall, or stay the same?
Answer:
We assume that there's a water level gauge of great precision somewhere, perhaps at the boat dock, to measure the lake level. This puzzle, and the next two puzzles, use Archimedes' principle: "The Buoyant force on an object submerged or floating is equal to the weight of the displaced liquid." They also use Newton's law: "The net force on a body in equilibrium is zero."
The anchor in the boat adds to the boat's weight, and the presence of the anchor there causes a displacement of an amount of water that weighs as much as the anchor. (The upward buoyant force due to the displaced water has the same size as the weight of the anchor, by Newton's law.) I.e., the anchor in the boat displaces a weight of water equal to its own weight. When the anchor is at the bottom of the lake it displaces an amount of water equal to its own volume. Its weight is greater than the weight of an equivalent volume of water (that's why it sank), so it displaces more water when in the boat than when at the bottom. The water level of the lake drops when the anchor is thrown overboard.
Physics on the Lake 2
You are in a boat on a perfectly calm lake. A floating log passes near. You grab the log and put it in the boat. During this process, does the level of the lake rise, fall, or stay the same?Answer:
The floating log displaces its own weight of water. The log in the boat displaces its own weight of water. The level of the lake is unchanged.
Physics on the Lake 3
You are in a boat on a perfectly calm lake. A wooden log is in the boat, and the anchor is at the bottom of the lake. This is the same log as in the previous problem, and it would float on the water if simply tossed overboard. But instead, you raise the anchor, tie the log to the anchor, and drop both overboard, the anchor pulling the log to the bottom. Consider the entire process, from (A) when the log was in the boat and the anchor was on the bottom, to (B) when the log and anchor were both on the bottom. During this process, A to B, does the level of the lake rise, fall, or stay the same?Answer:
The log in the boat displaces its own weight of water. The log at the bottom displaces its own volume of water. Its weight of water is less than its volume of water (that's why it normally floats), so the level of the lake rises. The anchor was at the bottom before and also after, so it causes no change in the lake level.
Milk Bottle

An old-fashioned bottle of nonhomogenized milk is left undisturbed. The cream rises to occupy the narrow neck at the top. Is the pressure of the milk on the bottom of the bottle now the same as before, greater, or less?
Note: Many materials, when mixed, occupy a volume different than their total volume when separate. This is generally a small effect. In this problem, this volume difference will be ignored. It would, in fact, have negligible contribution to the changes of pressure being considered here.
Answer:
The total force that the bottom of the bottle exerts on the table stays the same. After all, the total weight of bottle and contents hasn't changed. However, the pressure (force/area) of the milk on the bottom of the bottle decreases. Why?
First, we assume that a clever person made up this question, probably a physicist. So the wording of the problem should be examined with care. Why a milk bottle and not a beaker? Aha!, the shape of the bottle must play a role here. This suspicion is reinforced by the reference to the cream being only in the neck of the bottle.
Some students think that the problem has only to do with the liquid, forgetting that the liquid is in a container. They also imagine the bottle sitting on a scale, and since no mass has changed, the scale should read the same. That's correct. But then they incorrectly identify the scale reading with the pressure.
Some of you may not have ready access to Arnold B. Arons' A Guide to Introductory Physics Teaching, so I excerpt out the relevant parts of his discussion of this problem, from Section 11.3 HYDROSTATIC PRESSURE.
Understanding the concept of "hydrostatic pressure" involves a good bit more than acquiring the definition "force per unit area." A major step toward understanding resides in appreciation of the full significance of Pascal's Law: that the pressure at any point in a fluid is the same in all directions. The usual formal "proof" of this idea is given by examining the static equilibrium of a small volume of fluid, for example, one having a parallelepiped shape and triangular cross section. This treatment is so abstract that, even if it is presented, very few students assimilate its physical implications. Many introductory courses now eschew such treatments entirely in order to save time for other subjects. This is a legitimate choice, but teachers must then remember that something very subtle and fundamental has been left out and must be prepared to help close the gap at some subsequent point.Arons' "central column" is a cylinder of the same diameter as the narrow neck of the bottle. The reason that the average density in the central column is less after the cream separation is because the lighter cream, which was dispersed throughout the entire volume, is now entirely in the central column.The subtlety of the insight, and the fact that many individuals (including many active physicists) have not really acquired it, are indicated by the responses given to the following question: A container of the shape in Fig. 11.3.1 initially contains a uniformly dispersed mixture (or colloidal suspension) of two immiscible liquids of different densities (e.g., oil in water; cream in milk). As time goes by, the lower density fluid separates and collects in the throat of the container. How does the final pressure on the bottom of the container (after the separation is complete) compare with the initial pressure (when the fluids were uniformly dispersed)? Is it the same, greater, or smaller than the initial pressure?
Novices tend to say they do not know, but the majority of those who have had some physics but have never thought about such manifestations of fluid pressure tend to say that the pressure must remain the same. Those who suggest a line of reasoning rather than just making an intuitive guess say that, since the weight of the fluid is unchanged, the total force on the bottom, and therefore the pressure on the bottom, must remain unchanged.
This reasoning is incorrect because the pressure on the bottom of a container of any shape other than purely cylindrical is not equal to the weight of fluid divided by the area of the bottom. Since the fluid pressure is the same in all directions at any point, the fluid at a wall exerts a force on the wall, and the wall, in turn, exerts a force on the fluid. The sloping walls of the container exert a downward component of force that influences the pressure on the bottom of the container. Since, on separation, the average density of the fluid in the central column is less than it was initially, the pressure at the bottom of the central column has decreased. The pressure at the level of any point along the sloping walls has also decreased; the downward force exerted by the wall has decreased; the pressure has decreased all over the bottom.
This is a problem in which the answer is non-intuitive for most people. It is also a problem where ill-formed or wrong concepts can lead to a persuasive argument that is simply wrong.
Arons explicitly uses just part of Pascal's principle: the fact that pressure at a point acts equally in all directions. The other part, properly stated, deals with changes in pressure:
When the pressure is changed (increased or decreased) at one point in a homogenous, incompressible fluid, all other points experience the same pressure change.Some students may still be bothered. The pressure of the fluid on the bottle's bottom decreases, and therefore the total force of the fluid on the bottle's bottom decreases. Yet the force the bottom of the bottle exerts on the balance pan remains the same. What accounts for the difference?
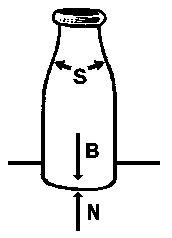 To answer this one
must consider the free body diagram of the glass bottle.
We are particularly interested in the vertical force
components. There's the bottle's own weight, which doesn't
change. There's the force of the fluid on the bottle's
sloping shoulder. This has a vertical component. The
horizontal components of pressure on the neck don't concern
us, and also, they add to zero before and also after the
cream separates. This is because they are uniform in size and
radial all around the bottle neck. The vertical components of pressure on the
neck add to an upward force that does change; it
decreases as the cream separates. This exactly equals the
size of the decrease of downward force of the fluid on the
bottom of the bottle, since the glass bottle remains in
equilibrium.
To answer this one
must consider the free body diagram of the glass bottle.
We are particularly interested in the vertical force
components. There's the bottle's own weight, which doesn't
change. There's the force of the fluid on the bottle's
sloping shoulder. This has a vertical component. The
horizontal components of pressure on the neck don't concern
us, and also, they add to zero before and also after the
cream separates. This is because they are uniform in size and
radial all around the bottle neck. The vertical components of pressure on the
neck add to an upward force that does change; it
decreases as the cream separates. This exactly equals the
size of the decrease of downward force of the fluid on the
bottom of the bottle, since the glass bottle remains in
equilibrium.
Why does the upward force component on the bottle neck decrease as the cream separates? Before separation the pressure at the neck was due to the milk-cream mixture above it. After separation, the liquid above had a greater ratio of cream to milk, and therefore a smaller average density.
Balance Puzzle.
Two identical containers have the same volume of water, and one is placed on each pan of a double-pan balance. Obtain a hollow ball and a solid ball that have the same volume. The hollow ball floats in water, the solid ball sinks. A string from the ceiling suspends the solid ball so it is completely submerged in the water on the left pan. The hollow ball is held submerged in the water of the right pan by a string fastened to the bottom of the beaker. Now what will be the condition of the double pan balance?- The balance indicator will read zero. The scale is still balanced.
- The left pan (with the solid ball) moves down.
- The left pan rises.
This diabolical problem has all sorts of distractions, tempting you to do it "the hard way". Let's look for the easy way.
Consider the initial balanced system, isolating the systems of the beakers and their contents. Let upward forces be positive, downward ones negative.
Adding the hollow ball and the tether string to the right beaker adds the mass of that ball, m, to that system. The tension of the tether string holding it submerged introduces two internal forces, and being equal and opposite, have no effect on the net external force on this system. The buoyant force of water on the ball is equal and opposite to the force of the ball on the water, and as internal forces, these have no effect on the net external force on this system. So the only change in net force on the right side of the scale is the weight of the ball, mg, a downward force.
Adding the suspended solid ball to the left beaker does not add the mass of that ball, M, to the system. But it does introduce an external force of the ball acting on the water. The buoyant force, B, of water on the ball is equal and opposite to the force of the ball on the water. So the change in the net force on the left side of the scale is of size B, and is a downward force.
This is consistent with our analysis of the heavy finger problem. The finger thrust into the water experiences an upward buoyant force equal to B. So the finger exerts and equal and opposite force on the water in the beaker. This is the downward force that unbalances the scale in that experiment. In this puzzle, B is larger in size than the hollow ball's weight, since the hollow ball would float if allowed to. So the left pan registers more weight.
This raises an interesting issue. Why did we say that the ball become part of the system on the right pan, but the ball on the left pan does not? The ball on the right is attached to the beaker with a string, and must move up and down exactly in synchronization with the beaker and water. Ignoring viscous drag as negligible, the ball on the left does not move as the left pan rises or falls, hence no work is done on or by that ball as the scale pan moves.
Work is not done on or by a body unless it moves under the action of a force. Balance scales move up or down in response to changes of force on its two pans. We must have that motion to determine the position of equilibrium, where the motion is periodic about the equilibrium point that we have calibrated as "zero" on the balance indicator of the scales.
Quite a few variations may be concocted to perplex students. Actually some of these are easier.
- Two balls of equal volume but different density, both suspended from the ceiling and completely immersed in the water.
- Two identical hollow balls, one floating on the water surface, one tethered to the bottom and completely submerged.
- Two identical steel balls, one in the bottom of one can, the other suspended from the ceiling, completely immersed in the water in the other can.
Optics
![[Figure 1. Hand
in front of plane mirror, showing hand image correctly
oriented.]](mirror-f.gif)
Mirror Reversal?
Why does a plane (flat) mirror seem to reverse your image left/right but not up/down? This frequently asked question has value for encouraging students to think more precisely and use words carefully. Why should this question even be interesting? Where's the "problem"? The discussion shouldn't be carried out entirely in the abstract. Have a large mirror to demonstrate. Rotate the mirror around its normal as a student looks at the image. The image doesn't rotate. The mirror seems to be operating with axial symmetry. Then why should the mirror treat up/down differently than right/left? (Some students will even suggest gravity has something to do with it, so have such students look in the mirror and lean their heads sidewise.) Have one student look into the mirror while another stands behind the mirror looking at the first student. Have each touch the top of their heads. Have each touch their right ear. Perhaps have each wear something distinctive on the right ear.The figure below shows that a pair of plane mirrors at right angles behaves differently than does a single plane mirror. Have two mirrors hinged (for ease of storage) with a precise arrangement to hold them at perfect right angle alignment for the demo. Curved cylindrical mirrors can be made by forcing thin plastic mirrors into a slight curve. This may not seem like physics, but it is certainly a good exercise in geometric thinking.
![[Figure 2. Man
looking in mirrors fastened at one edge, making angle of 90
degrees, with edge axis vertical, and also horizontal. Your
image seen in a cylindrical concave mirror, at two
orientations.]](mirrors.gif) Answer:
Answer:
The plane mirror doesn't reverse up/down, all agree. It doesn't reverse left/right either. If you point a finger up, the image finger points up. If you point a finger to your right, the image points its finger to your right. When you keep all right/left references consistently in a fixed reference frame there's no problem. But there's more that's worth investigating here.
There's considerable literature on this question, some of it misses the mark. A good summary can be found in: Martin Gardner's The New Ambidextrous Universe, Freeman, 1990 ... 1979, pp. 3-6 and 19-22. Much of the confusion rests on three things:
- The ambiguity of the terms up/down and left/right.
- Other semantic difficulties.
- Shifting reference coordinates during the discussion.
- Failure to explain the matter and still address the fact that the plane mirror is symmetric about any of its normals.
What I've forgotten is that my clone had to rotate 180 degrees about a vertical axis after leaving my body and then walking around to face me. That is a reversal of the clone's left/right coordinate reference. (It also reverses forward/backward, with respect to a line from my nose perpendicular to the mirror, a fact whose importance will be clearer in explanation 2.)
Explanation 2. The plane mirror, having symmetry about any normal, can be rotated about that axis without any change in the image. For simplicity, imagine the axis from your nose and normal to the mirror. All image transformations must be symmetric with respect to that axis. The mirror does not invert up/down or right/left. It inverts along the mirror's axis. If you mentally walked forward through the mirror, till your right ear was at the position of the image's right ear, and your left ear at its left ear, your nose would be at the back of its head, and the back of its head would be at your nose. The top of your head would be at the top of the image's head, and both are still "up." Your reference for right/left and up/down hasn't changed, for you walked along the symmetry axis of the mirror. The mirror inverts the image along its symmetry axis, inverting the nose to the back of the head and vice versa. It is a well-known, and easily provable fact, that any point on the image is exactly as far from the mirror as the corresponding object point. Thus your image's nose is nearer to you than the back of its head.
Further experiments.
Hold a printed page facing the mirror. The writing on the image is 'backwards' just as it would be if the printing were on a transparent sheet and you were trying to read it from the back side.
For a new slant on this problem, hold a large plane mirror at arm's length and in a horizontal plane facing up and look at the reflection of a poster on the wall that has printed text. The image is upside down and the text reads backwards. Now swing your arm to the right and rotate the mirror until it is in a vertical plane, keeping the poster's image in your view. The image rotates through 180 degrees, and is now upside down. Swing your arm up and high, rotating the mirror another 90 degrees till it is facing down. The image has rotated another 180 degrees, and is now right side up. The image rotates through twice the angle that the mirror rotates. Rotate your arm another 90 degrees until... Whoops. You'd better seek medical help for that arm.
The 'simple' magnifier
 |
| "It's really quite simple, Watson" |
|---|
The formula for the angular magnification of a simple magnifier is found to be M = d/f + 1 for the case where the final virtual image is at a distance d from the lens. Usually d is taken as the near point of the eye, 25 cm. Suppose we want the case where the image is placed at infinity. Why is it that when we plug d = ∞ into this equation we don't get the right answer? We seem to get M = ∞, which obviously isn't correct. What is the right answer? How do you get it? [1]
Answer:
This is a nice essay question to foil the plug-and-chug crowd—those students who do physics problems by blindly plugging data into a formula and grinding out an answer without involving the higher functions of the brain.
The wisest thing to do is to derive the magnification from first principles for the two cases, rather than to try to get one case from the other. They are fundamentally different cases.
Also, remember that the magnifier formulas are derived under the assumption that the magnifier is held near the eye. Students often forget this and assume that the formulas also apply when the magnifier is held near the page, much nearer than to the eye. They don't. The magnification is greatest when the lens is near the eye, and the object brought close. Also the field is larger and flatter when the magnifier is held near the eye. However, when Sherlock must examine something that can't be brought close, obviously the lens isn't very near the eye.
Angular magnification is M = tan(β)/tan(β0) where β is the angular size of the image seen with the optical instrument and β0 is the angular size of the object seen without the optical instrument.
In the case where the image is at the near point, d is the distance to the image, when the image is at the near point. The formula M = d/f + 1 is not valid for an image placed anywhere else.
In the case where the image is at infinity, d has only one meaning, the distance at which the object can be seen most clearly with the unaided eye. The formula M = d/f is not valid for an image placed anywhere else.
So, the meaning of d is different in the two cases. But one doesn't appreciate that fully until one derives both cases from first principles, with appropriate diagrams. Many textbooks do this derivation carelessly or incorrectly, though always arriving at the "right" formula.
What's the best image?
Hold a magnifier near your eye, and look at your hand. Move your hand closer or farther to see the details of your fingerprint "best". Try to understand what your hands, eye and brain have done to find the "best" image of your finger, and why.Look through a telescope at a distant object. Focus the telescope by moving the eyelens in its tube until the image is "best". Now (and this isn't easy) determine whether the virtual image is at infinity, at the near point, or somewhere in between. Explain this in comparison with the simple magnifier case.
Look through a microscope at a specimen. Focus the microscope until the image is "best." Where is the image? Why did you put the image there to get the "best" image?
Is the operational meaning of "best" the same in all of these cases?
Answers:
When using the simple magnifier close to the eye, an image may be seen clearly if it is located between the near point and far point of the eye. Consider looking through the lens at an object held in your hand. Most people bring the hand closer (and the image comes closer as well) until the image is at the near point of the eye. Bringing it even closer makes it "out of focus". There's a significant change in the image size, the image being larger as it is brought closer. Your brain easily senses this, and, desiring to see more detail, guides the hand holding the object to a position that makes the image as large as possible. This is not the most relaxed condition for the eye, but the brain weights clarity over comfort in this case.
When using a telescope, the image can be seen if it is anywhere between the near point and far point of the eye. But there's very little change in size of the image over this range—hardly enough to notice.So your brain guides your focusing hand to put the image where the eye will be most relaxed (the muscles controlling the lens will be under the least tension). This position is at the far point of the eye.
The microscope eyelens functions as a simple magnifier, so it's no surprise that the greatest clarity of image occurs when it is at the near point of the eye. However, for long term use, the user will often place the image at the far point for most relaxed and comfortable viewing.
Microscope magnification
The usual formula for the magnification of a standard microscope is given in some textbooks as:
- M = -(d/fo)(25/fe)
In this formula d is the distance from the objective lens, of focal length fo, to the real image near the eyelens. fe is the focal length of the eyelens. The first factor is the linear magnification of the objective, the second is the angular magnification of the eyelens, used as a simple magnifier with virtual image at infinity. The result, M, is angular magnification, a point that should be stressed, because one is looking at a virtual image. The 25/fe looks like the formula for the angular magnification of a simple magnifier used with the image at infinity. But the formula for the angular magnification of a simple magnifier of focal length f is given in most books is M = d/f + 1, where d is the distance from object to lens, usually taken as 25 cm or 10 inches. Yet those same books show a diagram of the microscope with the virtual image somewhere in the vicinity of the objective lens, that is, a finite distance not much different from 25 cm. Isn't there a contradiction here?
But we are passing up what folks in the ed-biz call a "teachable moment" if we don't raise a question here. When using a simple magnifier (or a microscope) one can use the instrument in such a way as to place the image anywhere from 25 cm (the near point of the eye) to infinity (the far point). Then why do we usually assume 25 cm?
When using a telescope, we can likewise place the image anywhere from 25 cm to infinity, depending on how we "focus" the instrument. Then why do we assume the image is at infinity when calculating the telescope's magnifying power?
The fact that textbooks give the angular magnification of a telescope as fo/fe tells us that the authors have assumed that the telescope is adjusted so that the focal point of the eyelens and objective lens are coincident, and that an object at infinity has its image at infinity. Yet some texts that make that point have a diagram showing a telescope with an image at a finite distance somewhere near the objective lens. Seems contradictory, doesn't it? See What's The "Best" Image above.
Endnotes:
[1] The use of the symbol ∞ here is colloquial. We must be continually careful to remember that infinity is not a number and should not be used in an algebraic equation. To write, or say, that q = ∞ is only saying that the quantity q "gets very large".
Additional insight problems with longer expositions.
Atwood's machine variants.Why are some pulleys crowned?
Other pages of insight problems.
More puzzles.Challenging Physics Problems. Puzzles requiring insight.
Physics puzzles. Nearly 100 physics puzzles.
![]() Input and suggestions are welcome at the address shown to the right. When
commenting on a specific document, please reference it by
name or content.
Input and suggestions are welcome at the address shown to the right. When
commenting on a specific document, please reference it by
name or content.
Return to Abuses of Physics.
Return to Donald Simanek's front page.
Revised Sept 2017.
